The de-Broglie wavelength L associated with an elementary particle of linear momentum p is bets represented by the graph
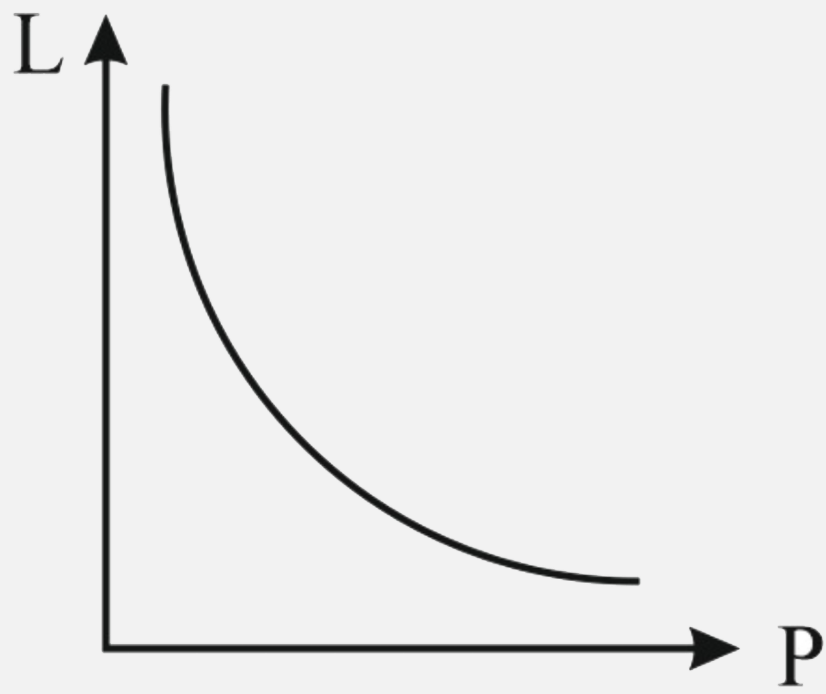
The correct Answer is:D
To solve the question regarding the de-Broglie wavelength L associated with an elementary particle of linear momentum p, we can follow these steps:
Step 1: Understand the de-Broglie wavelength formula
The de-Broglie wavelength λ is given by the formula:
λ=hp
where h is Planck's constant and p is the linear momentum of the particle.
Step 2: Relate the wavelength to momentum
From the formula, we can express the relationship between the wavelength L (which is used in the question) and the linear momentum p:
L=hp
This indicates that the wavelength L is inversely proportional to the linear momentum p.
Step 3: Analyze the relationship
Since L is inversely proportional to p, we can write:
L∝1p
This means that as the linear momentum p increases, the wavelength L decreases, and vice versa.
Step 4: Determine the graph representation
Given the inverse relationship, we can conclude that the graph of L versus p will be a hyperbola, which typically has the following characteristics:
- As p increases, L decreases.
- As p approaches zero, L approaches infinity.
Step 5: Identify the correct graph
From the options provided in the question, the correct representation of the relationship L∝1p will be a graph that shows a decrease in L as p increases, which is characteristic of a hyperbolic curve.
Conclusion
Thus, the correct option representing the de-Broglie wavelength L associated with an elementary particle of linear momentum p is the one that shows an inverse relationship, typically represented by a hyperbola.
---
The de-Broglie wavelength λ is given by the formula:
λ=hp
where h is Planck's constant and p is the linear momentum of the particle.
From the formula, we can express the relationship between the wavelength L (which is used in the question) and the linear momentum p:
L=hp
This indicates that the wavelength L is inversely proportional to the linear momentum p.
Since L is inversely proportional to p, we can write:
L∝1p
This means that as the linear momentum p increases, the wavelength L decreases, and vice versa.
Given the inverse relationship, we can conclude that the graph of L versus p will be a hyperbola, which typically has the following characteristics:
- As p increases, L decreases.
- As p approaches zero, L approaches infinity.
From the options provided in the question, the correct representation of the relationship L∝1p will be a graph that shows a decrease in L as p increases, which is characteristic of a hyperbolic curve.
Thus, the correct option representing the de-Broglie wavelength L associated with an elementary particle of linear momentum p is the one that shows an inverse relationship, typically represented by a hyperbola.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The de Broglie wavelength associated with particle is
Ainversely proportional to its momentumBinversely proportional to its energyCdirectly proportional to its velocityDdirectly proportional to its momentumThe de -Brogile wavelength associated with a matter particle is
ADirectly proportional to the momentum of the particleBDirectly proportional to the velocity of the particleCInversely proportional to the velocity of the particleDInversely proportional to Planck's constantMinimum de-Broglie wavelength is associated with.
AElectronBprotonCCO2 moleculeDSO2 molecule
Minimum de-Broglie wavelength is associated with.
View SolutionThe de-Broglie wavelength associated with a particle of momentum p is given as :
View SolutionThe de - Broglie wavelength associated with the particle of mass m moving with velocity v is
View SolutionThe de-Broglie wavelength associated with an electron having a kinetic energy of 10 eV is
View Solutionde-Broglie wavelength associated with an electron at V potential difference is :
View Solution
