For a reaction A+2B→2C, the following data were obtained . Initial concentration
[A][B]Rate(moll−1−1min)i.1.01.00.15ii2.01.00.30iii.3.01.00.45iv.1.02.00.15v.1.03.00.15
The rate law for this reaction
R=k[A][B]0
R=k[A][B]2
R=k[A][B]
R=k[A]2[B]
The correct Answer is:A
To determine the rate law for the reaction A+2B→2C based on the provided data, we will follow these steps:
Step 1: Write the general rate law expression
The rate law for the reaction can be expressed as:
Rate=k(A)α(B)β
where k is the rate constant, α is the order with respect to A, and β is the order with respect to B.
Step 2: Analyze the data
We have the following experimental data:
| Experiment | (A) (mol/L) | (B) (mol/L) | Rate (mol/L/min) |
|------------|-------------|-------------|-------------------|
| (i) | 1.0 | 1.0 | 0.15 |
| (ii) | 2.0 | 1.0 | 0.30 |
| (iii) | 3.0 | 1.0 | 0.45 |
| (iv) | 1.0 | 2.0 | 0.15 |
| (v) | 1.0 | 3.0 | 0.15 |
Step 3: Determine the order with respect to A (α)
Using experiments (i) and (ii):
- For experiment (i):
0.15=k(1.0)α(1.0)β
- For experiment (ii):
0.30=k(2.0)α(1.0)β
Dividing the second equation by the first:
0.300.15=k(2.0)α(1.0)βk(1.0)α(1.0)β
2=(2.0)α(1.0)α⟹2=2α
Thus, α=1.
Step 4: Determine the order with respect to B (β)
Using experiments (iv) and (v):
- For experiment (iv):
0.15=k(1.0)α(2.0)β
- For experiment (v):
0.15=k(1.0)α(3.0)β
Dividing the fifth equation by the fourth:
0.150.15=k(1.0)α(3.0)βk(1.0)α(2.0)β
1=(3.0)β(2.0)β⟹1=(3.02.0)β
Since the left side is 1, this implies β=0.
Step 5: Write the final rate law
Now we can write the rate law as:
Rate=k(A)1(B)0=k(A)
Conclusion
The rate law for the reaction A+2B→2C is:
Rate=k(A)
The rate law for the reaction can be expressed as:
Rate=k(A)α(B)β
where k is the rate constant, α is the order with respect to A, and β is the order with respect to B.
We have the following experimental data:
|------------|-------------|-------------|-------------------|
| (i) | 1.0 | 1.0 | 0.15 |
| (ii) | 2.0 | 1.0 | 0.30 |
| (iii) | 3.0 | 1.0 | 0.45 |
| (iv) | 1.0 | 2.0 | 0.15 |
| (v) | 1.0 | 3.0 | 0.15 |
Using experiments (i) and (ii):
- For experiment (i):
0.15=k(1.0)α(1.0)β
- For experiment (ii):
0.30=k(2.0)α(1.0)β
0.300.15=k(2.0)α(1.0)βk(1.0)α(1.0)β
2=(2.0)α(1.0)α⟹2=2α
Thus, α=1.
Using experiments (iv) and (v):
- For experiment (iv):
0.15=k(1.0)α(2.0)β
- For experiment (v):
0.15=k(1.0)α(3.0)β
0.150.15=k(1.0)α(3.0)βk(1.0)α(2.0)β
1=(3.0)β(2.0)β⟹1=(3.02.0)β
Since the left side is 1, this implies β=0.
Now we can write the rate law as:
Rate=k(A)1(B)0=k(A)
The rate law for the reaction A+2B→2C is:
Rate=k(A)
Topper's Solved these Questions
Similar Questions
(a) Write the rate expression. (b) Find the rate constant for
([A]0,[B]0,,,R_(0)Rate(mollitre−1s−1)),(0.1,0.2,,,0.05),(0.2,0.2,,,0.10),(0.1,0.1,,,0.05):}
View SolutionFor a gaseous reaction 2A+B2→2AB, the following rate data were obtained at 300 K
Rate of disappearance of B2 Concentration(mol lit−1min−1)[A][B2](i)1.8×10−30.0150.15(ii)1.08×10−20.090.15(iii)5.4×10−30.0150.45
Calculate the rate constant for the reaction and rate of formation of AB when [A] is 0.02 and [B2] is 0.04 "mol lit"^(-1)" at 300 K".
View Solution
Knowledge Check
For the reaction : 2A+B→C+D, measurement of the rate of the reaction at varying concentrations are given below
Trial No.[A][B]rate(m mole L−1s−1)10.0100.0102.520.0100.0205.030.0300.02045.0
The rate law is thereforeArate=k[A]2[B]Brate =k[A][B]2Crate =k[A][B]Drate =k[A]2[B]2form the gaseous reaction
2A+B2→2AB, the following rate data were obtained at 300K.
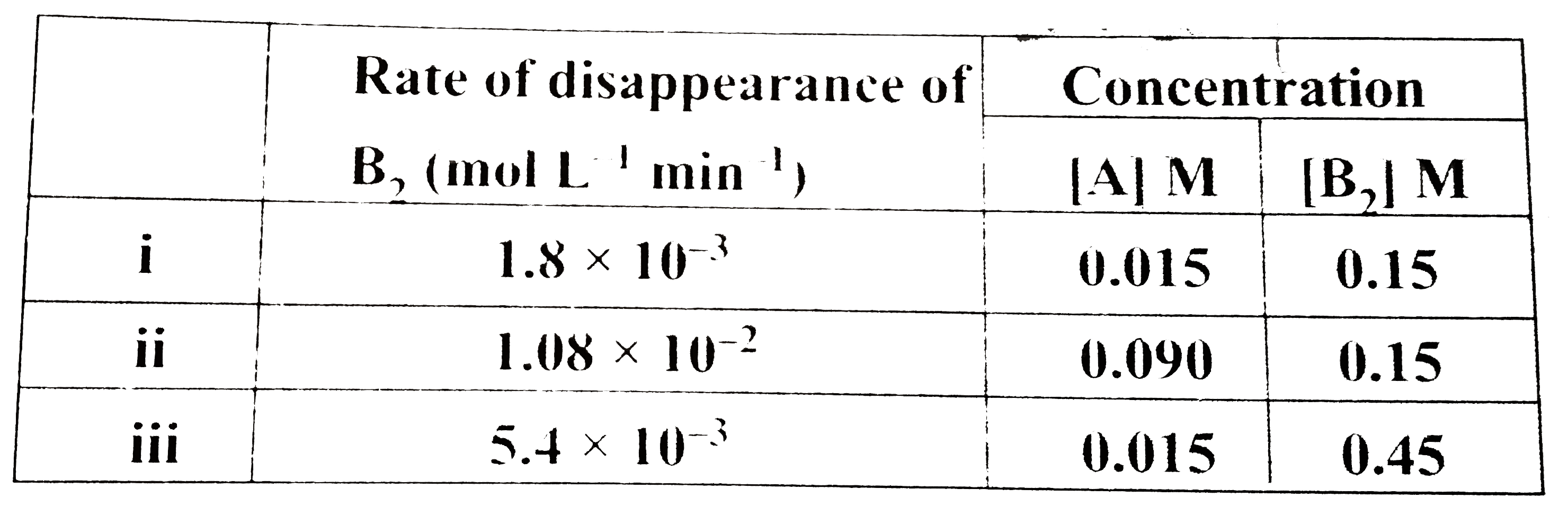
Calculate the rate constant for the reaction and the rate of formation of AB when [A] is 0.02 and [B2] is 0.04molL−1 at 300K.A0.5mol−1min−1litreB0.8mol−1min−1litreC1.5mol−1min−1litreD2mol−1min−1litreFor a hypothetical reaction, the following kinetic data suggested that the overall order of this reaction is
[A][B][C]mol dm−3mol dm−3mol dm−3s−12.01.01.02.04.02.01.04.01.0A3B1.5C1D0.5
For the reaction A + B → products, the following initial rates were obtained at various given initial concentration
S.No.[A]mol/L[B] mol/LInitial rate M/s1. 0.1 0.1 0.052. 0.2 0.1 0.103. 0.1 0.2 0.05
Determine the half-life period.
View SolutionThe following data are for the reaction A+B→ Products:
Conc.ofA(M)Conc.ofB(M)Initialrate(molL−1s−1)0.10.14.0×10−40.20.21.6×10−30.50.11.0×10−20.50.55.0×10−2
(a) What is the order with respect to A and B for the reaction?
(b) Calculate the rate constant
Determine the reaction rate when the concentrations of A and B are 0.2M and 0.35M respectvely.
View SolutionFor the given reaction ,
A+B→ Products
Following data are given :
initial conc.(m/L)initial conc.(m/L)initial conc.(mL−1S−1)[A]0[B]00.10.10.050.20.10.10.10.20.05
calculate the Rate constant
View SolutionFor the non-stoichiometric reaction 2A+B→C+D
The following kinetic data were obtained in theee separate experiment, all at 98K
∣∣ ∣ ∣ ∣ ∣∣Initial concentration (A)Initial concentration (B)Initial rate of formation of C(molL−1s−1)0.01M0.1M1.2×10−30.1M0.2M1.2×10−30.2M0.1M2.4×10−3∣∣ ∣ ∣ ∣ ∣∣
The rate law for the formation of C is:
View SolutionDuring the kinetic study of the reaction, 2A+B→C+D , following results were obtained
Run[A]/molL−1[B]molL−1Initial rate formation of D/molL−1min−1I0.10.16.0×10−3II0.30.27.2×10−2III0.30.42.88×10−1IV0.40.12.40×10−2
Based on the above data which one of the following is correct ?
View Solution
