Doubtnut Promotions Sticky

The vector difference between the absolute velocities of two bodies is called their relative velocity. The concept of relative velocity enables us to treat one body as being at rest while the other is in motion with the relative velocity. This jormalism greatly simplifies many problems. If →vAis the absolute velocity of a body A and →–––(B)that of another body B then the relative velocity of A in relation to B is →–––(AD)=→VA−→vB and →vBA=→vB-→vA.
The principle follows here is that the relative velocity of two bodies remains unchaged if the same additional velocity is imparted to both the bodies. A simple way of carrying out this operation is to be represent the velocities in magnitude and direction from a common point. Then the line joining the tips of the vectors represents the relative velocity
Q A river is flowing from west to east at a speed of 5 m/s. A man on the south bank of the river, capable of swimming at 10 m/s in still water, wants to cross the river without drifting, he. should swim
The vector difference between the absolute velocities of two bodies is called their relative velocity. The concept of relative velocity enables us to treat one body as being at rest while the other is in motion with the relative velocity. This jormalism greatly simplifies many problems. If vecv(A)is the absolute velocity of a body A and vec(B)that of another body B then the relative velocity of A in relation to B is vec(AD)=vecV(A)-vecv(B) and vecv(BA)=vecv(B)-vecv(A). The principle follows here is that the relative velocity of two bodies remains unchaged if the same additional velocity is imparted to both the bodies. A simple way of carrying out this operation is to be represent the velocities in magnitude and direction from a common point. Then the line joining the tips of the vectors represents the relative velocity Q A river is flowing from west to east at a speed of 5 m/s. A man on the south bank of the river, capable of swimming at 10 m/s in still water, wants to cross the river without drifting, he. should swim

A

due north

B

30∘ east of north

C

30∘ west of north

D

60∘ east of north

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

|
Answer

Step by step video, text & image solution for The vector difference between the absolute velocities of two bodies is called their relative velocity. The concept of relative velocity enables us to treat one body as being at rest while the other is in motion with the relative velocity. This jormalism greatly simplifies many problems. If vecv_(A)is the absolute velocity of a body A and vec_(B)that of another body B then the relative velocity of A in relation to B is vec_(AD)=vecV_(A)-vecv_(B) and vecv_(BA)=vecv_(B)-vecv_(A). The principle follows here is that the relative velocity of two bodies remains unchaged if the same additional velocity is imparted to both the bodies. A simple way of carrying out this operation is to be represent the velocities in magnitude and direction from a common point. Then the line joining the tips of the vectors represents the relative velocity Q A river is flowing from west to east at a speed of 5 m/s. A man on the south bank of the river, capable of swimming at 10 m/s in still water, wants to cross the river without drifting, he. should swim by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023
Class 12PHYSICSKINEMATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    The vector difference between the absolute velocities of two bodies is called their relative velocity. The concept of relative velocity enables us to treat one body as being at rest while the other is in motion with the relative velocity. This jormalism greatly simplifies many problems. If →vAis the absolute velocity of a body A and →–––(B)that of another body B then the relative velocity of A in relation to B is →–––(AD)=→VA−→vB and →vBA=→vB-→vA.
    The principle follows here is that the relative velocity of two bodies remains unchaged if the same additional velocity is imparted to both the bodies. A simple way of carrying out this operation is to be represent the velocities in magnitude and direction from a common point. Then the line joining the tips of the vectors represents the relative velocity
    Q A train A moves to the east with velocity of 40 km/hr and a train B moves due north with velocity of 30 km/hr. The relative velocity of A w.rt. B is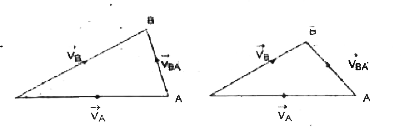
    ocr_image

    A70 km/hr towards East
    B10 km/hr towards North East
    C50 km/hr at some angle South of East
    D50 km/hr at some angle North of East.
  • Question 2 - Select One

    In perfectily inelastaic collition the relative velocity of the bodies

    Abefore impect it zero
    Bbefore impect is equal the after impact
    Cbefore impect is zero
    Dis characterized by none of the above
  • Question 3 - Select One

    In the above question, if another body is at rest, then velocity of the compound body after collision is

    Av/2
    B2v
    Cv
    Dzero