Question

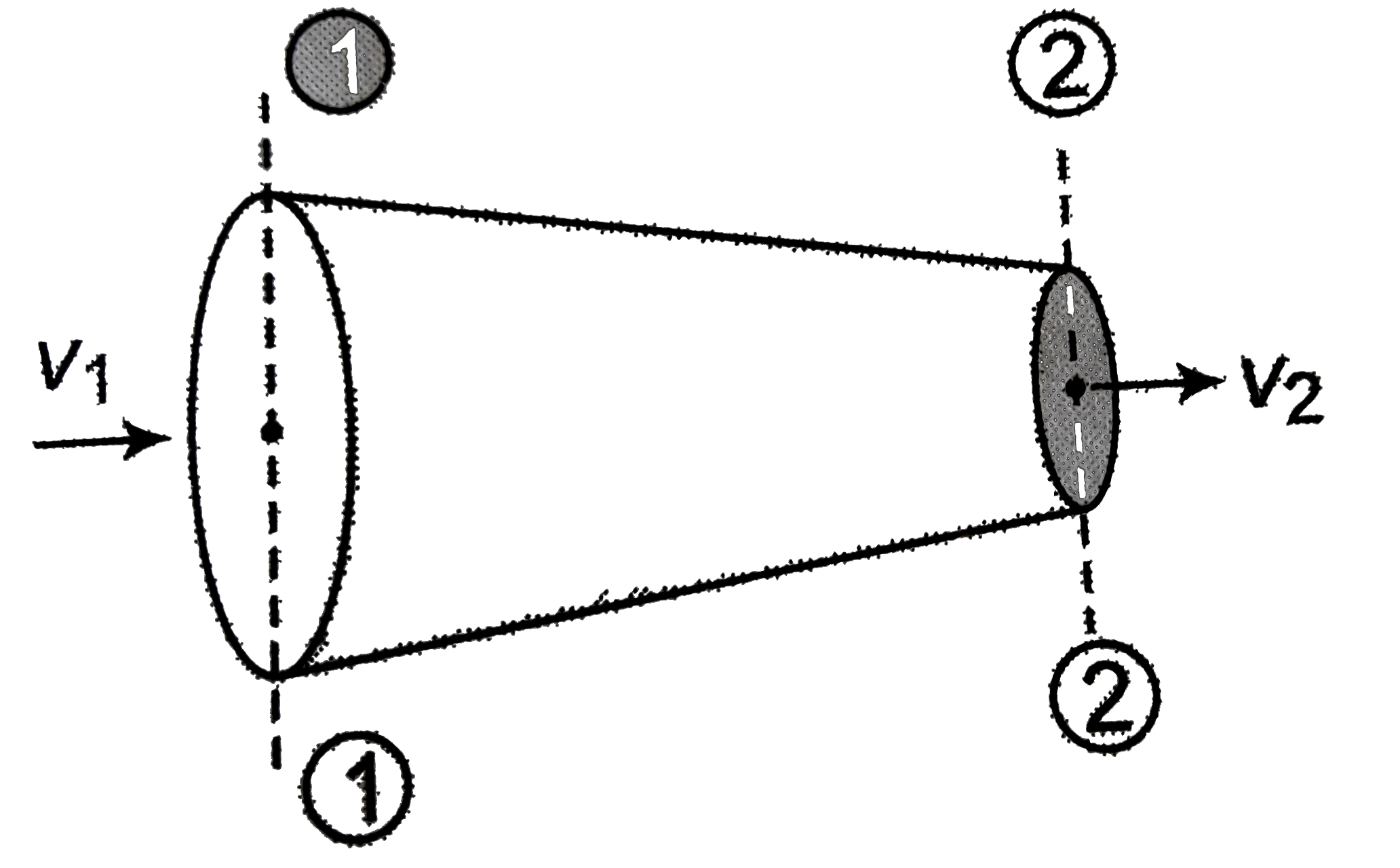
Water is flowing steadily through a horizontal pipe of non - uniform cross - section . If the velocity of water cross - section is $0.02m^2$ is 2m/s , what is the velocity of water at another point where the cross - section is $0.01m^2$ ?

Options

A. 1 m/s
B. 2 m/s
C. 3 m/s
D. 4 m/s

Answer

D

Explanation

To solve the problem of finding the velocity of water at a different cross-section of a pipe, we can use the principle of conservation of mass, which is represented by the continuity equation. Here’s a step-by-step solution:

Step 1: Understand the Continuity Equation
The continuity equation states that for an incompressible fluid flowing through a pipe, the product of the cross-sectional area (A) and the velocity (V) at one point is equal to the product of the cross-sectional area and velocity at another point. Mathematically, this can be expressed as:
\( A_1 V_1 = A_2 V_2 \)

Step 2: Identify the Given Values
From the problem, we have:
- \( A_1 = 0.02 \, m^2 \) (cross-sectional area at point 1)
- \( V_1 = 2 \, m/s \) (velocity at point 1)
- \( A_2 = 0.01 \, m^2 \) (cross-sectional area at point 2)
- We need to find \( V_2 \) (velocity at point 2).

Step 3: Substitute the Known Values into the Continuity Equation
Using the continuity equation:
\( A_1 V_1 = A_2 V_2 \)
Substituting the known values:
\( 0.02 \, m^2 \times 2 \, m/s = 0.01 \, m^2 \times V_2 \)

Step 4: Solve for \( V_2 \)
Now, we can solve for \( V_2 \):
\( 0.04 \, m^3/s = 0.01 \, m^2 \times V_2 \)
To isolate \( V_2 \), divide both sides by \( 0.01 \, m^2 \):
\( V_2 = \frac{0.04 \, m^3/s}{0.01 \, m^2} \)
\( V_2 = 4 \, m/s \)

Step 5: Conclusion
The velocity of water at the cross-section where the area is \( 0.01 \, m^2 \) is \( 4 \, m/s \).