Question

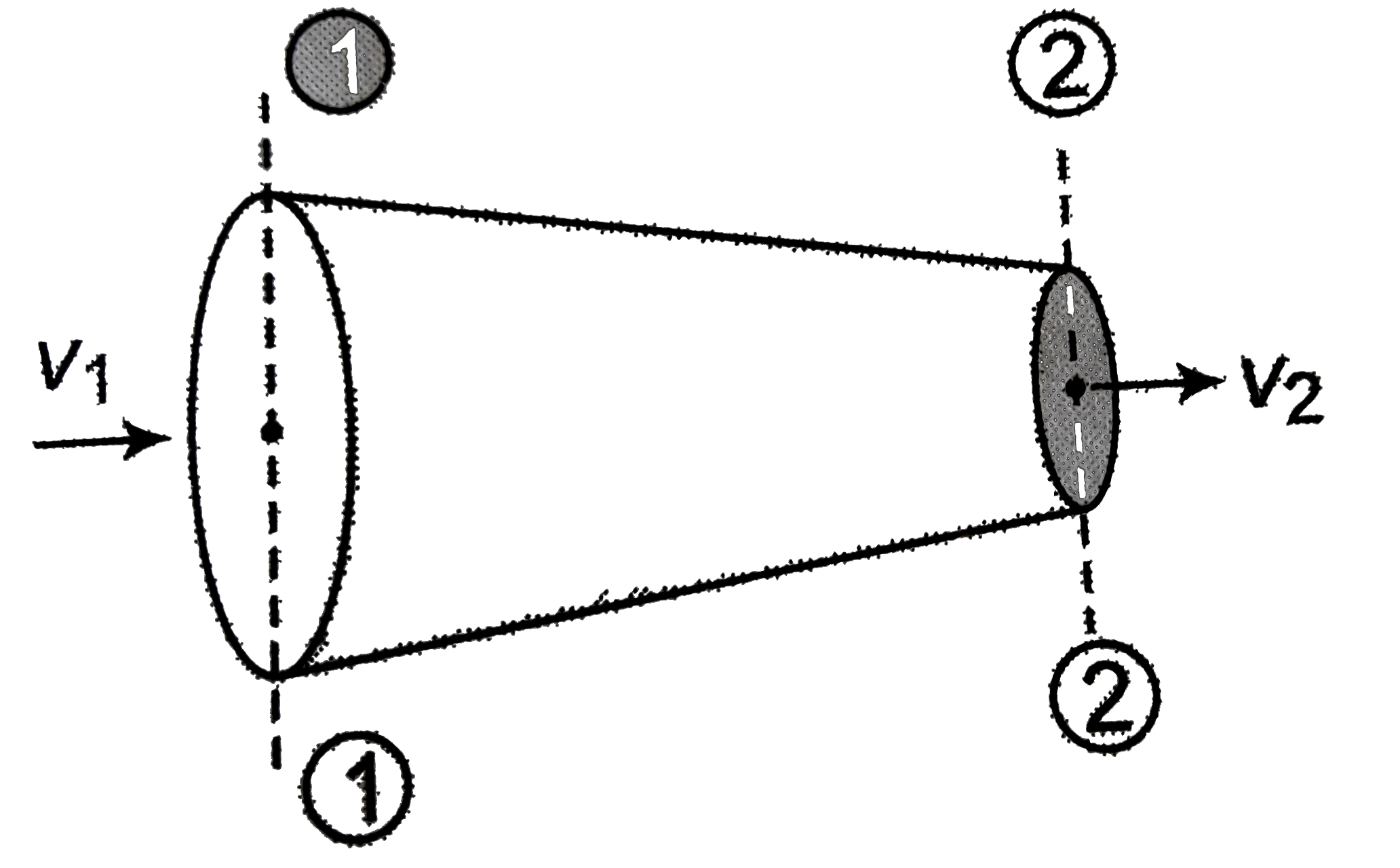
Water is flowing through a horizontal pipe of varying cross-section. If the pressure of water equals 2 cm of Hg where velocity of flow is $32cm{s}^{-1}$, what is the pressure at another point where the velocity of flow is $40cm{s}^{-1}$?

Explanation

To solve the problem, we will apply Bernoulli's principle, which states that for an incompressible, frictionless fluid, the total mechanical energy along a streamline is constant. The equation can be expressed as:

$$P+\frac{1}{2}\rho V^2+\rho gh=\text{constant}$$

Since the pipe is horizontal, the height (h) remains constant, and we can ignore the potential energy term ($$\rho gh$$). Therefore, the equation simplifies to:

$$P_1+\frac{1}{2}\rho V_1^2=P_2+\frac{1}{2}\rho V_2^2$$

Step 1: Identify the known values
- \( P_1 = 2 \, \text{cm of Hg} \)
- \( V_1 = 32 \, \text{cm/s} \)
- \( V_2 = 40 \, \text{cm/s} \)

Step 2: Convert pressure from cm of Hg to a consistent unit
The density of mercury (\( \rho_{Hg} \)) is \( 13.6 \, \text{g/cm}^3 \). We will convert the pressure into dynes/cm² for consistency:
\( P_1 = 2 \, \text{cm of Hg} = 2 \times 13.6 \times 980 \, \text{dynes/cm}^2 \)

Step 3: Substitute known values into Bernoulli's equation
Using the simplified Bernoulli's equation:
\( P_1 + \frac{1}{2} \rho V_1^2 = P_2 + \frac{1}{2} \rho V_2^2 \)

Rearranging for \( P_2 \):
\( P_2 = P_1 + \frac{1}{2} \rho V_1^2 - \frac{1}{2} \rho V_2^2 \)

Step 4: Substitute the values into the equation
Substituting \( P_1 \) and the velocities:
\( P_2 = 2 \times 13.6 \times 980 + \frac{1}{2} \rho (32^2) - \frac{1}{2} \rho (40^2) \)

Step 5: Calculate the kinetic energy terms
The density of water (\( \rho \)) is \( 1 \, \text{g/cm}^3 \), so:
\( \frac{1}{2} \rho (32^2) = \frac{1}{2} \times 1 \times 1024 = 512 \, \text{dynes/cm}^2 \)
\( \frac{1}{2} \rho (40^2) = \frac{1}{2} \times 1 \times 1600 = 800 \, \text{dynes/cm}^2 \)

Step 6: Substitute kinetic energy terms back into the equation
Now substituting these values back:
\( P_2 = 2 \times 13.6 \times 980 + 512 - 800 \)

Step 7: Calculate \( P_2 \)
Calculating the pressure:
1. Calculate \( 2 \times 13.6 \times 980 \):
\( 2 \times 13.6 \times 980 = 26656 \, \text{dynes/cm}^2 \)
2. Now substitute:
\( P_2 = 26656 + 512 - 800 \)
\( P_2 = 26656 - 288 \)
\( P_2 = 26368 \, \text{dynes/cm}^2 \)

Step 8: Convert \( P_2 \) back to cm of Hg
To convert back to cm of Hg:
\( P_2 \text{ in cm of Hg} = \frac{26368}{13.6 \times 980} \)

Calculating:
1. Calculate \( 13.6 \times 980 = 13328 \)
2. Now divide:
\( P_2 = \frac{26368}{13328} \approx 1.978 \, \text{cm of Hg} \)

Final Answer
Thus, the pressure at the point where the velocity of flow is \( 40 \, \text{cm/s} \) is approximately:
\( P_2 \approx 1.978 \, \text{cm of Hg} \)

---