The gravitational field strength →E and gravitational potential V are releated as
→E=−(δVδxˆi+δVδyˆj+δVδzˆk)
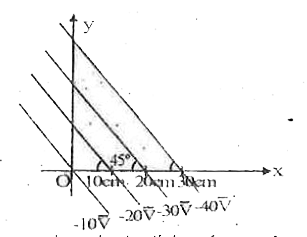
In the figure, transversal lines represent equipotential surfaces. A particle of mass m is released from rest at the origin. The gravitational unit of potential , 1→V=1cm2/s2
y-component of E a the point whose co-ordinates are (4cm,4cm) is
1cm/s2
8cm/s2
4cm/s2
none of these
The correct Answer is:A
Step by step video, text & image solution for The gravitational field strength vecE and gravitational potential V are releated as vecE=-((deltaV)/(deltax)hati+(deltaV)/(deltay)hatj+(deltaV)/(deltaz)hatk) In the figure, transversal lines represent equipotential surfaces. A particle of mass m is released from rest at the origin. The gravitational unit of potential , 1vecV=1cm^(2)//s^(2) y-component of E a the point whose co-ordinates are (4cm,4cm) is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - GRAVITATIONEXERCISE - Assertion & Reasoning type1videos
GRAVITATION
- BOOK - FIITJEECHAPTER - GRAVITATIONEXERCISE - Numerical based Questions4videos
GRAVITATION
- BOOK - FIITJEECHAPTER - GRAVITATIONEXERCISE - Solved problem (Objective)15videos
GRAVITATION
- BOOK - FIITJEECHAPTER - GMP ASSESMENTEXERCISE - Numerical Based61videos
GMP ASSESMENT
- BOOK - FIITJEECHAPTER - HEAT AND TEMPERATUREEXERCISE - NUMERICAL BASES QUESTIONS1videos
HEAT AND TEMPERATURE
Similar Questions
A particle of mass 1 kg is released from rest at a point where gravitational potential is 5 J/kg. After some time, it passes through a point B with a speed of 1 m/s. What is the gravitational potential at B ?
View SolutionThe gravitational field in a region is given by the equation E=(5i+12j)N/kg. If a particle of mass 2kg is moved from the origin to the point (12m,5m) in this region, the change in the gravitational potential energy is
View Solution
Knowledge Check
- Question 1 - Select One
The gravitational field strength →E and gravitational potential V are releated as
→E=−(δVδxˆi+δVδyˆj+δVδzˆk)
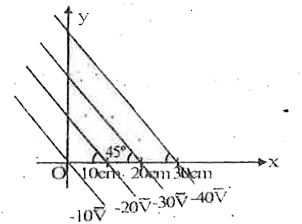
In the figure, transversal lines represent equipotential surfaces. A particle of mass m is released from rest at the origin. The gravitational unit of potential , 1→V=1cm2/s2
x-component of the velocity of the particle at the point (4cm,4cm) isA4cm/sB2cm/sC2√2cmsD1cm/s - Question 2 - Select One
The gravitational field strength →E and gravitational potential V are releated as
→E=−(δVδxˆi+δVδyˆj+δVδzˆk)
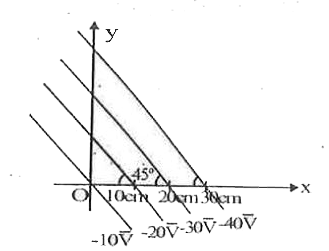
In the figure, transversal lines represent equipotential surfaces. A particle of mass m is released from rest at the origin. The gravitational unit of potential , 1→V=1cm2/s2
x-component of the velocity of the particle at the point (4cm,4cm) isA4cm/sB2cm/sC2√2cmsD1cm/s - Question 3 - Select One
The gravitational field strength →E and gravitational potential V are releated as
→E=−(δVδxˆi+δVδyˆj+δVδzˆk)
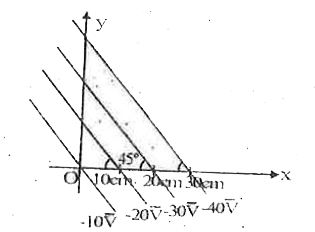
In the figure, transversal lines represent equipotential surfaces. A particle of mass m is released from rest at the origin. The gravitational unit of potential , 1→V=1cm2/s2
Speed of the particle (v) (y is in cm and v in cm/s) as function of its y-co-ordinate isAv=2√yBv=√2yCv=2yDv=2y+4y2+2y2
A particle of mass 1kg moves along the curve y=x2 Magnitude of angular momentum of the particle about origin,when particle has x co-ordinate is 12m and x-component of velocity is 4m/s is
View SolutionThe gravitational field strength →E and gravitational potential V are releated as
→E=−(δVδxˆi+δVδyˆj+δVδzhark)
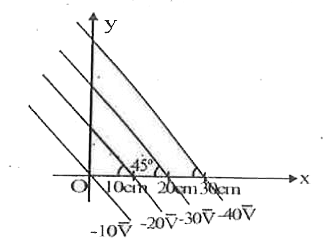
In the figure, transversal lines represent equipotential surfaces. A particle of mass m is released from rest at the origin. The gravitational unit of potential , 1→V=1cm2/s2
Speed of the particle (v) (y is in cm and v is in cm//s) as function of its y-co ordinate isView SolutionWe know that electric field (E ) at any point in space can be calculated using the relation
→E=−δVδxˆi−δVδyˆj−δVδzˆk

if we know the variation of potential (V) at that point. Now let the electric potential in volt along the x-axis vary as V=2x2, where x is in meter. Its variation is as shown in figure
Draw the variation of electric field (E ) along the x-axis.View SolutionWe know that electric field (E ) at any point in space can be calculated using the relation
→E=−δVδxˆi−δVδyˆj−δVδzˆk

if we know the variation of potential (V) at that point. Now let the electric potential in volt along the x-axis vary as V=2x2, where x is in meter. Its variation is as shown in figure
Will the particle perform a simple harmonic motion? Also, find the time period of its oscillations.View SolutionWe know that electric field (E ) at any point in space can be calculated using the relation
→E=−δVδxˆi−δVδyˆj−δVδzˆk

if we know the variation of potential (V) at that point. Now let the electric potential in volt along the x-axis vary as V=2x2, where x is in meter. Its variation is as shown in figure
A charge particle of mass 10 mg and charge 2.5μC is released from rest at x=2m. Find its velocity when it crosses origin.View Solution

