A particle describes uniform circular motion. Find the torque on it about the centre of the circle.
Video Solution
Text Solution
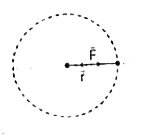
τ=→r×→F, where F = net force.
In uniform circular motion →rand→F are collinear (but oppositely directed)
⇒ →r×→F=0
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE ) LEVEL - I15videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE ) LEVEL - II & III15videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - NUMERICALS BASED QUESTIONS4videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - PHYSICS PART2EXERCISE - Numerical Based Question Decimal Type6videos
PHYSICS PART2
- BOOK - FIITJEECHAPTER - SEMICONDUCTOR AND DEVICEEXERCISE - SOLVED PROBLEMS Objective: Level-I20videos
SEMICONDUCTOR AND DEVICE
Similar Questions
Knowledge Check
- Question 1 - Select One
In uniform circular motion
AAcceleration is constantBMagnitude of acceleration is contantCTangential acceleration is zeroDTangential acceleration is not zero - Question 2 - Select One
In uniform circular motion
AAcceleration is constantBMagnitude of acceleration is contantCTangential acceleration is zeroDTangential acceleration is not zero - Question 3 - Select One or More
In uniform circular motion
AAcceleration is constantBMagnitude of acceleration is contantCTangential acceleration is zeroDTangential acceleration is not zero

