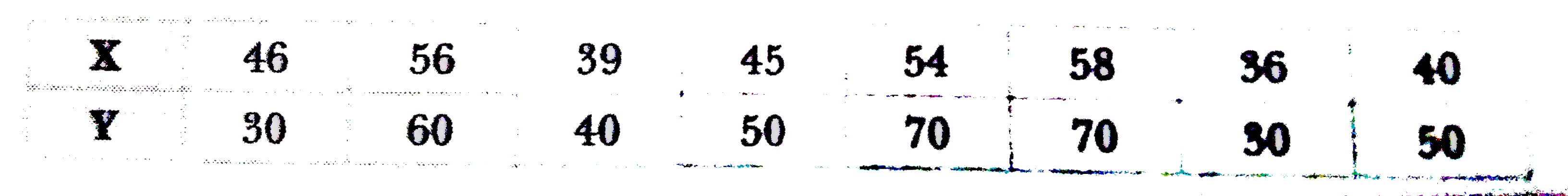Find the coefficient of correlation e(X,Y), when cov (X,Y) =-14.6, var(X)=3.5 var(Y)=64.
To find the coefficient of correlation e(X,Y), we will use the formula:
e(X,Y)=cov(X,Y)√var(X)⋅var(Y)
Step 1: Identify the given values
We are given:
- Covariance cov(X,Y)=−14.6
- Variance var(X)=3.5
- Variance var(Y)=64
Step 2: Substitute the values into the formula
Substituting the given values into the formula:
e(X,Y)=−14.6√3.5⋅64
Step 3: Calculate the product of the variances
Now, calculate var(X)⋅var(Y):
3.5⋅64=224
Step 4: Calculate the square root of the product
Next, we find the square root of 224:
√224≈14.97
Step 5: Substitute back into the formula
Now, substitute this value back into the formula for the coefficient of correlation:
e(X,Y)=−14.614.97
Step 6: Perform the division
Now, perform the division:
e(X,Y)≈−0.975
Final Answer
Thus, the coefficient of correlation e(X,Y) is approximately:
e(X,Y)≈−0.975
---
We are given:
- Covariance cov(X,Y)=−14.6
- Variance var(X)=3.5
- Variance var(Y)=64
Substituting the given values into the formula:
Now, calculate var(X)⋅var(Y):
Next, we find the square root of 224:
Now, substitute this value back into the formula for the coefficient of correlation:
Now, perform the division:
Thus, the coefficient of correlation e(X,Y) is approximately:
Topper's Solved these Questions
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Solved Problems(Subjective)
9 Videos
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Solved Problems(Objective)
24 Videos
- SET, RELATION & FUNCTIONBOOK - FIITJEECHAPTER - SET, RELATION & FUNCTIONEXERCISE - Exercise 3
8 Videos
- STRAIGHT LINE BOOK - FIITJEECHAPTER - STRAIGHT LINE EXERCISE - NUMERICAL BASED
4 Videos
Similar Questions
Knowledge Check
Find the coefficient of x6y3 in the expansion of (x+2y).
A972B9C323C9C3D152Find the coefficient of x3y∈(x+2y)×(5x+y)3
A250B175C475D325If the coefficient of correlation between x and y os 0.6, covariance is 27 and variance of y is 25, then what is the variance x?
A9/5B81/25C9D81
Calculate coefficient of correlation between series X and Y.

View SolutionThe coefficient of correlation between X and Y is 0.6. Their covariance is 4.8 var (x)=9. Find the value of σy.
View SolutionIf x and y are two uncorrelated variables such that u=x+y and v=x-y, find the coefficient of correlation betweeen u and v in terms of σxandσy.
View SolutionFind the Kari Pearson's Coefficient of correlation between x and y for the data

View SolutionFind the coefficient of correlation between the values of x and y :
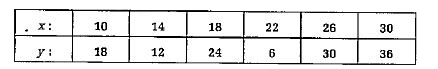
Note : Here,values in x, increase by the interval of 4 and values of y have the common factor 6.
View Solution