Karl Pearlon's coefficient of skewness of a distribution is 0.32. Its S.D. is 6.5 and mean 39.6. The median of the distribution is given by
28.61
38.91
29.13
28.31
To find the median of the distribution given Karl Pearson's coefficient of skewness, we can use the formula for skewness:
Skewness=3(Mean−Median)Standard Deviation
Given:
- Skewness = 0.32
- Mean = 39.6
- Standard Deviation (SD) = 6.5
We can rearrange the formula to solve for the median:
0.32=3(39.6−Median)6.5
Step 1: Multiply both sides by 6.5
0.32×6.5=3(39.6−Median)
Calculating the left side:
0.32×6.5=2.08
So we have:
2.08=3(39.6−Median)
Step 2: Divide both sides by 3
2.083=39.6−Median
Calculating the left side:
2.083≈0.6933
So we have:
0.6933=39.6−Median
Step 3: Rearranging to find the Median
Median=39.6−0.6933
Calculating the right side:
Median≈39.6−0.6933≈38.9067
Rounding to one decimal place, we find:
Median≈38.9
Thus, the median of the distribution is approximately 38.9.
- Skewness = 0.32
- Mean = 39.6
- Standard Deviation (SD) = 6.5
Topper's Solved these Questions
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Exercise 1
5 Videos
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Exercise 2
4 Videos
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Solved Problems(Subjective)
9 Videos
- SET, RELATION & FUNCTIONBOOK - FIITJEECHAPTER - SET, RELATION & FUNCTIONEXERCISE - Exercise 3
8 Videos
- STRAIGHT LINE BOOK - FIITJEECHAPTER - STRAIGHT LINE EXERCISE - NUMERICAL BASED
4 Videos
Similar Questions
Knowledge Check
Karl Pearson's coefficient of skewness of a distribution is 0⋅32. Its s.d. is 6⋅5 and mean is 29⋅6. The mode and median of the distribution are
A27⋅52,28.91B26⋅91,27⋅23C25⋅67,26⋅34Dnone of theseFind the median of the distribution. 5, 9, 4, 6, 12, 8
A7B9C6D5What is the median of the distribution 3, 7, 6, 9, 5,4, 2?
A5B6C7D8
What is the median of the distribution 3, 7, 6, 9, 5, 4, 2?
View SolutionThe mode of a distribution is 24 and the mean is 60. Whatis its median?
View SolutionIf the mean of the distribution
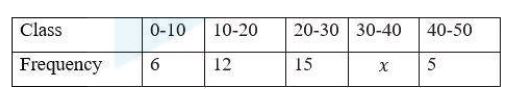
is 24.6, then the value of x is
View SolutionIf for a moderately skewed distribution, mode = 60 and mean = 66, then median =
View SolutionSeven of the eight numbers in a distribution are 11, 16, 6, 10, 13, 11, 13
Given that the median of the distribution is 12, find the mean of the distribution.
View Solution
