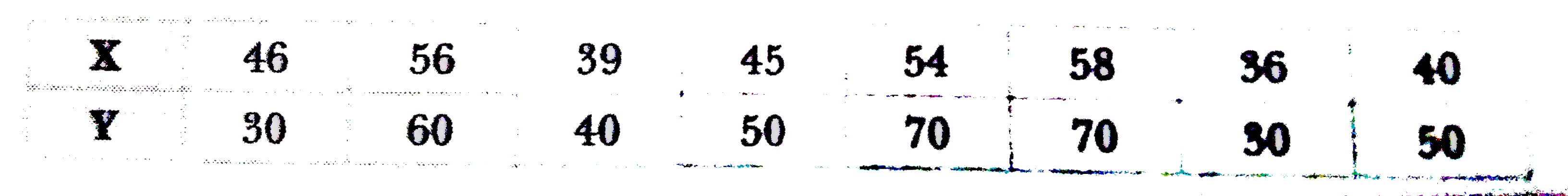The coefficient of correlation between X and Y is 0.6 U and V are two variables defined as U=x−32,V=y−23, then the coefficient of correlation between U and V is
0.1
0.3
0.2
1
The correct Answer is:A
To find the coefficient of correlation between the variables U and V, we can use the following steps:
Step 1: Understand the relationship between X, Y, U, and V
We are given that the coefficient of correlation between X and Y is rXY=0.6. The variables U and V are defined as:
U=X−32
V=Y−23
Step 2: Identify the transformation
The transformations for U and V can be expressed in terms of X and Y:
- U is a linear transformation of X (specifically, it is scaled and shifted).
- V is a linear transformation of Y (also scaled and shifted).
Step 3: Use the property of correlation coefficients
The correlation coefficient remains unchanged under linear transformations. Specifically, if we have:
U=aX+bandV=cY+d
then the correlation coefficient between U and V is given by:
rUV=rXY√(a2)(c2)
where a and c are the scaling factors of the transformations.
Step 4: Identify the scaling factors
From the definitions of U and V:
- For U: a=12
- For V: c=13
Step 5: Calculate the coefficient of correlation between U and V
Using the property of correlation coefficients:
rUV=rXY⋅1√(a2)(c2)
Substituting the values:
rUV=0.6⋅1√(12)2⋅(13)2
Calculating the denominator:
√(12)2⋅(13)2=√14⋅19=√136=16
Thus:
rUV=0.6⋅116=0.6⋅6=3.6
Final Answer
The coefficient of correlation between U and V is 3.6.
We are given that the coefficient of correlation between X and Y is rXY=0.6. The variables U and V are defined as:
U=X−32
V=Y−23
The transformations for U and V can be expressed in terms of X and Y:
- U is a linear transformation of X (specifically, it is scaled and shifted).
- V is a linear transformation of Y (also scaled and shifted).
The correlation coefficient remains unchanged under linear transformations. Specifically, if we have:
U=aX+bandV=cY+d
then the correlation coefficient between U and V is given by:
rUV=rXY√(a2)(c2)
where a and c are the scaling factors of the transformations.
From the definitions of U and V:
- For U: a=12
- For V: c=13
Using the property of correlation coefficients:
rUV=rXY⋅1√(a2)(c2)
Substituting the values:
rUV=0.6⋅1√(12)2⋅(13)2
Calculating the denominator:
√(12)2⋅(13)2=√14⋅19=√136=16
Thus:
rUV=0.6⋅116=0.6⋅6=3.6
The coefficient of correlation between U and V is 3.6.
Topper's Solved these Questions
- STATISTICSBook:FIITJEEChapter:STATISTICSExercise:Assignment Problems (Objective) Level II
Explore 25 Videos
- STATISTICSBook:FIITJEEChapter:STATISTICSExercise:Assertion -Reasoning Type MCQ Single Correct(Level II)
Explore 2 Videos
- STATISTICSBook:FIITJEEChapter:STATISTICSExercise:Assignment Problems (Subjective)
Explore 14 Videos
- SET, RELATION & FUNCTIONBook:FIITJEEChapter:SET, RELATION & FUNCTIONExercise:Exercise 3
Explore 8 Videos
- STRAIGHT LINE Book:FIITJEEChapter:STRAIGHT LINE Exercise:NUMERICAL BASED
Explore 4 Videos
Similar Questions
Knowledge Check
If r is the coefficient of correlation between two variable, then
Ar≥1Br≤1C|r|≤1D|r|≥1Let the correlation coefficient between X and Y be 0.6, random variable Z and W are defined as Z=X+5 and W=y3 What is the correlation coefficient between Z and W
A0.1B0.2C0.36D0.6If the coefficient of correlation between x and y os 0.6, covariance is 27 and variance of y is 25, then what is the variance x?
A9/5B81/25C9D81
If the two lines of regression are 3x+2y=26and6x+y=31, then the coefficient of correlation between x and y is ……………..
View SolutionFind the Kari Pearson's Coefficient of correlation between x and y for the data

View SolutionThe coefficient of correlation between X and Y is 0.6. Their covariance is 4.8 var (x)=9. Find the value of σy.
View SolutionIf x and y are two uncorrelated variables such that u=x+y and v=x-y, find the coefficient of correlation betweeen u and v in terms of σxandσy.
View SolutionLet the correlation coefficient between X and Y be 0.6. Random variables Z and W are defined as Z=X+5andW=Y3. What is the correlation coefficient between Z and W?
View Solution