A conducting wire carries a current of 0.965 ampere. Rate of flow of electrons per second at a given point is :
1×10−5NA
NA
0.965NA
NA0.965
The correct Answer is:A
To find the rate of flow of electrons per second in a conducting wire carrying a current of 0.965 amperes, we can follow these steps:
Step 1: Understand the Relationship Between Current and Charge
The current (I) in a wire is defined as the amount of charge (Q) that passes through a point in the wire per unit time (t). The formula for current is given by:
I=Qt
Where:
- I is the current in amperes (A),
- Q is the charge in coulombs (C),
- t is the time in seconds (s).
Step 2: Calculate the Charge Flowing in One Second
Since the current is given as 0.965 A and we are considering a time period of 1 second, we can rearrange the formula to find the charge:
Q=I×t
Substituting the values:
Q=0.965A×1s=0.965C
Step 3: Determine the Charge of a Single Electron
The charge of a single electron (e) is approximately:
e=1.6×10−19C
Step 4: Calculate the Number of Electrons Flowing Per Second
To find the number of electrons (n) flowing per second, we can use the formula:
n=Qe
Substituting the values we have:
n=0.965C1.6×10−19C
Step 5: Perform the Calculation
Calculating the above expression:
n=0.9651.6×10−19
n≈6.03125×1018
Step 6: Round the Result
We can round this to:
n≈6.03×1018
Conclusion
The rate of flow of electrons per second at a given point in the wire is approximately:
6.03×1018electrons/second
---
The current (I) in a wire is defined as the amount of charge (Q) that passes through a point in the wire per unit time (t). The formula for current is given by:
I=Qt
Where:
- I is the current in amperes (A),
- Q is the charge in coulombs (C),
- t is the time in seconds (s).
Since the current is given as 0.965 A and we are considering a time period of 1 second, we can rearrange the formula to find the charge:
Q=I×t
Substituting the values:
Q=0.965A×1s=0.965C
The charge of a single electron (e) is approximately:
e=1.6×10−19C
To find the number of electrons (n) flowing per second, we can use the formula:
n=Qe
Substituting the values we have:
n=0.965C1.6×10−19C
Calculating the above expression:
n=0.9651.6×10−19
n≈6.03125×1018
We can round this to:
n≈6.03×1018
The rate of flow of electrons per second at a given point in the wire is approximately:
6.03×1018electrons/second
Similar Questions
Knowledge Check
- Question 1 - Select One
An amperes is a rate of flow of electric current of
Aone faraday per secondBone coulomb per secondCone coulomb per minutesDone faraday per minutes - Question 2 - Select One
A metal wire carries a current of 1 ampere. The number of electrons passing a given point in the wire in 1 second is
A6.24×1020B6.24×1016C6.24×1018D6.24×1014
A long copper wire carries a current of 10 ampere . Calculate the magnetic flux per metre of the wire for a plane surface S inside the wire as shown in the figure.
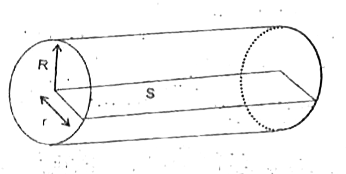 View Solution
View SolutionA long copper wire carries a current of I ampere. Calculate the magnetic flux per meter of the wire for a plane surface S inside the wire as shown in Fig.
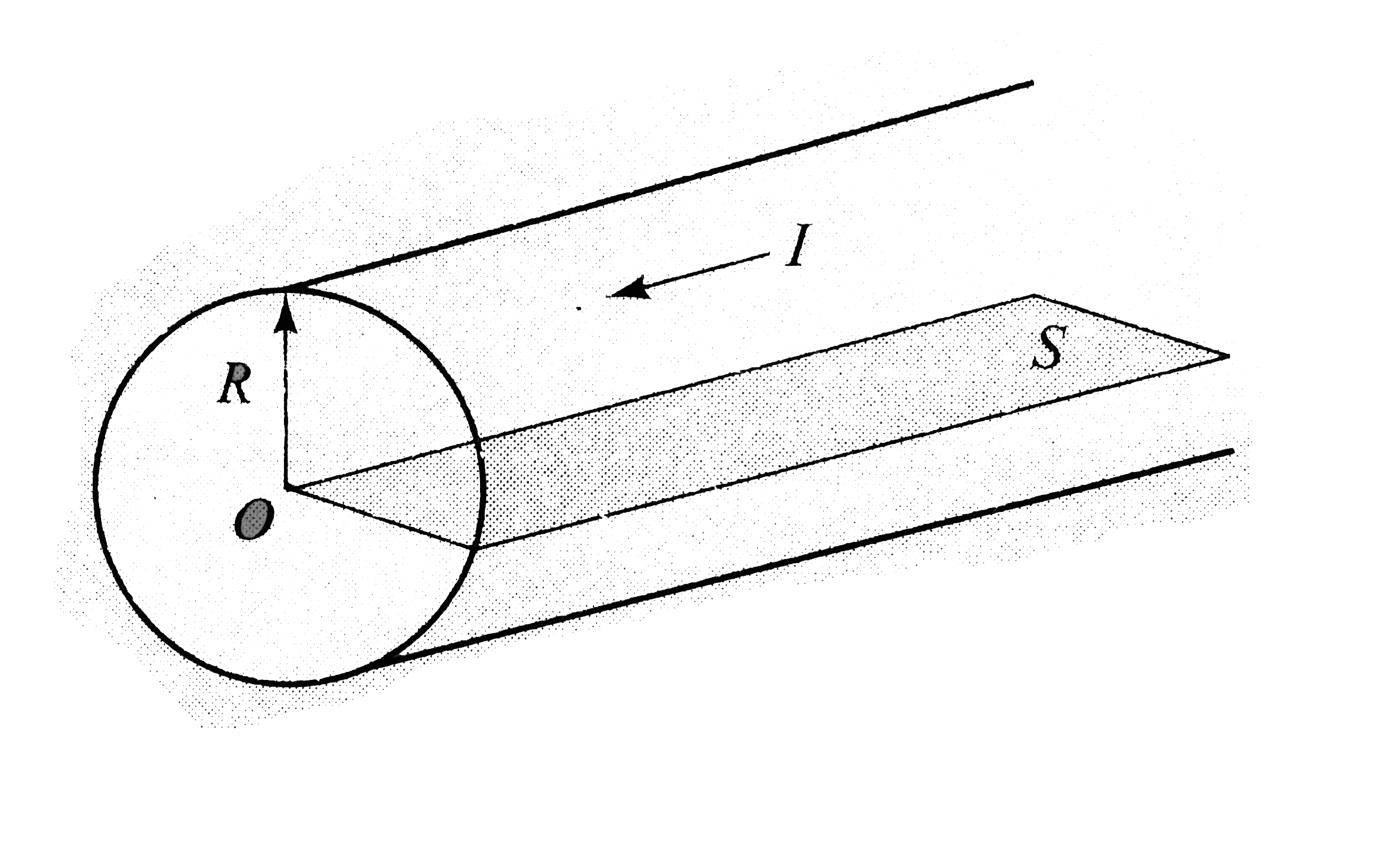 View Solution
View SolutionA conducting wire carrying current I is shown in the figure. The magnitude of magnetic field at the point P will be
 View Solution
View SolutionThere is a copper wire of radius 1mm and it carries a current of 2 ampere, calculate the drift speed of electrons assuming there are 8.5×1028 free electrons per metre cube.
View SolutionThere is a thin conducting wire carrying current. What is the value of magnetic field induction at any point on the conductor itself?
View SolutionA long, straight wire carries a current. Is Ampere's law
valid for a loop that does not enclose the wire, or that
encloses the wire but is not circular?View Solution

