दिखाइए गए परिपथ में, R1=1.0Ω,R2=2.0Ω,E1=2V और E2=E3=4V है। बिंदुओं a एवं b के बिच विभवांतर लगभग (वोल्ट में) है
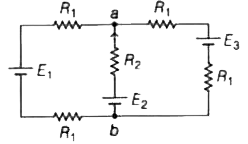
2.3
3.3
2.7
3.7
The correct Answer is:B
Step by step video & image solution for दिखाइए गए परिपथ में, R_(1)=1.0Omega,R_(2)=2.0Omega,E_(1)=2V और E_(2)=E_(3)=4V है। बिंदुओं a एवं b के बिच विभवांतर लगभग (वोल्ट में) है by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- सॉल्वड पेपर (JEE MAIN 2019)BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - सॉल्वड पेपर (JEE MAIN 2019)EXERCISE - 8 April, Shift-II
30 Videos
- सॉल्वड पेपर (JEE MAIN 2019)BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - सॉल्वड पेपर (JEE MAIN 2019)EXERCISE - 9 April, Shift-I
30 Videos
- सॉल्वड पेपर (JEE MAIN 2019)BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - सॉल्वड पेपर (JEE MAIN 2019)EXERCISE - 12 Jan, Shift-II (JANUARY ATTEMPT)
30 Videos
- सामान्य भौतिकी BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - सामान्य भौतिकी EXERCISE - विश्लेषणात्मक प्रश्न
9 Videos
- सॉल्वड पेपर (JEE MAIN 2021)BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - सॉल्वड पेपर (JEE MAIN 2021)EXERCISE - Physics (Section B)
10 Videos
Similar Questions
.40Ca तथा .16O के नाभिको के द्रव्यमान घनत्व के अनुपात का सन्निकट मान होगा
View Solution5μF धारिता के एक संधारित्र को 5μC तक आवेशित किया जाता है। यदि संधारित्र की प्लेटो को दूर हटाकर उसकी धारिता 2μF कर दी जाए, तो किया गया कार्य होगा
View Solutionएक m द्रव्यमान का अल्फ़ा कण किसी अज्ञात द्रव्यमान के स्थिर नाभिक से एक-विमीय प्रत्यास्थ संघट्ट करके अपनी प्रारम्भिक गतिज ऊर्जा का 64% भाग क्षय करके ठीक विपरीत दिशा में प्रकीर्णित हो जाता है। नाभिक का द्रव्यमान होगा
View Solutionनीचे दी गई राशियों के MKS पद्धति में मात्रक बताइए।
(i) यंग प्रत्यास्थता गुणांक
(ii) चुम्बकीय प्रेरण
(iii) लेन्सों की क्षमता
View SolutionN2 गैस को औसत रेखीय गतिज ऊर्जा ___∘ सेल्सियस ताप पर विरामावस्था से 0.1 Volt विभवान्तर से त्वरित इलेक्ट्रान के गतिज ऊर्जा के बराबर होती है। (दिया है kB=1.38×10−23J/K) (नकटतम् पूर्णांक तक भरिये)
View SolutionIn the network shown the potential difference between A and B is (R = r_(1) = r_(2) = r_(3) = 1 Omega, E_(1) = 3V, E_(2) = 2V, E_(3) = 1V )
View SolutionIn the circuit shown here, E_(1) = E_(2) = E_(3) = 2 V and R_(1) = R_(2) = 4 ohms . The current flowing between point A and B through battery E_(2) is
View SolutionA double-layer cylindrical capacitor consists of conductors of radii R_(1) and R_(3) and two layers of dielectrics of relative permittivtties epsilon_(1) and espion_(2) . The radius of the common surface of the dielectrics. Is R_(2) . The breakdown field strengths of the dielectrics are E_(1) and E_(2) respectively. What is the breakdown voltage of this capacitor if (a) epsilon_(1)R_(1)E_(1) lt epsilon_(2)R_(2)E_(2) (b) epsilon_(2)R_(2)E_(2)gtepsilon_(1)E_(1)R_(1) ? [Hint: If E_(1)=E_(1max) and E_(2)gt ,E_(2max) dielectric 1 will break first and if E_(2)=E_(2max') E_(1) gt E_(1max) dielectric 2 will break first.]
View SolutionIn the circuit as shown in figure, E_(1)=2V , E_(2)=2V, E_(3)=1V, and R=r_(1)=r_(2)=r_(3)Omega . The potential difference between points A and B will be
View SolutionIn the following circuit E_(1)=E_(2)=E_(3) 2V and R_(1)=R_(2)=AOmega . The current passing through battery E_(2) between points A and B is
View SolutionIn the network shown the potential difference between A and B is R(=r_(1)=r_(2)=r_(3)=1Omega,E_(1)=3V,E_(2)=2V,E_(3)=1V)
View Solutionबेज प्रमेय के अनुसारE1,E2 प्रतिदर्श समष्टि के विभाजन का निर्माण करती है अर्थात E1,E2 असंयुक्त है और E1∩E2=S ,A एक शून्येतर प्रायिकता कि घटना है , तब P(E1A)=.......... और P(E2A)=..........
View SolutionIf E_(1) , E_(2),E_(3) ... E_(n) represent emfs of a number of cells of internal resistances r_(1) , r_(2) , ... r_(n) then mention their equivalent emf and internal resistance when these are connected in series.
View Solutionदिखाए गए परिपथ में, R1=1.0Ω,R2=2.0Ω,E1=2V और E2=E3=4V है। बिन्दुओं a एवं b के बीच विभवान्तर लगभग (वोल्ट में) है
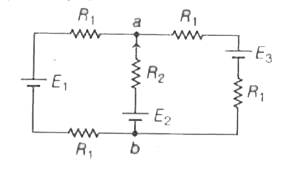
View SolutionFor the circuit shown, with R1=1.0Ω,R2=2.0Ω,E1=2V and E2=E3=4V, the potential difference between the points 'a' and 'b' is approximately (in V):
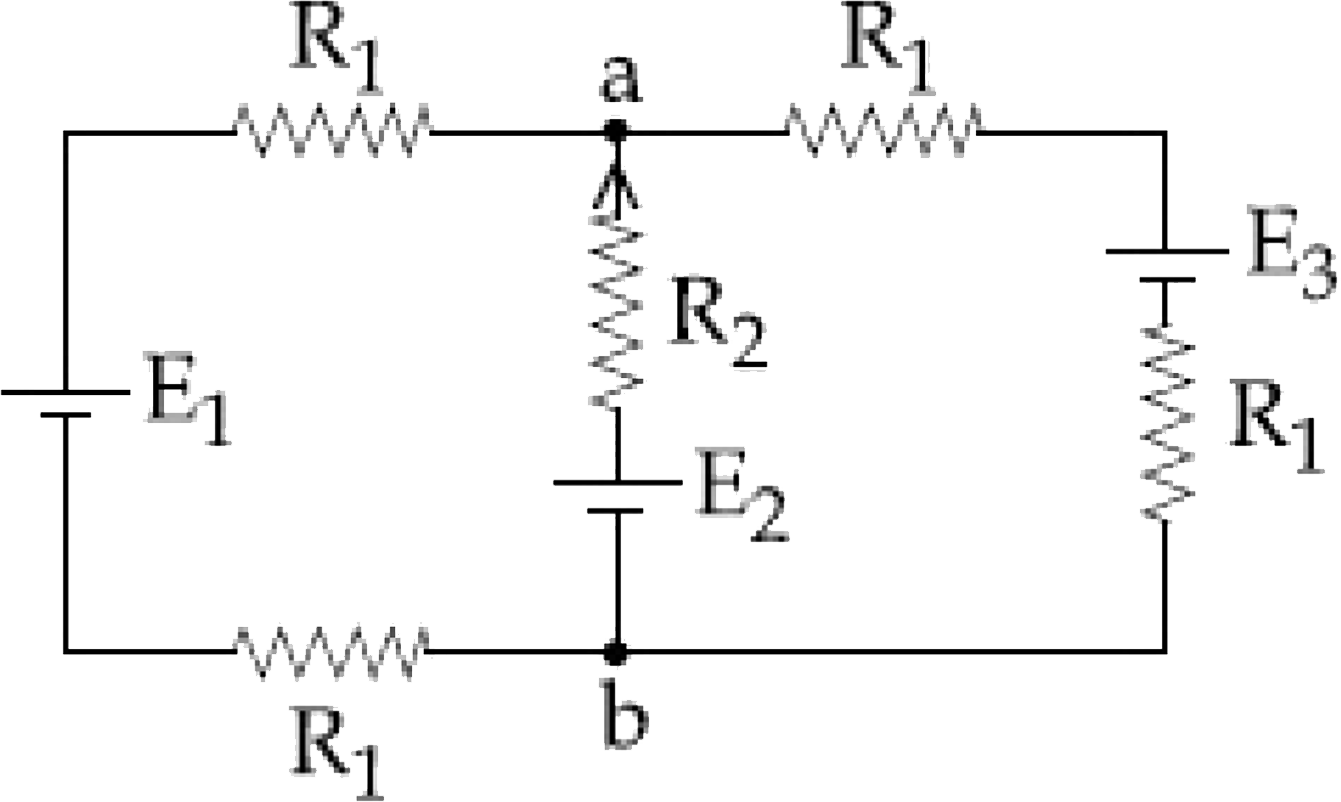
View Solution
