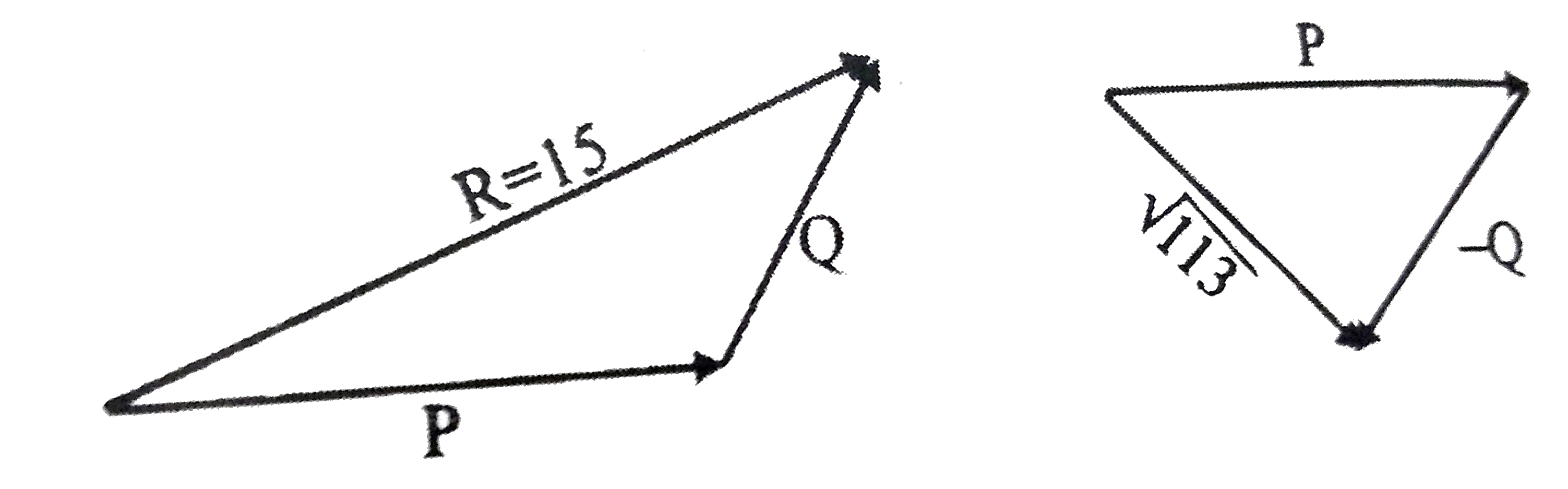
Two vectors $\to P and \to Q$ are added, the magnitude of resultant is 15 units. If $\to Q$ is reversed and added to $\to P$ resultant has a magnitude $\sqrt{113}$ units. The resultant of $\to P$ and a vector perpendicular $\to P$ and equal in magnitude to $\to Q$ has a magnitude

A

13 units

B

17 units

C

19 units

D

20 units

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

$P^{2} + Q^{2} + 2 P Q cos θ = 225$ …(i)
$P^{2} + Q^{2} - 2 P Q cos θ = 113$ …..(ii)
By adding (i) & (ii) $2 (P^{2} + Q^{2}) = 338$
$P^{2} + Q^{2} = 169 \Rightarrow \sqrt{P^{2} + Q^{2}} = 13$

|

Updated on:21/07/2023

Class 12 PHYSICS UNIT & DIMENSIONS, BASIC MATHS AND VECTOR

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The resultant $\to P and \to Q$ is perpendicular to $\to P$ . What is the angle between $\to P and \to Q$ ?
A ${cos}^{- 1} (P / Q)$
B ${cos}^{- 1} (- P / Q)$
C ${sin}^{- 1} (P / Q)$
D ${sin}^{- 1} (- P / Q)$
Question 2 - Select One
The resultant of two vectors $\to P$ and $\to Q$ is $\to R$ . If the magnitude of $\to Q$ is doubled, the new resultant vector becomes perpendicular to $\to P$ . Then, the magnitude of $\to R$ is equal to
A $P + Q$
BP
C $P - Q$
DQ
Question 3 - Select One
Two vector $\to A$ and $\to B$ have equal magnitudes. Then the vector $\to A + \to B$ is perpendicular :
A $\to A \times \to B$
B $\to A - \to B$
C $3 \to A - 3 \to B$
DAll of these

ALLEN-UNIT & DIMENSIONS, BASIC MATHS AND VECTOR -Solved Examples

Home
Ask Doubt
Profile