The time period (T) of a spring mass system depends upon mass (m) & spring constant (k) & length of the spring (l)[k=Forcelength]. Find the relation among T, m , l & k using dimensional method.
The correct Answer is:T=a√mk
To find the relation among the time period T, mass m, spring constant k, and length of the spring l using the dimensional method, we will follow these steps:
Step 1: Identify the dimensions of each variable
- The dimension of time period T is (T).
- The dimension of mass m is (M).
- The spring constant k is defined as k=Fl, where F is force and l is length. The dimension of force F is (M)(L)(T−2) and the dimension of length l is (L). Therefore, the dimension of spring constant k is:
(k)=(F)(l)=(M)(L)(T−2)(L)=(M)(T−2)
- The dimension of length l is (L).
Step 2: Assume a relationship
We assume that the time period T can be expressed as a function of m, k, and l:
T=C⋅mp⋅kq⋅lr
where C is a dimensionless constant, and p, q, and r are the powers we need to determine.
Step 3: Write the dimensions of the equation
Substituting the dimensions into the equation gives:
(T)=(M)p⋅(M)q⋅(T)−2q⋅(L)r
This can be expressed as:
(T)=(M)p+q⋅(L)r⋅(T)−2q
Step 4: Equate dimensions
Now we equate the dimensions on both sides:
- For the dimension of mass M:
0=p+q(1)
- For the dimension of length L:
0=r(2)
- For the dimension of time T:
1=−2q(3)
Step 5: Solve the equations
From equation (2), we have:
r=0
From equation (3):
q=−12
Substituting q into equation (1):
0=p−12⟹p=12
Step 6: Write the final relation
Substituting the values of p, q, and r back into the assumed relationship gives:
T=C⋅m12⋅k−12⋅l0
Thus, we can simplify this to:
T=C⋅√mk
Final Relation
The final relation among T, m, l, and k is:
T∝√mk
- The dimension of time period T is (T).
- The dimension of mass m is (M).
- The spring constant k is defined as k=Fl, where F is force and l is length. The dimension of force F is (M)(L)(T−2) and the dimension of length l is (L). Therefore, the dimension of spring constant k is:
(k)=(F)(l)=(M)(L)(T−2)(L)=(M)(T−2)
- The dimension of length l is (L).
We assume that the time period T can be expressed as a function of m, k, and l:
T=C⋅mp⋅kq⋅lr
where C is a dimensionless constant, and p, q, and r are the powers we need to determine.
Substituting the dimensions into the equation gives:
(T)=(M)p⋅(M)q⋅(T)−2q⋅(L)r
This can be expressed as:
(T)=(M)p+q⋅(L)r⋅(T)−2q
Now we equate the dimensions on both sides:
- For the dimension of mass M:
0=p+q(1)
- For the dimension of length L:
0=r(2)
- For the dimension of time T:
1=−2q(3)
From equation (2), we have:
r=0
q=−12
0=p−12⟹p=12
Substituting the values of p, q, and r back into the assumed relationship gives:
T=C⋅m12⋅k−12⋅l0
Thus, we can simplify this to:
T=C⋅√mk
The final relation among T, m, l, and k is:
T∝√mk
Topper's Solved these Questions
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (S-2)
7 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-1) Single Correct Type Questions.
37 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Solved Examples
7 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - MATHS
18 Videos
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 2 (Previous Year Questions)
7 Videos
Similar Questions
Knowledge Check
I. Time period of a spring-mass system depends on its.
II, Time period of a spring-mass system depends on its mass.
III. Time period of a spring-mass system depends on ‘ spring constant Choose the correct option regarding the above statementsAI and IIBI and IIICII and IIIDAll of the aboveFind the time period of oscillation of block of mass m. Spring, and pulley are ideal. Spring constant is k.
 A2π√mkBπ√mkC4π√mkD2π√m2k
A2π√mkBπ√mkC4π√mkD2π√m2kIf the system is suspended by the mass m the length of the spring is l1. If it is inverted and hung by mass M, the length of the spring is l2. Find the natural length of the spring.
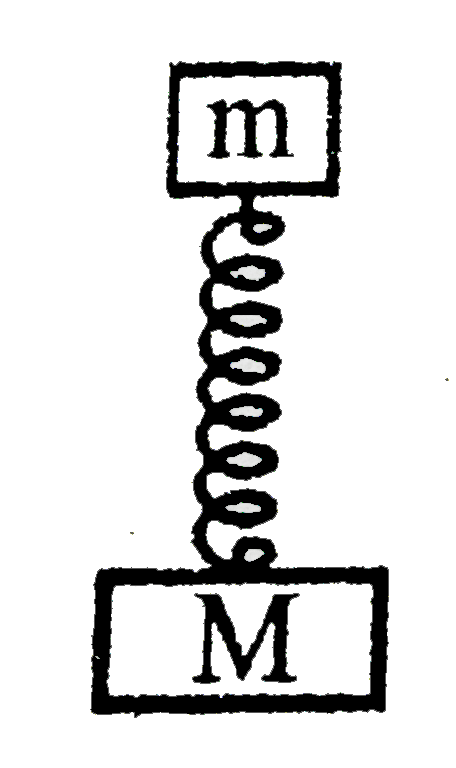 Aml1+Ml2m+MBml2+Ml1m+MCml1−Ml2m−MDml2−Ml1m−M
Aml1+Ml2m+MBml2+Ml1m+MCml1−Ml2m−MDml2−Ml1m−M

The period of oscillation of a mass M suspended from a spring of spring constant K is T. the time period of oscillation of combined blocks is
View SolutionThe periodic time of a mass suspended by a spring (force constant K) is T. If the spring is cut in three equal pieces, what will be the force constant of each part? If the same mass be suspended from one piece, what will be the periodic time?
View SolutionThe periodic time of a mass suspended by a spring (force constant k) is T. if the spring is cut in three equal pieces, what will be the force constant of each part? If the same mass be suspended from one piece, what will be the periodic time?
View SolutionThe period of oscillation of a mass M, hanging from a spring of force constant k is T. When additional mass m is attached to the spring, the period of oscillation becomes 5T/4. m/M =
View SolutionTwo identical blocks A and B , each of mass m resting on smooth floor are connected by a light spring of natural length L and spring constant k, with the spring at its natural length. A third identical block C (mass m) moving with a speed v along the line joining A and B collides with A. The maximum compression in the spring is
View Solution