The solution set of |sinx|<12 is

Video Solution
Doubtnut Promotions Banner
|
Answer

Step by step video & image solution for The solution set of |sinx| < 1/2 is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023
Class 12MATHSDEFAULT

Similar Questions

Knowledge Check

  • Recall that sinx+cosx=u (say)
    and sinxcosx=v (say) are connected by
    (sinx+cosx)2=sin2x+cos2x+2sincosx
    ⇒u2=1+2v
    ⇒v=u2−12
    It follows that any rational integral function of sinx+cosx, and sinxcosx i.e., R(sinx+cosx,sinxcosx), or in our notation R(u,v) can be transformed to R(u,u2−12). Thus, to solve an equation of the form R(u,v)=0, we form a polynomial equation in u and than look for solutions.
    The solution set of
    sinx+cosx−2√2sinxcosx=0 is completely described by

    Ax=2nπ+π4,2nπ−5π12,2nπ+11π12,n∫Z
    Bx=2nπ−π4,2nπ+π12,2nπ+7π12,n∫Z
    Cx=2nπ+π4,2nπ−π12,2nπ−7π12,n∫Z
    Dx=2nπ−π4,2nπ+π12,2nπ−7π12,n∫Z

  • The solution set of inequality
    (ex−1)(2x−3)(x2+x+2)(sinx−2)(x+1)x≤0

    A[32,∞)
    B(−∞,−1)∪[32,∞)
    C(−1,0)∪[32,∞)
    DR−{0,−1}

  • If 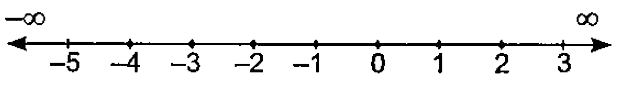ocr_image is the solution set of a linear in equation in x, then the solution set of x in set-builder form is:

    A{x:−4≤x≤2,x∈R}
    B{x:−5<x<3,x∈R}
    C{x:−4≤x≤2,x∈N}
    D{x:−5<x<3,x∈I}