Name the gas law which relates volume and pressure of gas at constant temperature.
Step-by-Step Solution:
1. Identify the Relationship: We need to identify the gas law that relates the volume and pressure of a gas while keeping the temperature constant.
2. Recall the Gas Laws: Among the various gas laws, we should recall the specific laws that deal with pressure and volume. The most relevant law here is Boyle's Law.
3. State Boyle's Law: Boyle's Law states that at constant temperature, the pressure (P) of a gas is inversely proportional to its volume (V). This can be mathematically expressed as:
P∝1V
or
PV=K
where K is a constant.
4. Understanding the Inverse Relationship: This means that if the volume of the gas increases, the pressure decreases, and vice versa, as long as the temperature remains constant.
5. Formulate the Equation: From Boyle's Law, we can derive the equation:
P1V1=P2V2
This equation shows that the product of the initial pressure and volume is equal to the product of the final pressure and volume.
6. Conclusion: Therefore, the gas law that relates the volume and pressure of a gas at constant temperature is Boyle's Law.
Final Answer: Boyle's Law
---
P∝1V
or
PV=K
where K is a constant.
P1V1=P2V2
This equation shows that the product of the initial pressure and volume is equal to the product of the final pressure and volume.
Topper's Solved these Questions
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STATES OF MATTER : GASES, LIQUIDS AND SOLIDSEXERCISE - 1-MARK QUESTIONS15videos
STATES OF MATTER : GASES, LIQUIDS AND SOLIDS
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STATES OF MATTER : GASES, LIQUIDS AND SOLIDSEXERCISE - 2-MARK QUESTIONS21videos
STATES OF MATTER : GASES, LIQUIDS AND SOLIDS
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STATES OF MATTER : GASES, LIQUIDS AND SOLIDSEXERCISE - ASSERTION AND REASON TYPE QUESTIONS5videos
STATES OF MATTER : GASES, LIQUIDS AND SOLIDS
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - SOME BASIC CONCEPTS OF CHEMISTRY EXERCISE - UNIT TEST12videos
SOME BASIC CONCEPTS OF CHEMISTRY
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STRUCTURE OF ATOMEXERCISE - UNIT TEST8videos
STRUCTURE OF ATOM
Similar Questions
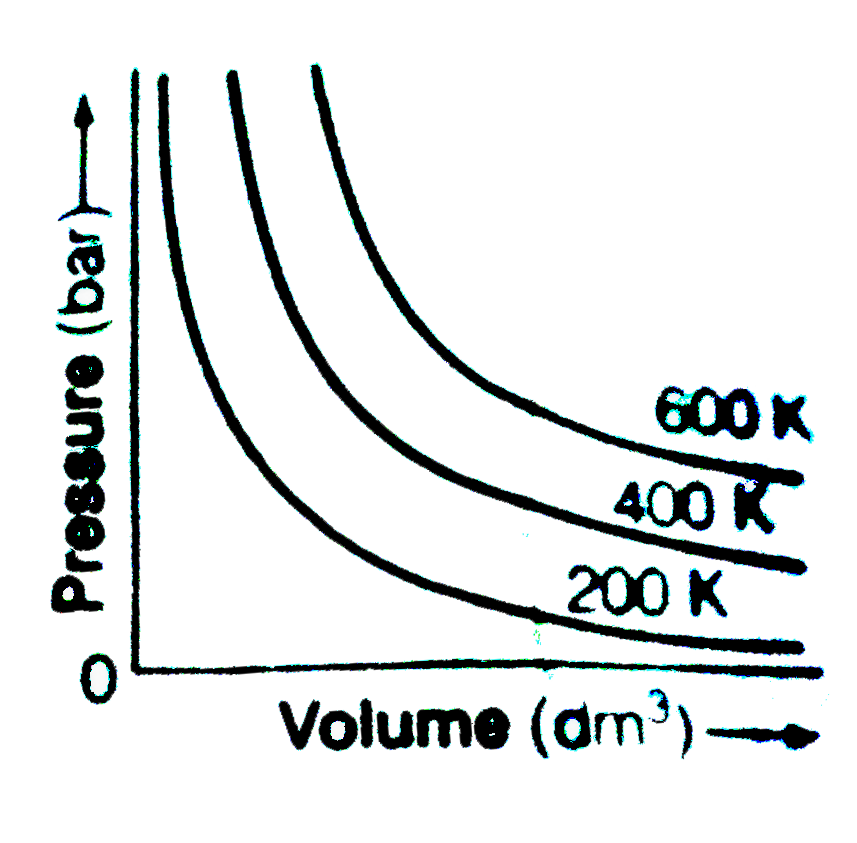
The variation of pressure with volume of the gas at different temperatures can be graphically represented as shown in figure. On the basis of this graph answer the following question.
(i) How will the volume of a gas change if its pressure is increased at constant temperature ?
(ii) At a constant pressure, how will the volume of a gas change if the temperature is increased from 200 K to 400 K ?
 View Solution
View SolutionThe percentage change in the pressure of a gas at constant temperature is 200% (increases). Calcualte the percentage change in the volume of the gas.
View Solution
Knowledge Check
- Question 1 - Select One
Which is the correct expression that relates changes of entropy with the change of pressure for an ideal gas at constant temperature, among the following?
AΔS=nRTlnP2BΔS=T(P2−P1)CΔS=nRlnP1P2DΔS=2.303nRlnP1P2 - Question 2 - Select One
Which is the correct expression that relates changes of entropy with the change of pressure for an ideal gas at constant temperature, among the following?
AΔS=nRTln.P2P1BΔS=T(P2−P1)CΔS=nRln.P1P2DΔS=2.303nRTln.P1P2 - Question 3 - Select One
Which is the correct expression that relates changes of entropy with the change of pressure for an ideal gas at constant temperature, among the following?
AΔS=nRT ln P2P1BΔS=T(P2−P1)CΔS=nR ln (P1P2)DΔS=2.303nRT ln (P1P2)
The percentage change in the pressure of gas at constant temperature is 200% (increases). Calculate the percentage change in the volume of the gas.
View SolutionChoose the correct option for graphical representation of Boyle's Law, which shows a graph of pressure vs volume of gas at different temperatures:
View SolutionAssertion : The pressure of a gas is inversely proportional to its volume at constant temperature and n.
Reason : The gas volume is directly proportional to n at constant temperature and pressure .View SolutionAssertion : The pressure of a gas is inversely proportional to the volume at contant temperature and n
Reason : The gas volume is directly proportional to n at constant temperature and pressureView SolutionWhich of the following is not the correct set of pressure and volume at constant temperature and constant moles of gas?
View Solution

