A 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. The position of image and the magnification respectively are
3.33,57
6.7cm,1.8
0.15 cm, 1.8
6.7cm,59
The correct Answer is:D
To solve the problem, we need to find the position of the image and the magnification when a needle of height 4.5 cm is placed 12 cm away from a convex mirror with a focal length of 15 cm.
1. Identify the given values:
- Height of the needle (object height, h) = 4.5 cm
- Object distance (u) = -12 cm (the object distance is taken as negative in mirror formula convention)
- Focal length of the convex mirror (f) = +15 cm (focal length is positive for convex mirrors)
2. Use the mirror formula:
The mirror formula is given by:
1f=1v+1u
Substituting the known values into the formula:
115=1v+1−12
3. Rearranging the equation:
1v=115+112
To add these fractions, find a common denominator. The least common multiple of 15 and 12 is 60.
115=460,112=560
Therefore,
1v=460+560=960
4. Calculate v:
Taking the reciprocal gives:
v=609=203≈6.67 cm
5. Calculate the magnification (m):
The magnification formula for mirrors is given by:
m=−vu
Substituting the values:
m=−203−12=203×12=2036=59
Final Results:
- Position of the image (v) = 6.67 cm (approximately)
- Magnification (m) = 59
- Height of the needle (object height, h) = 4.5 cm
- Object distance (u) = -12 cm (the object distance is taken as negative in mirror formula convention)
- Focal length of the convex mirror (f) = +15 cm (focal length is positive for convex mirrors)
The mirror formula is given by:
1f=1v+1u
Substituting the known values into the formula:
115=1v+1−12
1v=115+112
To add these fractions, find a common denominator. The least common multiple of 15 and 12 is 60.
115=460,112=560
Therefore,
1v=460+560=960
Taking the reciprocal gives:
v=609=203≈6.67 cm
The magnification formula for mirrors is given by:
m=−vu
Substituting the values:
m=−203−12=203×12=2036=59
- Position of the image (v) = 6.67 cm (approximately)
- Magnification (m) = 59
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRAUMENTS BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE - 1 (C.W)(REFRACTION)
10 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE - 1 (C.W)(REFRACTION THROUGH SPHERICAL SURFACES)
1 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - CUQ (ASSERTION & REASON)
16 Videos
- NUCLEI BOOK - NARAYNACHAPTER - NUCLEI EXERCISE - ASSERTION & REASON
5 Videos
- SEMI CONDUCTOR DEVICESBOOK - NARAYNACHAPTER - SEMI CONDUCTOR DEVICESEXERCISE - Level-II (H.W)
36 Videos
Similar Questions
A 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. Give the location of the image and the magnification. Describe what happens as the needle is moved farther from the mirror.
View SolutionA 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. Give the location of the image and the magnification. Describe what happens as the needle is moved farther from the mirror?
View Solution
Knowledge Check
A 4.5cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. Give the location of the image and the magnification. Describe what happens as the needle is moved farther from the mirror.
A6 cm , 0.718B6.7 cm , 0.558CCannot be determinedDNONE OF THESEA 4.5cm needle is placed 12cm away from a convex mirror of focal length 15cm. Given the location of the image and the magniftication.
A6.7cm,5/9B7.5cm,5/9C6.7cm,9/5D7.5cm,9/5An object is placed at 20 cm from a convex mirror of focal length 10 cm. The image formed by the mirror is
AReal and at 20cm from the mirrorBVirtual and at 20 cm from the mirrorCVirtual and at 20/3cm from the mirrorDReal and at 20/3 cm from the mirror
A 2.5 cm candle is placed 12 cm away from a convex mirror of focal length 30 cm. Give the location of the image and the magnification.
View SolutionA 0.2 cm high object is placed 15 cm from a concave mirror of focal length 5 cm. Find position and size of the image.
View SolutionA 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm
With reference to the above situation, match the items in Column I with Column II and choose the correct option from the codes below.
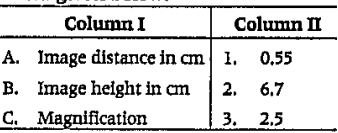
View SolutionA 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm.
With reference to the above situation, match the items in Column I with Column II and choose the correct option from the codes given below.
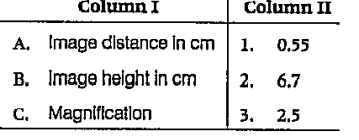
View SolutionIf an object is 30 cm away from a concave mirror of focal length 15 cm the image will be
View Solution
