A mark is made on the surface of a glass sphere of diameter 10 cm and refractive index 1.5. It is viewed through the glass from a portion directly opposite. The distance of the image of the mark from the centre of the sphere will be
15 cm
17.5 cm
20 cm
22.5 cm
The correct Answer is:A
To solve the problem of finding the distance of the image of the mark from the center of the glass sphere, we can follow these steps:
1. Identify Given Data:
- Diameter of the glass sphere, D=10cm
- Radius of the glass sphere, R=D2=5cm
- Refractive index of glass, μ2=1.5
- Refractive index of air, μ1=1
2. Set Up the Sign Convention:
- The object (mark) is on the surface of the sphere. Since we are measuring distances from the center of the sphere and the mark is on the surface, we take the object distance u=−5cm (negative as per the sign convention for real objects).
3. Use the Lens Maker's Formula:
The formula relating the object distance u, image distance v, and the radius of curvature R is given by:
μ2v−μ1u=μ2−μ1R
Substituting the known values:
1.5v−1−5=1.5−15
4. Simplify the Equation:
1.5v+15=0.55
1.5v+15=0.11
5. Multiply through by 5v to eliminate fractions:
5⋅1.5=0.5v+v
7.5=1.5v
6. Solve for v:
v=7.51.5=5cm
7. Determine the Distance from the Center:
Since the image distance v is measured from the center of the sphere, and the mark was at the surface, the distance of the image from the center of the sphere is 5cm.
Final Answer:
The distance of the image of the mark from the center of the sphere is 5cm.
---
- Diameter of the glass sphere, D=10cm
- Radius of the glass sphere, R=D2=5cm
- Refractive index of glass, μ2=1.5
- Refractive index of air, μ1=1
- The object (mark) is on the surface of the sphere. Since we are measuring distances from the center of the sphere and the mark is on the surface, we take the object distance u=−5cm (negative as per the sign convention for real objects).
The formula relating the object distance u, image distance v, and the radius of curvature R is given by:
μ2v−μ1u=μ2−μ1R
Substituting the known values:
1.5v−1−5=1.5−15
1.5v+15=0.55
1.5v+15=0.11
5⋅1.5=0.5v+v
7.5=1.5v
v=7.51.5=5cm
Since the image distance v is measured from the center of the sphere, and the mark was at the surface, the distance of the image from the center of the sphere is 5cm.
The distance of the image of the mark from the center of the sphere is 5cm.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE-2 (H.W)(LENSES & THEIR COMBINATIONS)23videos
RAY OPTICS AND OPTICAL INSTRAUMENTS
- BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE-2 (H.W)(REFRACTION THROUGH A PRISM)9videos
RAY OPTICS AND OPTICAL INSTRAUMENTS
- BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE-2 (H.W)(REFRACTION)21videos
RAY OPTICS AND OPTICAL INSTRAUMENTS
- BOOK - NARAYNACHAPTER - NUCLEI EXERCISE - ASSERTION & REASON5videos
NUCLEI
- BOOK - NARAYNACHAPTER - SEMI CONDUCTOR DEVICESEXERCISE - Level-II (H.W)36videos
SEMI CONDUCTOR DEVICES
Similar Questions
If a mark of size 0.2 cm on the surface of a glass sphere of diameter 10 cm and μ=1.5is viewed through the diametrically opposite point, where will the image be seen and of what size? (in mm)
View SolutionA mark placed on the surface of glass sphere is viewed through glass from an oppositely directed position. If the diameter of the sphere is 30 cm, locate the image of the mark. For glass, μ=1.5
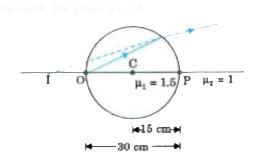 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A point object is placed at the centre of a glass sphere of radius 6 cm and refractive index 1.5. The distance of the virtual image from the surface of the glass is
A2 cmB4 cmC6 cmD12 cm - Question 2 - Select One
A point object is placed at the centre of a glass sphere of radius 6cm and refractive index 1.5. The distance of virtual image from the surface is
A6cmB4cmC12cmD9cm - Question 3 - Select One
A point object is placed at the center of a glass sphere of radius 6cm and refractive index 1.5. The distance of virtual image from the surface is
A6cmB4cmC12cmD9cm
A hollow sphere made of a transparent material of refractive index 1.5 has a small mark on its surface as shown. The mark is observed from the farther side. Find the distance of the image of mark from the centre of the sphere.
 View Solution
View SolutionA mark placed on the surface of a sphere is viewed through glass from a position directly opposite. If the diameter of the sphere is 10cm and refractive index of glass is 1.5, find the position of the image.
View SolutionA small ink dot on a paper is viewed through a glass slab of thickness 10cm , and refractive index 1.5. By what distance would the dot appear to be raised ?
View SolutionA small ink dot on a paper is viewed through a glass slab of thickness 10cm and refractive index 1.5. By what distance would the dot appear to be raised?
View SolutionThere is a small air bubble at the centre of a solid glass sphere of radius 'r' and refractive index μ. What wil be the apparent distance of the bubble from the centre of the sphere, when viewed from outside?
View Solution

