Parallel rays are incident on a transparent sphere along its one diameter. After refraction, these rays converge at the other end of this diameter. The refractive index for the material of sphere is
1
1.5
1.6
2
The correct Answer is:D
To solve the problem of finding the refractive index of a transparent sphere when parallel rays of light are incident along one diameter and converge at the other end after refraction, we can use the lens maker's formula for a spherical surface. Here's a step-by-step solution:
1. Identify the Given Information:
- The rays are parallel and incident on the sphere along its diameter.
- After refraction, they converge at the other end of the diameter.
- The diameter of the sphere is given as D.
2. Define the Variables:
- Let μ1 be the refractive index of air (approximately 1).
- Let μ2 be the refractive index of the sphere (this is what we need to find).
- The object distance u for parallel rays is taken as −∞ (since they are coming from infinity).
- The image distance v is equal to the diameter D (the rays converge at the other end).
- The radius of curvature R of the sphere is D2.
3. Apply the Refraction Formula:
The formula for refraction at a spherical surface is given by:
μ2v−μ1u=μ2−μ1R
4. Substitute the Values:
- Substitute u=−∞, v=D, R=D2, and μ1=1:
μ2D−1−∞=μ2−1D2
Since 1−∞ approaches 0, the equation simplifies to:
μ2D=μ2−1D2
5. Cross-Multiply and Simplify:
Cross-multiplying gives us:
2μ2=D(μ2−1)
Expanding this, we have:
2μ2=Dμ2−D
Rearranging terms gives:
Dμ2−2μ2=D
Factoring out μ2:
μ2(D−2)=D
6. Solve for μ2:
Dividing both sides by D−2:
μ2=DD−2
7. Determine the Value of μ2:
Since the diameter D is a positive value, we can analyze the options given:
- If D=2, then μ2=22−2 which is undefined.
- If D>2, μ2 will yield values greater than 1.
- The options provided are 1, 1.5, 1.6, and 2.
Testing D=2 gives μ2=2 as a valid solution.
Conclusion:
The refractive index for the material of the sphere is μ2=2.
- The rays are parallel and incident on the sphere along its diameter.
- After refraction, they converge at the other end of the diameter.
- The diameter of the sphere is given as D.
- Let μ1 be the refractive index of air (approximately 1).
- Let μ2 be the refractive index of the sphere (this is what we need to find).
- The object distance u for parallel rays is taken as −∞ (since they are coming from infinity).
- The image distance v is equal to the diameter D (the rays converge at the other end).
- The radius of curvature R of the sphere is D2.
The formula for refraction at a spherical surface is given by:
μ2v−μ1u=μ2−μ1R
- Substitute u=−∞, v=D, R=D2, and μ1=1:
μ2D−1−∞=μ2−1D2
Since 1−∞ approaches 0, the equation simplifies to:
μ2D=μ2−1D2
Cross-multiplying gives us:
2μ2=D(μ2−1)
Expanding this, we have:
2μ2=Dμ2−D
Rearranging terms gives:
Dμ2−2μ2=D
Factoring out μ2:
μ2(D−2)=D
Dividing both sides by D−2:
μ2=DD−2
Since the diameter D is a positive value, we can analyze the options given:
- If D=2, then μ2=22−2 which is undefined.
- If D>2, μ2 will yield values greater than 1.
- The options provided are 1, 1.5, 1.6, and 2.
The refractive index for the material of the sphere is μ2=2.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRAUMENTS BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE-2 (H.W)(LENSES & THEIR COMBINATIONS)
23 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE-2 (H.W)(REFRACTION THROUGH A PRISM)
9 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE-2 (H.W)(REFRACTION)
21 Videos
- NUCLEI BOOK - NARAYNACHAPTER - NUCLEI EXERCISE - ASSERTION & REASON
5 Videos
- SEMI CONDUCTOR DEVICESBOOK - NARAYNACHAPTER - SEMI CONDUCTOR DEVICESEXERCISE - Level-II (H.W)
36 Videos
Similar Questions
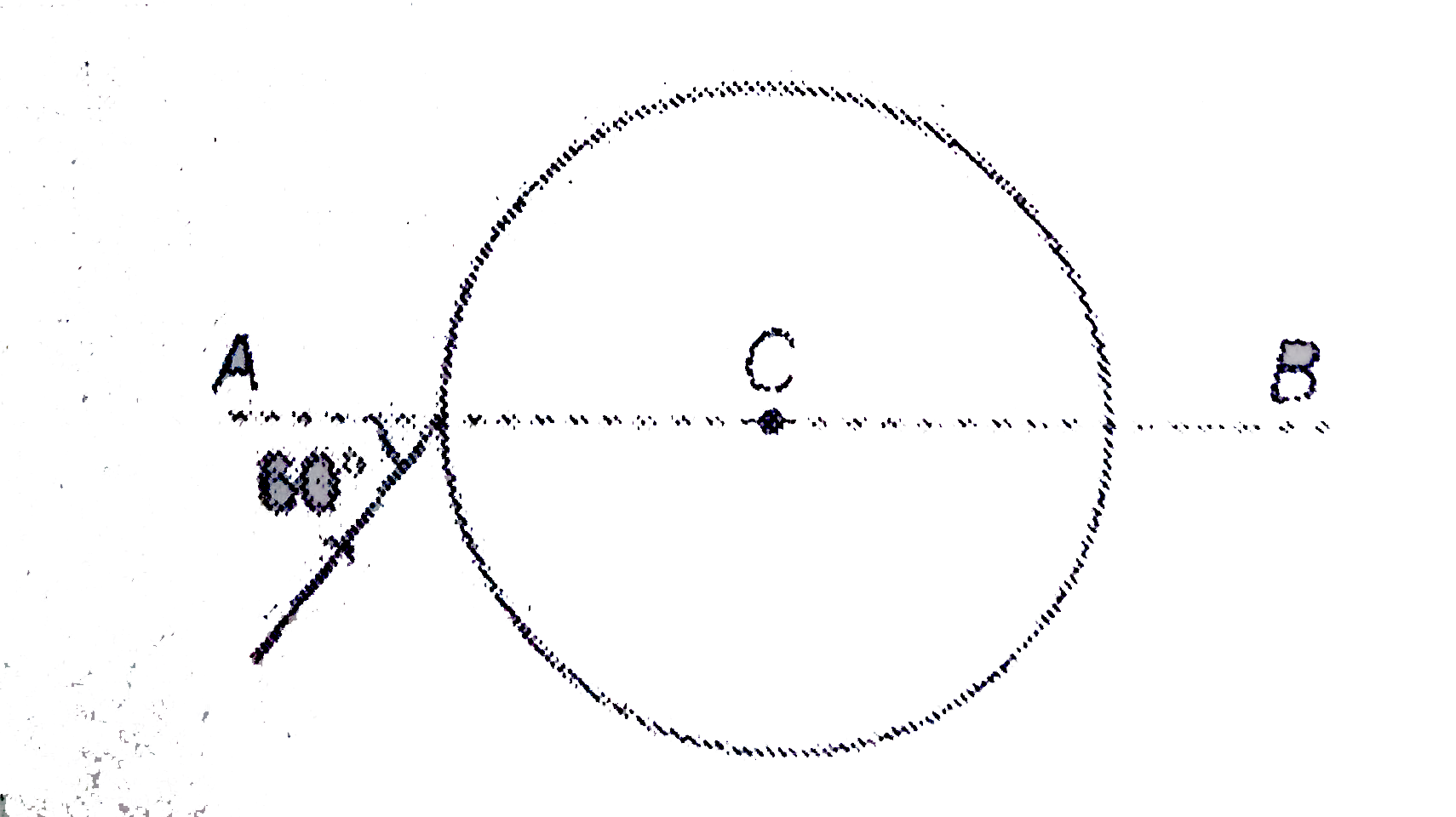
A ray of light falls on a transparent sphere with centre at C as shown in figure. The ray emerges from the sphere parallel to line AB. The refractive index of the sphere is
View SolutionA ray of light is incident normally on one of the refracting surfaces of a prism of refracting angle A,. The emergent ray grazes the other refracting surface. Find the refractive index of the material ofprism.
View Solution
Knowledge Check
A ray of light is incident normally on one of the refracting surfaces of a prism of refracting angle A,. The emergent ray grazes the other refracting surface. Find the refractive index of the material ofprism.
A1.155B1.75C1.33D1.66A point source of light is kept on the surface of a sphere and it is found that parallel light rays emerge from the other side of the sphere. What is refractive index of material of the sphere ?
A1.5B2C2.5D3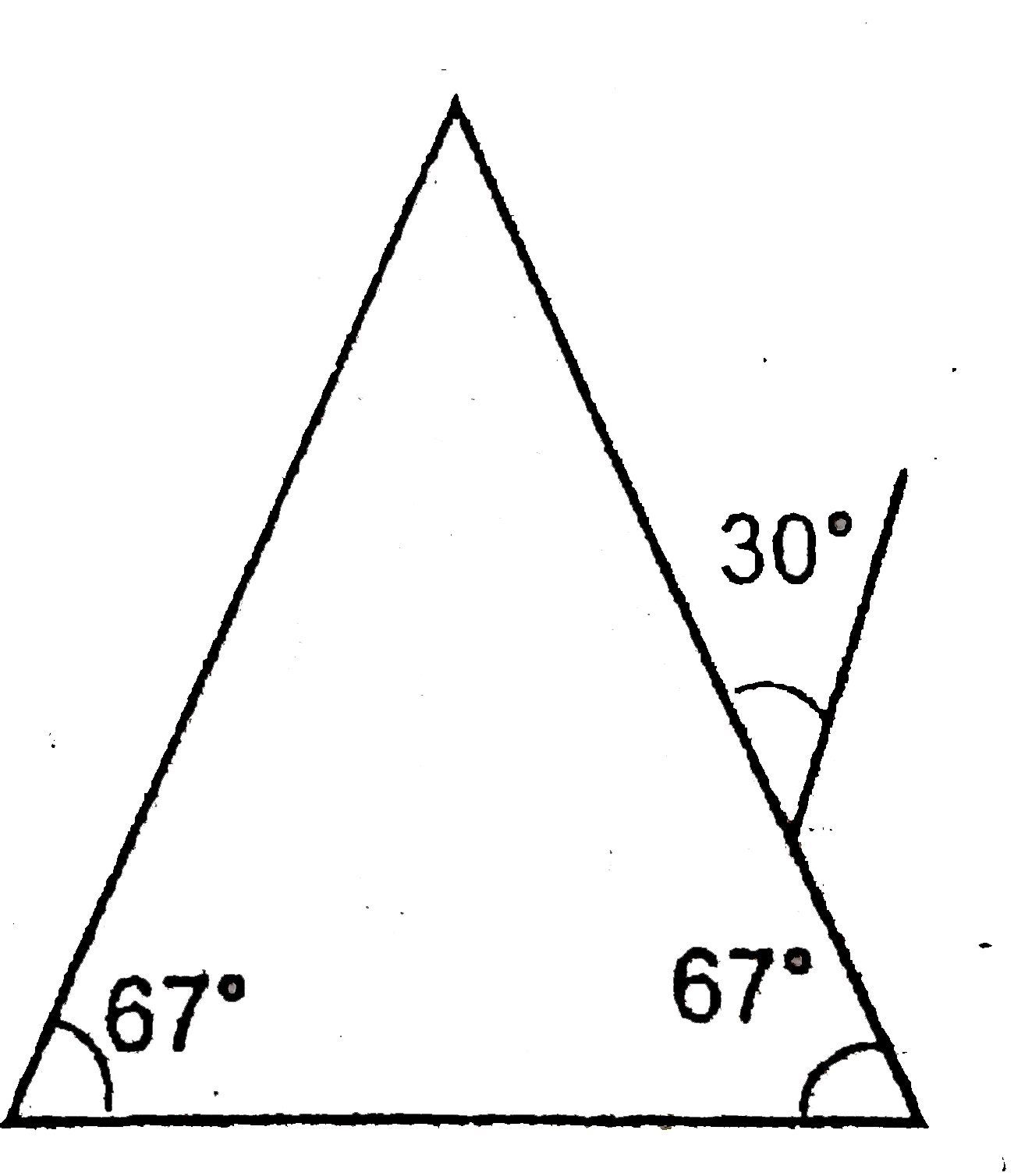
A ray of light incident on one of the face of a prism as shown. After refraction through this surface ray is incident on base of the prism. The refractive index of the prism is √3. The net deviation of the ray due to prism isA60∘ clockwiseB104∘ clockwiseC104∘ anticlockwiseD166∘ clockwise
A ray of light falls on a transparent sphere with centre at C as shown in figure. The ray emerges from the sphere parallel to line AB. The refractive index of the sphere is

View SolutionA ray of light is incident on a transparent plate of a material of refractive index √3 at the polarising angle. Find the angle of refraction.
View SolutionA monochromatic ray of light is incident normally on one of the refracting surfaces of a prism . The emergent ray grazes the second refracting surface . What is the angle of the prism if the refractive index of the material of the prism is 1.6 ?
View SolutionA monochromatic ray of light is incident normally on one of the refracting surfaces of a prism . The emergent ray grazes the second refracting surface . What is the angle of the prism if the refractive index of the material of the prism is 1.6 ?
View SolutionA monochromatic ray of light is incident normally on one of the refracting surfaces of a prism . The emergent ray grazes the second refracting surface . What is the angle of the prism if the refractive index of the material of the prism is 1.6 ?
View Solution
