A small mass m attached to one end of a spring with a negligible mass and an unstretched length L, executes vertical oscillations with angular frequency ω0. When the mass is rotated with an angular speed ω by holding the other end of the spring at a fixed point, the mass moves uniformly in a circular path in a horizontal plane. Then the increase in length of the spring during this rotation is
ω2Lω20−ω2
ω20Lω2−ω20
ω2Lω20
ω20Lω2
The correct Answer is:A
To solve the problem, we need to analyze the forces acting on the mass m when it is rotated in a circular path while attached to the spring. Here's a step-by-step solution:
Step 1: Understand the system
We have a mass m attached to a spring with an unstretched length L. The mass executes vertical oscillations with an angular frequency ω0. When the mass is rotated with an angular speed ω, it moves in a horizontal circular path.
Step 2: Define the variables
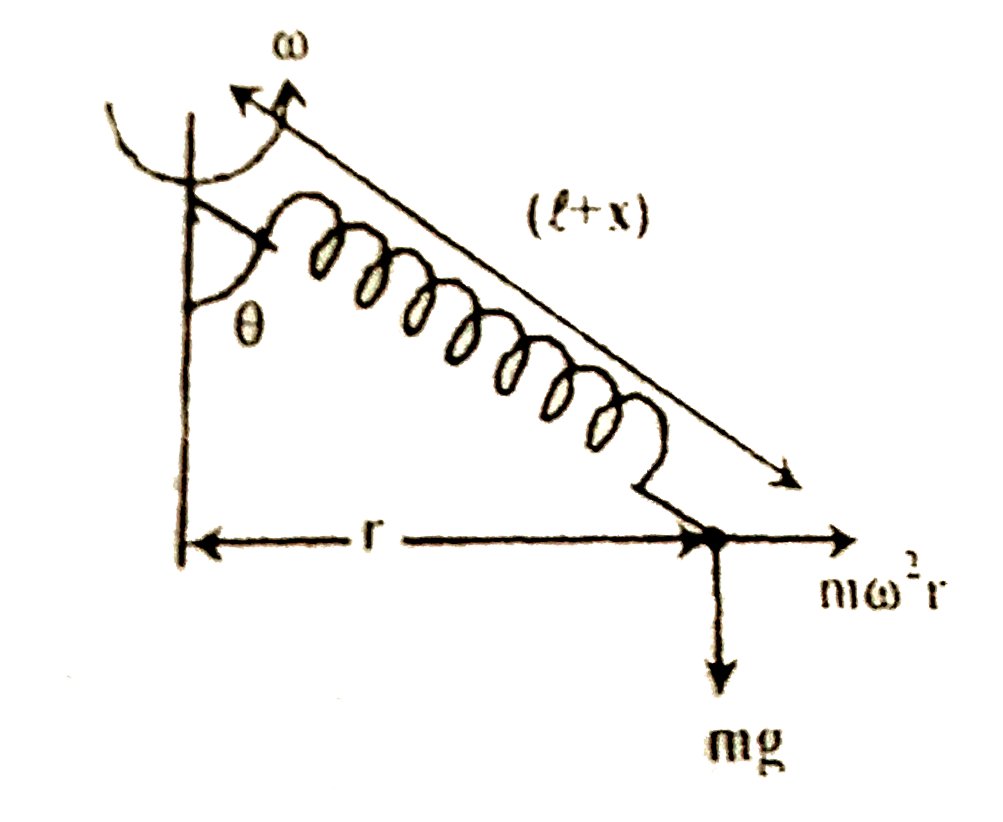
- Let x be the extension of the spring when the mass is rotated.
- The total length of the spring when extended is L+x.
- The radius r of the circular motion is given by r=(L+x)sinθ, where θ is the angle the spring makes with the vertical.
Step 3: Write the forces acting on the mass
1. The spring force acting upwards is kx (where k is the spring constant).
2. The weight of the mass acting downwards is mg.
3. The centrifugal force acting outward due to circular motion is mrω2.
Step 4: Set up the force balance
In the vertical direction, the forces must balance:
kxcosθ=mg
In the horizontal direction, the centrifugal force is balanced by the horizontal component of the spring force:
mrω2=kxsinθ
Step 5: Substitute for r
Substituting r=(L+x)sinθ into the centrifugal force equation gives:
m(L+x)sinθω2=kxsinθ
Dividing both sides by sinθ (assuming sinθ≠0):
m(L+x)ω2=kx
Step 6: Express k in terms of ω0
From the vertical oscillation, we know:
ω0=√km⟹k=mω20
Substituting this into the equation gives:
m(L+x)ω2=mω20x
Cancelling m (assuming m≠0):
(L+x)ω2=ω20x
Step 7: Rearranging the equation
Rearranging gives:
Lω2=x(ω20−ω2)
Thus, we can solve for x:
x=Lω2ω20−ω2
Conclusion
The increase in the length of the spring during rotation is:
x=Lω2ω20−ω2
We have a mass m attached to a spring with an unstretched length L. The mass executes vertical oscillations with an angular frequency ω0. When the mass is rotated with an angular speed ω, it moves in a horizontal circular path.
- Let x be the extension of the spring when the mass is rotated.
- The total length of the spring when extended is L+x.
- The radius r of the circular motion is given by r=(L+x)sinθ, where θ is the angle the spring makes with the vertical.
1. The spring force acting upwards is kx (where k is the spring constant).
2. The weight of the mass acting downwards is mg.
3. The centrifugal force acting outward due to circular motion is mrω2.
In the vertical direction, the forces must balance:
kxcosθ=mg
In the horizontal direction, the centrifugal force is balanced by the horizontal component of the spring force:
mrω2=kxsinθ
Substituting r=(L+x)sinθ into the centrifugal force equation gives:
m(L+x)sinθω2=kxsinθ
Dividing both sides by sinθ (assuming sinθ≠0):
m(L+x)ω2=kx
From the vertical oscillation, we know:
ω0=√km⟹k=mω20
Substituting this into the equation gives:
m(L+x)ω2=mω20x
Cancelling m (assuming m≠0):
(L+x)ω2=ω20x
Rearranging gives:
Lω2=x(ω20−ω2)
Thus, we can solve for x:
x=Lω2ω20−ω2
The increase in the length of the spring during rotation is:
x=Lω2ω20−ω2
Topper's Solved these Questions
Similar Questions
An underformed spring with the spring constant k has length l0. When the system (Fig.) rotates at an angular
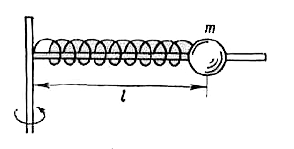
velocity ω, the weight with mass m causes an extension of the spring. Find the length l of the rotating spring.
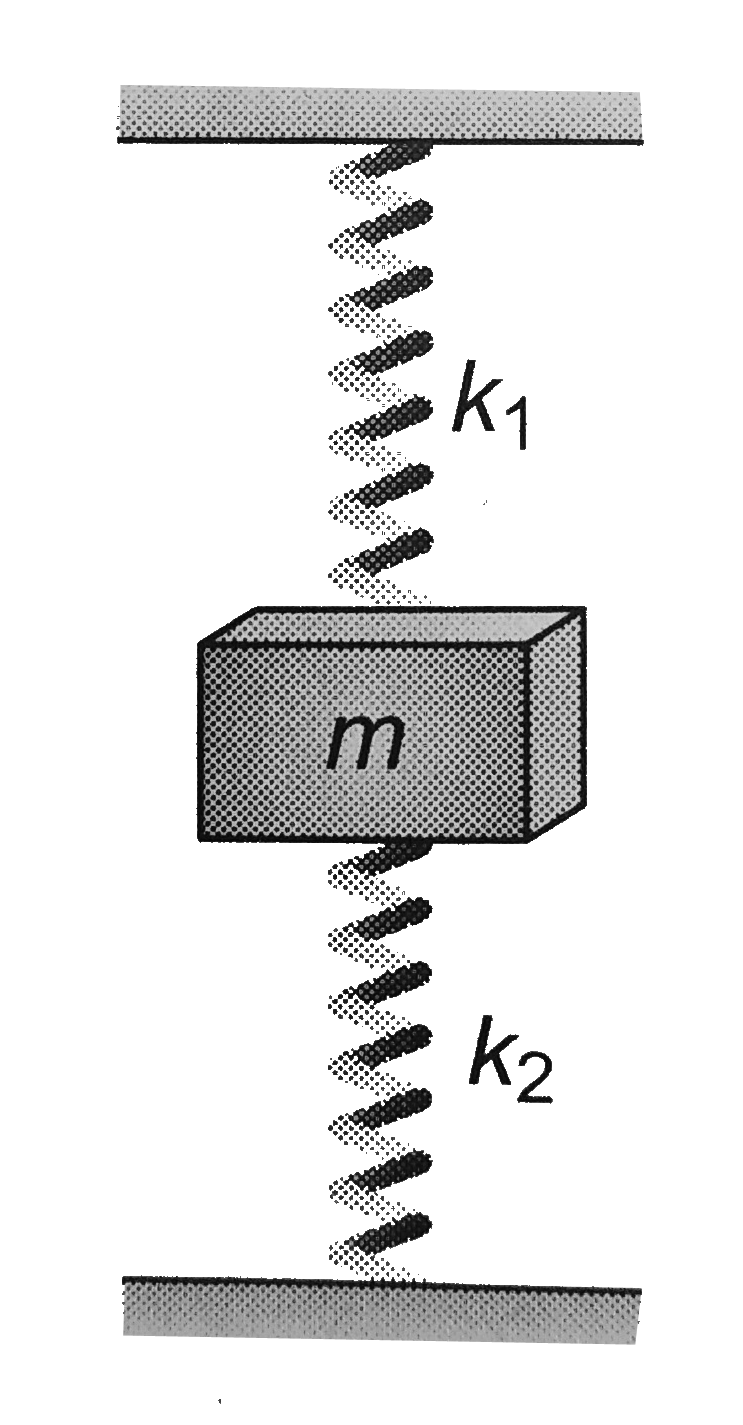
View SolutionIn the figure shown a block of masss m is atteched at ends of two spring The other ends of the spring are fixed The mass m is released in the vertical plane when the spring are released The velocity of the block is maximum when
View Solution
Knowledge Check
A particle of mass m is fixed to one end of a light spring of force constant k and unstretched length l. The other end of the spring is fixed on a vertical axis which is rotaing with an angular velocity ω. The increase in length of th espring will be
 Amω2lkBmω2lk−mω2Cmω2lk+mω2DNone of these
Amω2lkBmω2lk−mω2Cmω2lk+mω2DNone of theseA particles of mass m is fixed to one end of a light spring of force constant k and unstreatched length l. the system is rotated about the other end of the spring with an angular velocity ω in gravity free space. The increase in length of the spring is
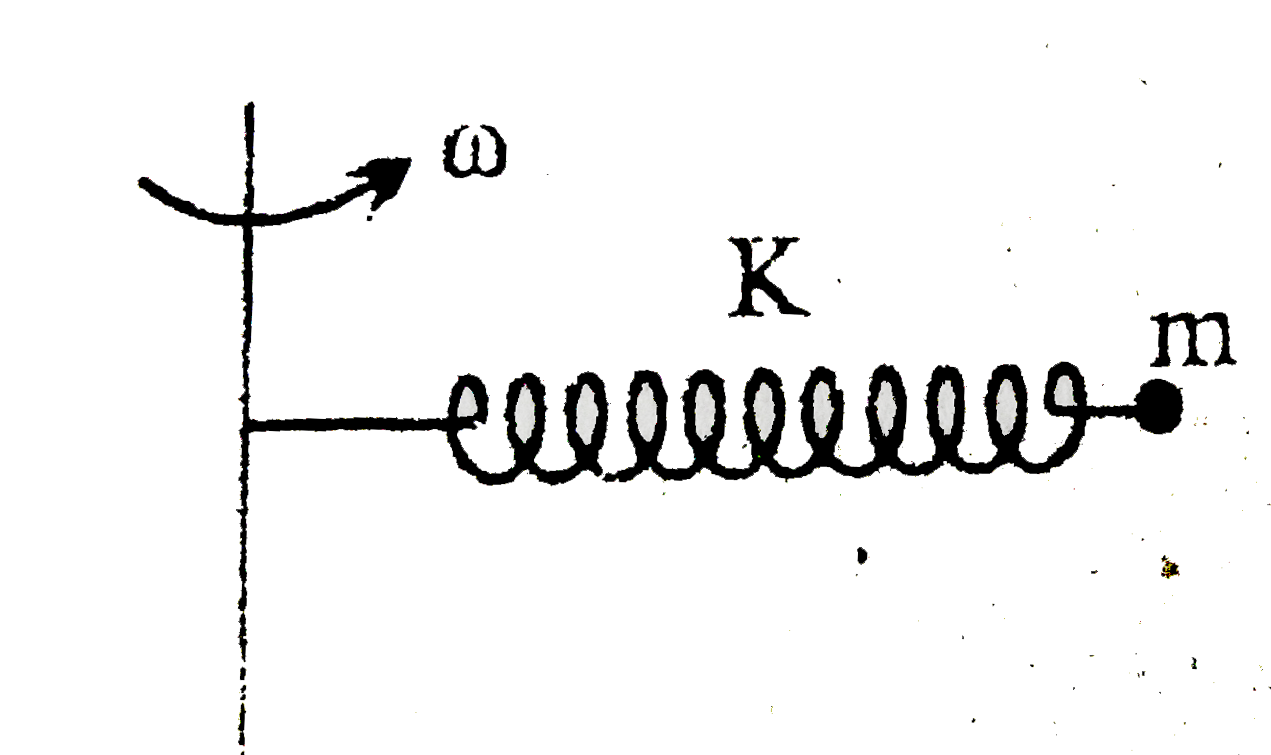 Amω2lkBmω2lk−mω2Cmω2lk+mω2Dnone of these
Amω2lkBmω2lk−mω2Cmω2lk+mω2Dnone of theseA uniform chain of mass M and length L is rotated in a horizontal circle about its one end at an angular frequency ω. The tension at its axis is
AMω2LBMω2L8CMω2L4DMω2L2
A block of mass m is connected at one end of spring fixed at other end having natural length l0 and spring constant K. The block is rotated with constant angular speed (ω) in gravity free space. Find the elongation in spring.
View SolutionA mass m attached to spring of natural length l0 and spring constant k. One end of string is attached to centre of disc in horizontal plane which is being rotated by constant angular speed ω. Find extension per unit length in spring (given k>>mω2 ) :
View SolutionIn a spring- mass system , the length of the spring is L, and it has a mass M attached to it and oscillates with an angular frequency ω. The spring is then cut into two parts, one (i) with relaxed length αL and the other (ii) with relaxed length (1−α) L. The force constants of the two spring A and B are
View SolutionA body of mass m is tied to one end of a spring and whirled round in a horizontal circle with a constant angular velocity .The elongation is 1 cm . If the angular velocity is doubled , the elongation in the spring is 5 cm. what is the original length of the spring ?
View SolutionA mass m is vertically suspended from a spring of negligible mass, the system oscillates with a frequency n. what will be the frequency of the system, if a mass 4m is suspended from the same spring?
View Solution
