An airplane flying at a velocity of 900kmh−1 loops the loop. If the maximum force pressing the pilot against the seat is five times its weight, the loop radius should be
1562 m
1402 m
1315 m
1167 m
The correct Answer is:A
To solve the problem, we need to determine the radius of the loop that the airplane is flying through, given that the maximum force pressing the pilot against the seat is five times the pilot's weight.
1. Convert Velocity to m/s:
The airplane's velocity is given as 900km/h. We need to convert this to meters per second (m/s).
Velocity=900km/h×1000m1km×1h3600s=250m/s
2. Identify Forces at the Top of the Loop:
At the top of the loop, the forces acting on the pilot are:
- Weight (mg) acting downwards.
- Normal force (N) acting downwards (the force pressing the pilot against the seat).
- Centripetal force (Fc) required to keep the airplane in circular motion.
The centripetal force is given by:
Fc=mv2r
3. Set Up the Equation:
According to the problem, the normal force is five times the weight of the pilot:
N=5mg
At the top of the loop, the net force acting towards the center (downwards) is the sum of the weight and the normal force:
N+mg=Fc
Substituting the expressions we have:
5mg+mg=mv2r
Simplifying this gives:
6mg=mv2r
4. Cancel Mass (m):
Since m appears in all terms, we can cancel it out:
6g=v2r
5. Rearranging for Radius (r):
Rearranging the equation to solve for r:
r=v26g
6. Substituting Values:
Now, substitute v=250m/s and g=10m/s2:
r=(250)26×10=6250060=1041.67m
7. Final Calculation:
Simplifying further:
r≈1041.67m
Conclusion:
The radius of the loop should be approximately 1041.67m.
The airplane's velocity is given as 900km/h. We need to convert this to meters per second (m/s).
Velocity=900km/h×1000m1km×1h3600s=250m/s
At the top of the loop, the forces acting on the pilot are:
- Weight (mg) acting downwards.
- Normal force (N) acting downwards (the force pressing the pilot against the seat).
- Centripetal force (Fc) required to keep the airplane in circular motion.
Fc=mv2r
According to the problem, the normal force is five times the weight of the pilot:
N=5mg
At the top of the loop, the net force acting towards the center (downwards) is the sum of the weight and the normal force:
N+mg=Fc
Substituting the expressions we have:
5mg+mg=mv2r
Simplifying this gives:
6mg=mv2r
Since m appears in all terms, we can cancel it out:
6g=v2r
Rearranging the equation to solve for r:
r=v26g
Now, substitute v=250m/s and g=10m/s2:
r=(250)26×10=6250060=1041.67m
Simplifying further:
r≈1041.67m
The radius of the loop should be approximately 1041.67m.
Topper's Solved these Questions
Similar Questions
An aeroplane flying in the sky dives with a speed of 360km/h in a verticale circle of radius 200 m The weight of pilot sitting in it is 75 kg Calulate the force with which the pilot presses his seat when the aeroplane is (i) at the lowest position and (ii) at the highest position Take g=10m/s−2 .
View SolutionA plane flying at a constant speed of 300 m/s makes a wingover* in the vertical plane with the radius 1.3 km. Find the change in weight in the upper and the lower points of the loop.
View Solution
Knowledge Check
A pilot of mass 81 kg loops the loop with steady speed of 300km/h. If the radius is 0.5 km then the force with which the pilot is pressed into the seat at the highest point of the loop ,is (g=10m/s2)
A3.15×102NBzeroC8.10×102ND19.35×102NThe pilot of an aircraft, who is not tied to his seat, can loop a verticle circle in air without falling out at the top of the loop . What is the minimum speed required so that he can successfully negotitate a loop of radius 4 km ? (g=10m/s2)
A100m/sB200m/sC300m/sD400m/sAn object of mass m is released from rest at a height h above the surface of a table. The object slides along the inside of the loop. The loop track consisting of a ramp and a circular loop of radius R shown in the figure. Assume that the track is frictionless. When the object is at the top of the circular track it pushes against the track with a force equal to three times its weight. What height was the object dropped from?
 A3RB4RC5RD6R
A3RB4RC5RD6R
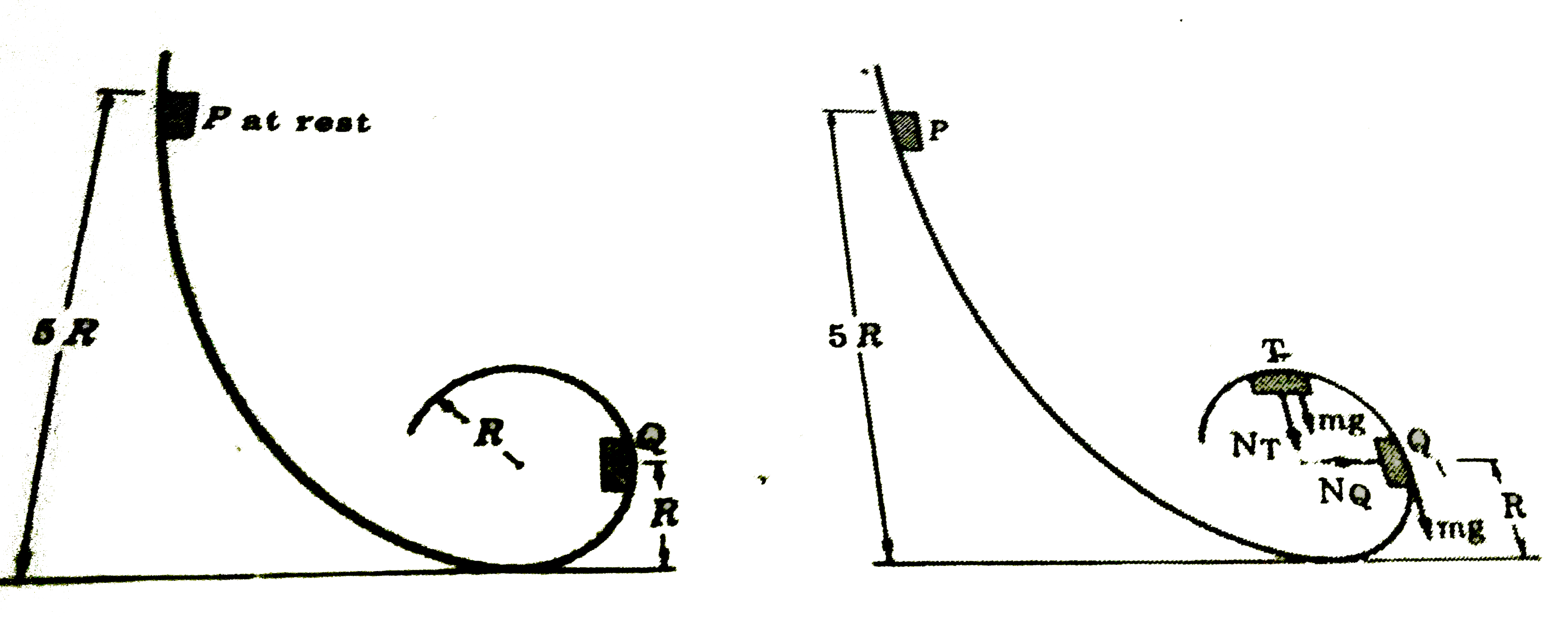
A small block of mass m slides along a smooth track, as shown in fig. (i) If it starts from rest at P, what is the resulting force acting on it at Q ? (ii) at what height above the bottom of the loop should the block be released so that the force it exerts against the track at the top of the loop equals its weight?
View SolutionA conducting circular loop of radius a and resistance per unit length R is moving with a constant velocity v0 , parallel to an infinite conducting wire carrying current i0. A conducting rod of length 2a is approaching the centre of the loop with a constant velocity v02 along the direction 2 of the current. At the instant t=0, the rod comes in contact with the loop at A and starts sliding on the loop with the constant velocity. Neglecting the resistance of the rod and the self-inductance of the circuit, find the following when the rod slides on the loop.
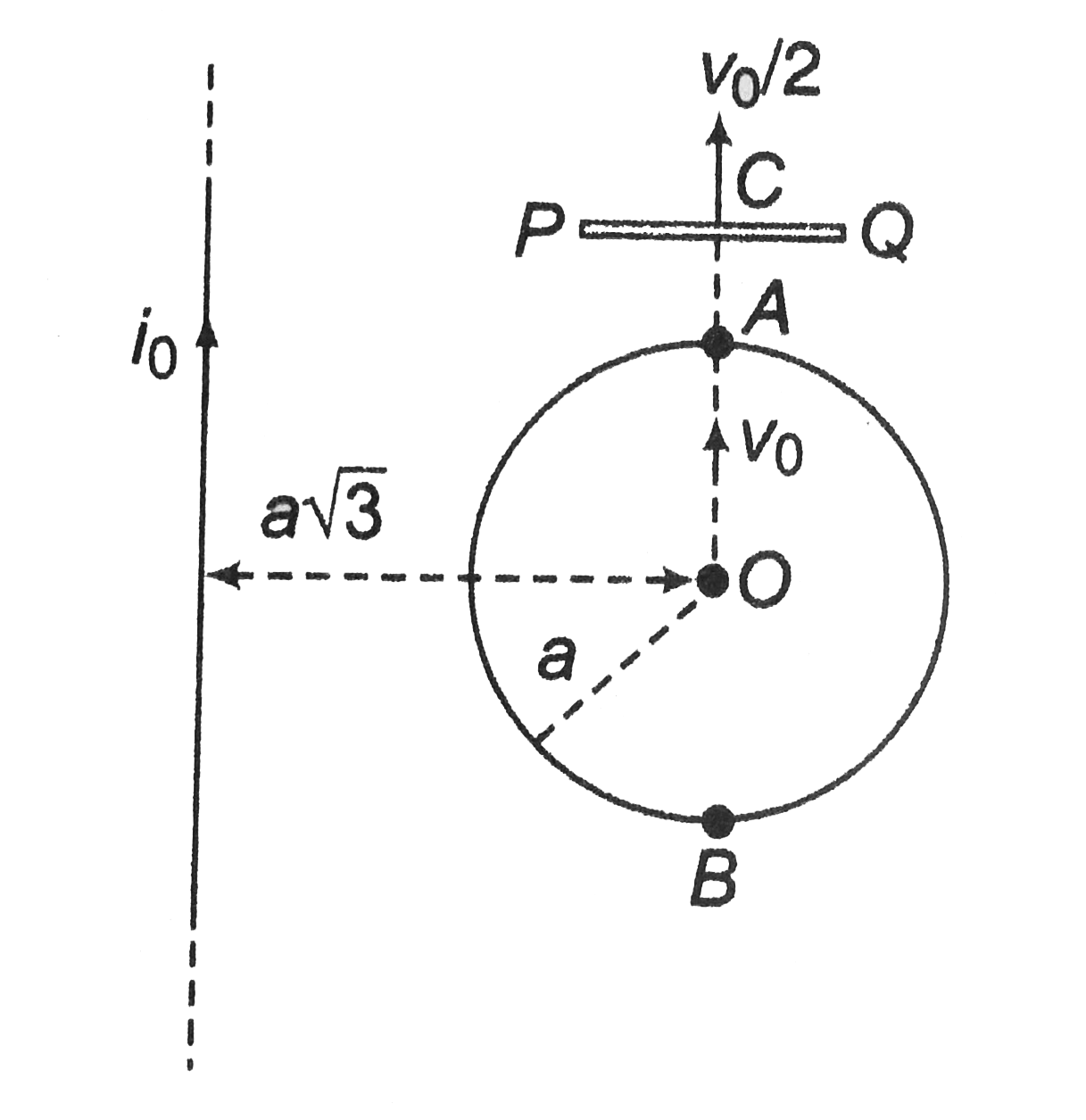
(a) The current through the rod when it is at a distance of (a2) from the point A of the loop.
(b) Force required to maintain the velocity of the rod at that instant.
View SolutionA fighter plane flying in the sky describes a vertical circle of radius 150 m when looping with a speed of 420 km/h . The weight of the pilot sitting inside it is 90 kg . With what force the pilot presses his seat when the plane is at the
(i) highest position of circle
(ii) lowest position of circle
View SolutionAn aeroplane flying in the sky dives with a speed of 360km/h in a verticale circle of radius 200 m The weight of pilot sitting in it is 75 kg Calulate the force with which the pilot presses his seat when the aeroplane is (i) at the lowest position and (ii) at the highest position Take g=10m/s−2 .
View SolutionA fighter aeroplane flying in the sky dives with a speed of 360km/h in a vertical circle of radius 200 m .Weight of the pilot sitting in it is 75 kg . What will be the value of force with which the pilot presses his seat when the aeroplane is at highest position ? (g=10m/s2)
View Solution
