A circuit containing capacitors and as shown in the figure are in steady state with key closed.At the instant , if is opened and is closed then the maximum current in the circuit will be:
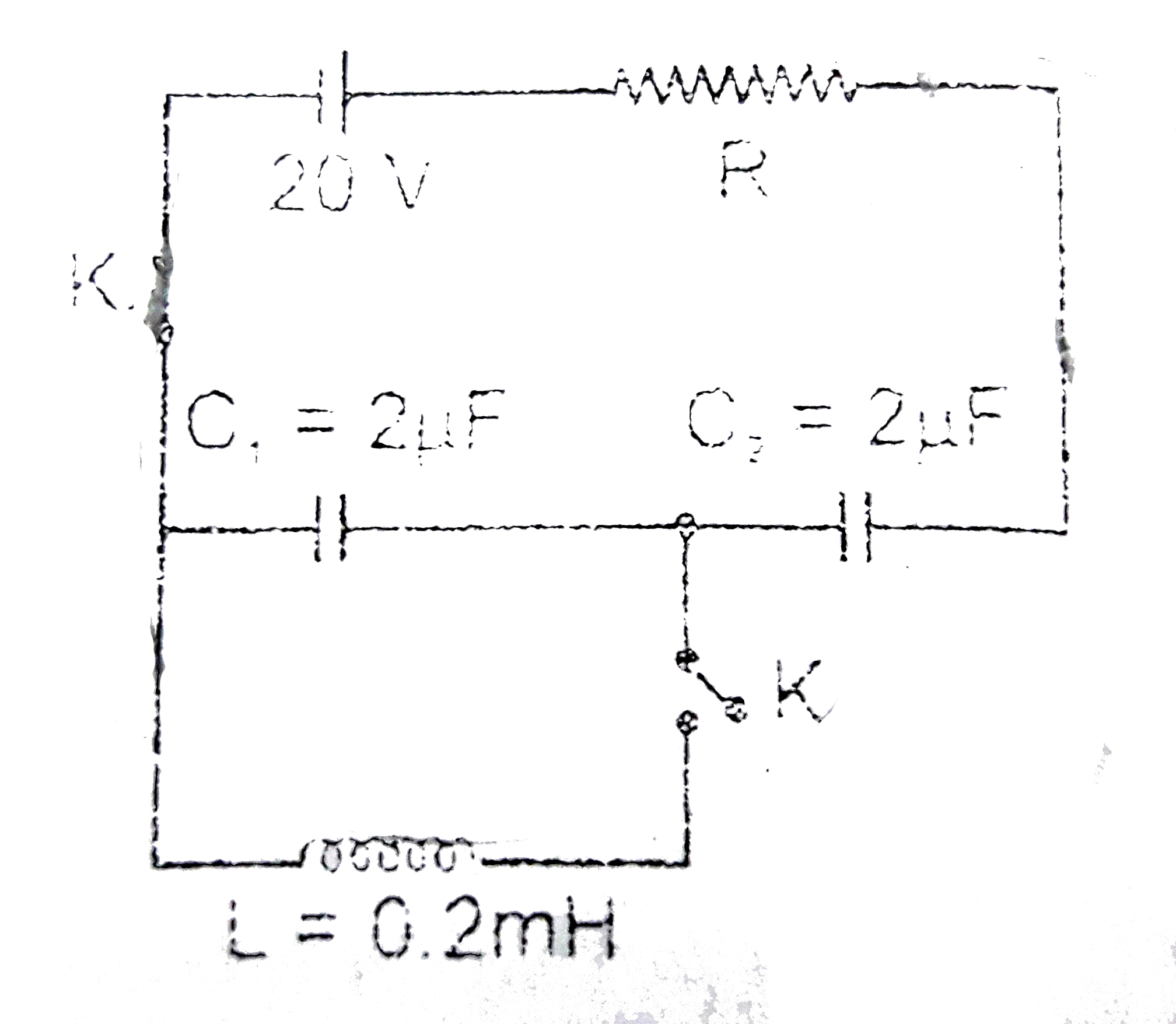
None of these
The correct Answer is:A
When swith is and is closed it becomes circuit so applying energy conservation
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-2 PART 115videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-2 PART 217videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-163videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESONANCECHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems31videos
ELECTRODYNAMICS
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP39videos
ELECTROSTATICS
Similar Questions
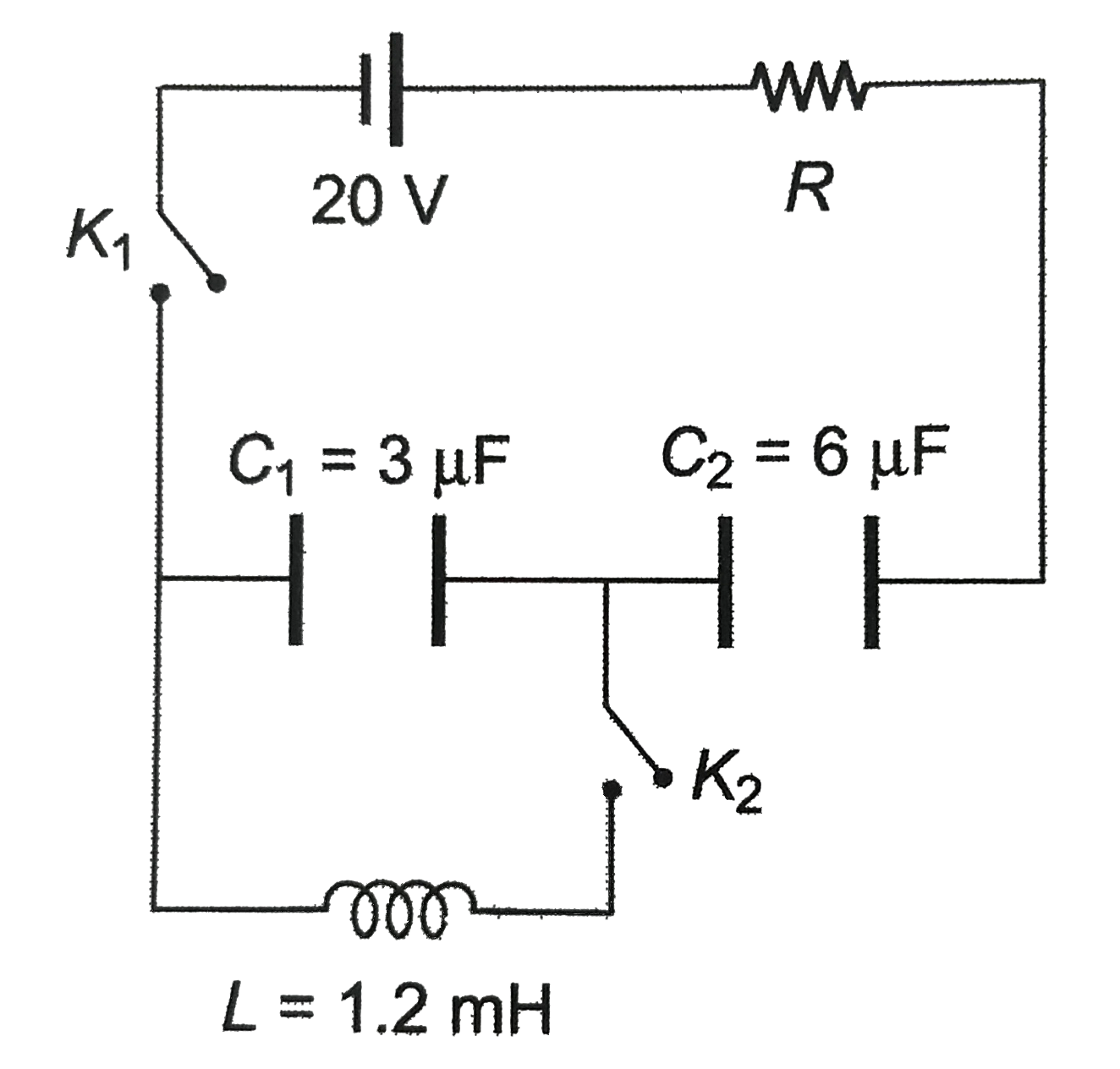
A circuit containing capacitors C1 and C2 shown in the figure is in the steady state with key K1 cloesed. At the instant t=0 , K1 is opened and K2 is closed.
(a) Find the angular frequency of oscillation of the LC circuit.
(b) Determine the first instant t , when enregy in the indctor becomes one third of that in the capacitor.
(c) Calculate the change on the plates of the capacitor at that instant.
 View Solution
View SolutionA circuit containing capacitors C1 and C2, shown in the figure is in the steady state with key K1 and K2 opened. At the instant t=0, K1 is opened and K2 is closed.
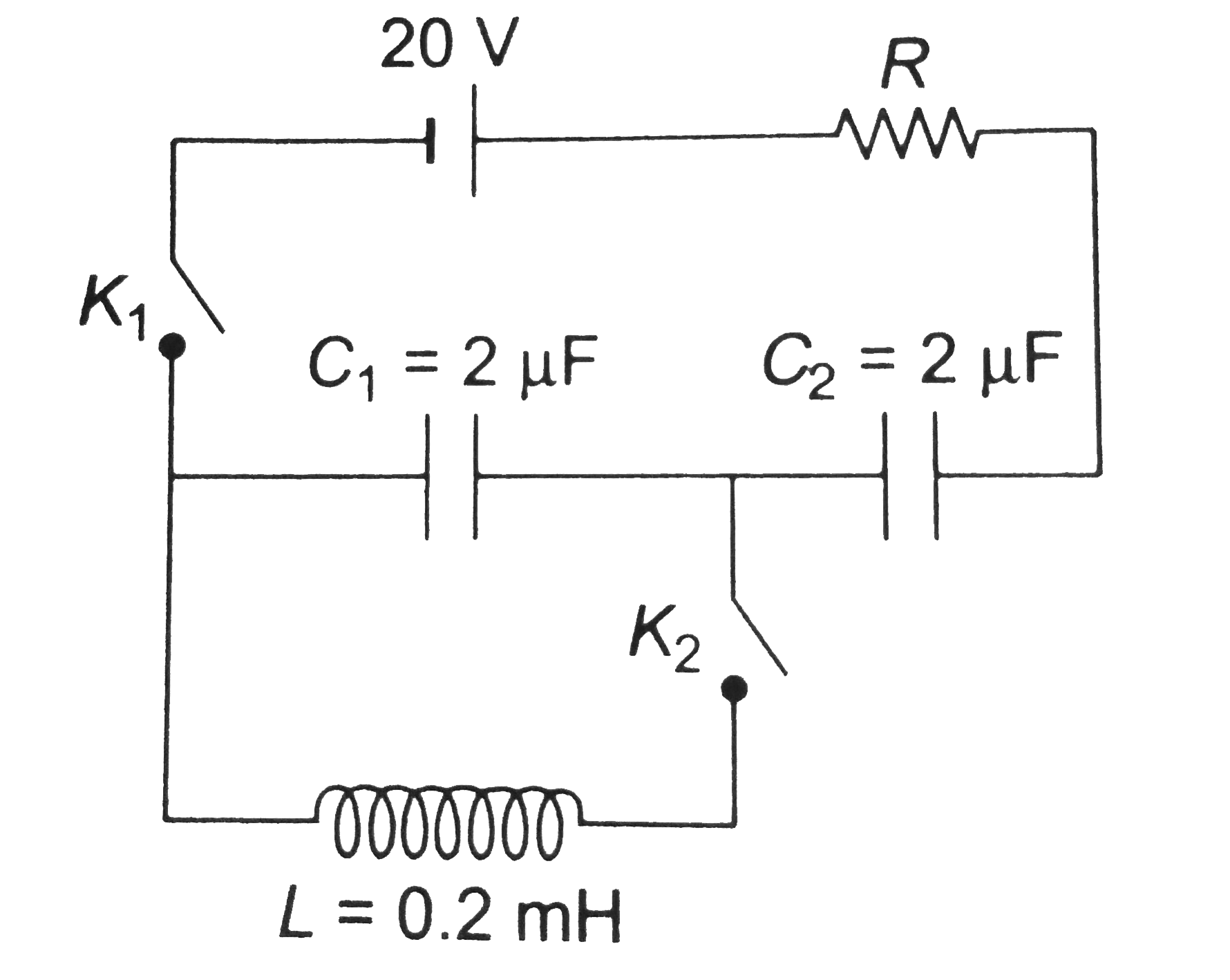
(a) Find the angular frequency of oscillations of L−C circuit.
(b) Determine the first instant t, when energy in the inductor becomes one third of that in the capacitor.
(c) Calculate the charge on the plates of the capacitor at that instant.View Solution
Knowledge Check
- Question 1 - Select One or More
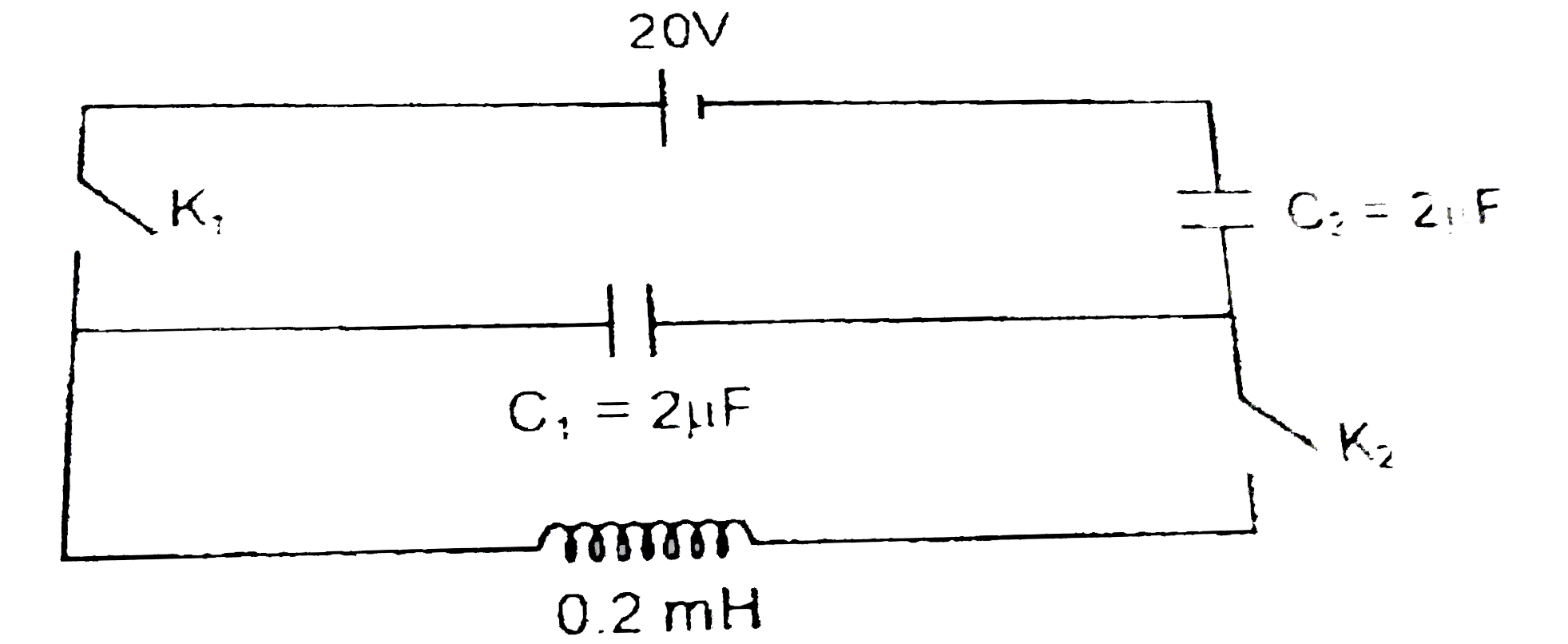
A circuit containing two capacitor C1 and C2 shown in figure is in steady state with switch K1 closed and K2 open. At the instant t=0,K1 is opened and K2 is closed. The correct statements are.AAngular frequency of oscillation of LC circuit is 5×104radsecBcharge on capacitor C1 at t=0 is 40μCCMaximum current in iductor is 1ADCharge on capacitor C1 is 10√3μC when energy in the inductor becomes one third of the energy in capacitor. - Question 2 - Select One
A circuit containing capacitors C1andC2 shown in Fig. is in the steady state with key K1 closed. At the instant t = 0, K1 is opened and K2 is closed. The angular frequency of oscillation of the circuit and charge across C1att=125(μ)s are respectively
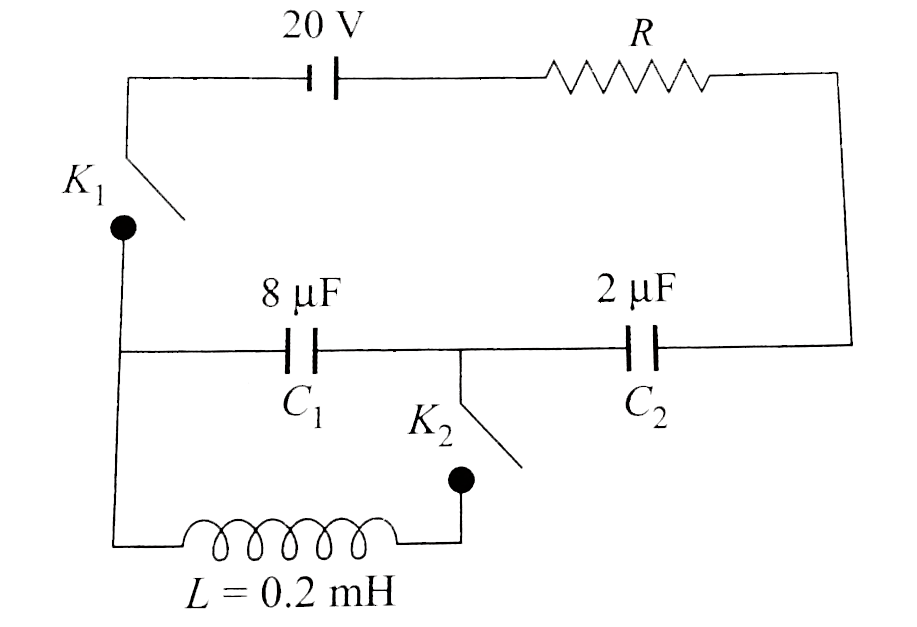 A25×103rads−1,32(μ)CB5×104rads−1,16(μ)CC5×104rads−1,−16(μ)CD25×103rads−1,−32(μ)C
A25×103rads−1,32(μ)CB5×104rads−1,16(μ)CC5×104rads−1,−16(μ)CD25×103rads−1,−32(μ)C - Question 3 - Select One
Shown in the figure is an R−L circuit. Just after the key (K) is closed
 Athe current in the circuit is zeroBpotential drop across the resistor is zeroCemf developed across the inductor equals the emf of the batteryDno heat is dissipated in the circuit
Athe current in the circuit is zeroBpotential drop across the resistor is zeroCemf developed across the inductor equals the emf of the batteryDno heat is dissipated in the circuit
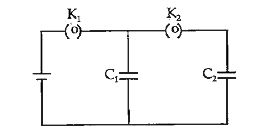
In the circuit shown in Figure, initially key K1 is closed and key K2 is open. Then K1 is opened and K2 is closed. Then
 View Solution
View SolutionThe circuit shown in figure is in the steady state with switch S1 closed. At t=0, S1 is opened and switch S2 is closed.
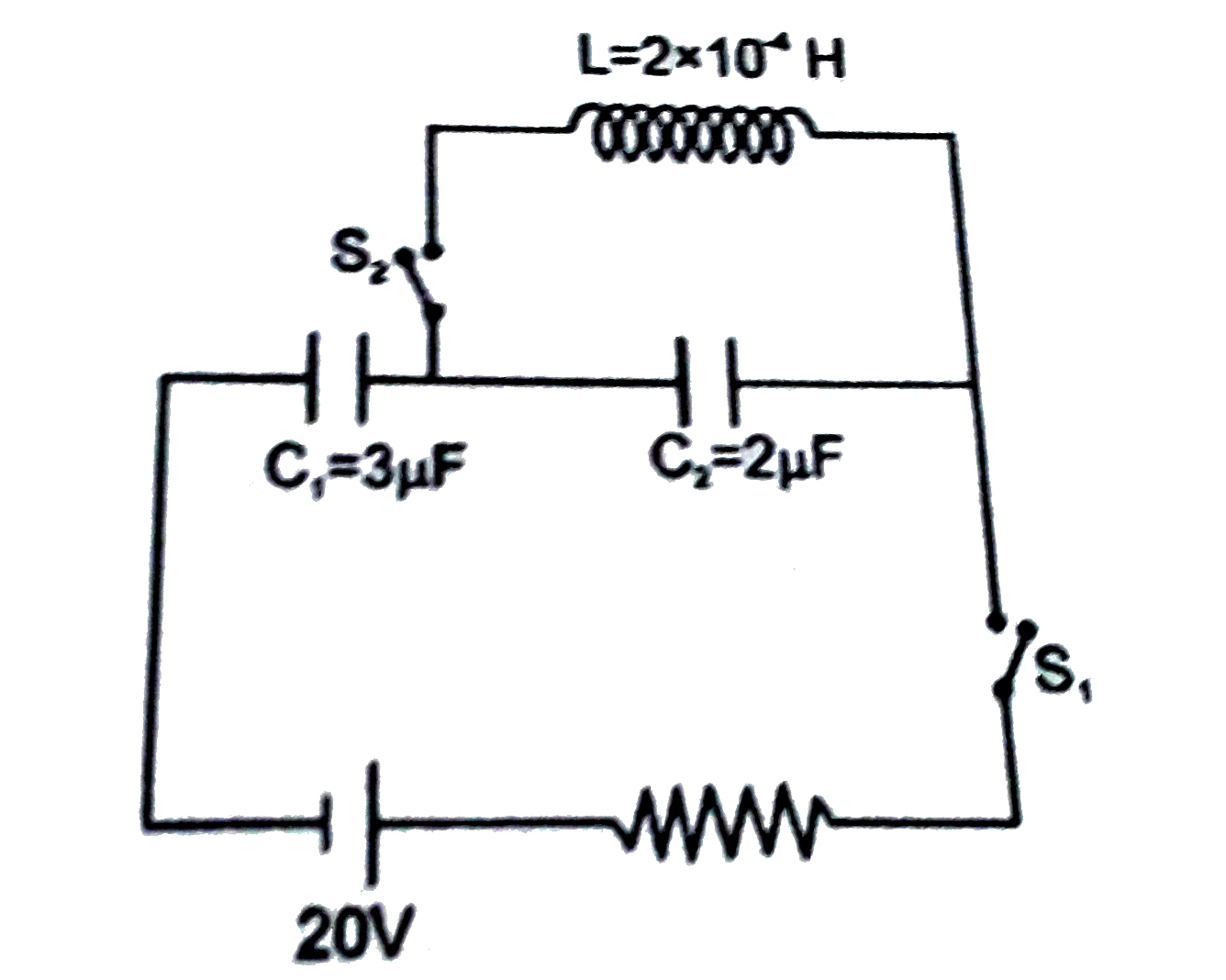
Find the first instant t, when energy in inductor becomes one third of that in capacitorView SolutionThe circuit shown in figure is in the steady state with switch S1 closed.At t=0,S1 is opened and switch S2 is closed. The first instant t when energy in inductor becomes one third of that in capacitor is equal to π×10−5xsec. Then finout value of x.
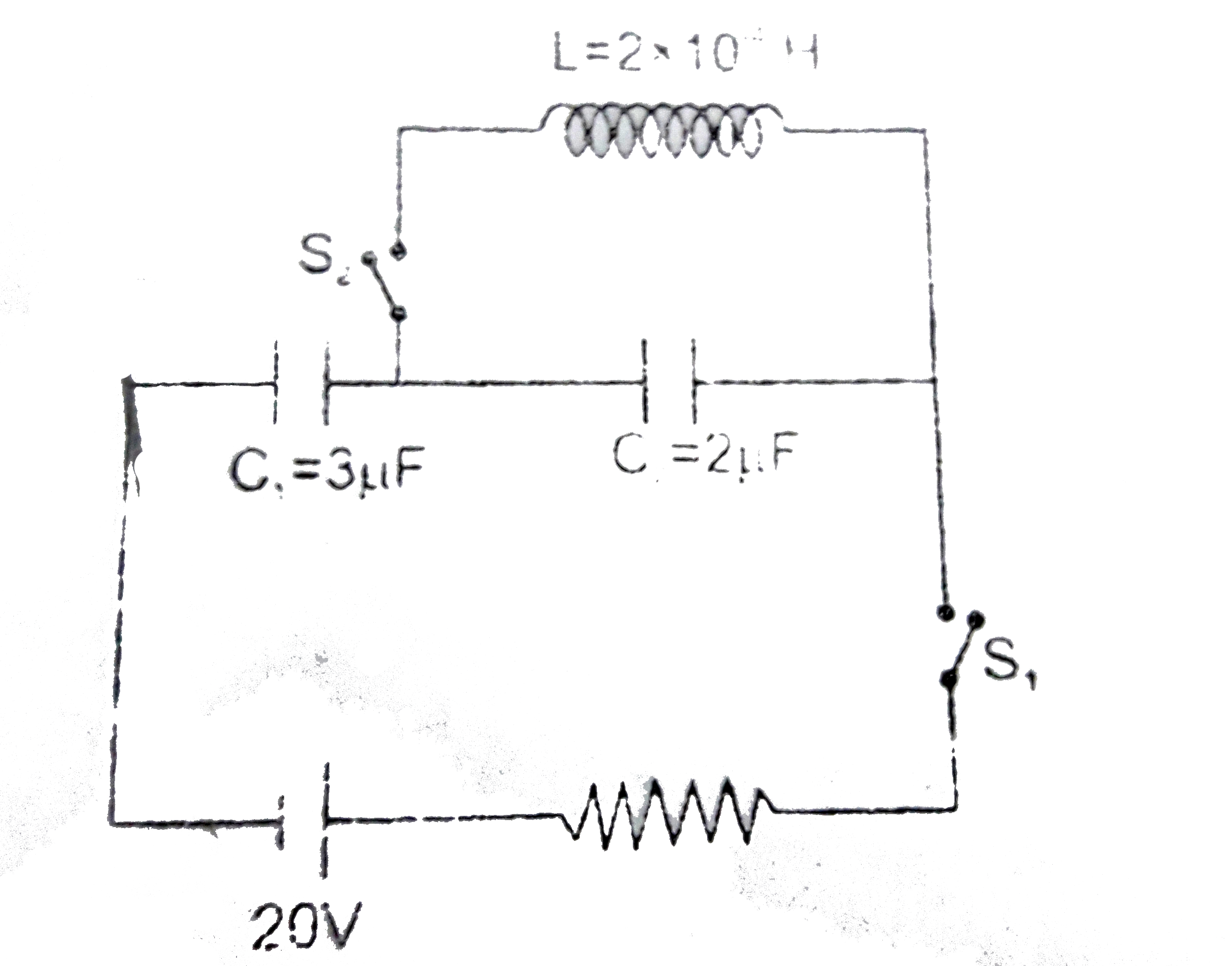 View Solution
View SolutionAs shown in the figure, the key K is closed, the direction of the induced current in the coil B will be -
 View Solution
View SolutionIn the circuit shown in the figure The steady state currents i1andi2 in the coils after the switch S is closed are
 View Solution
View Solution

