A spherical balloon of radius 3 cm containing helium gas has a pressure of 48×10−3 bar . At the same temperature , the pressure , of a spherical balloon of radius 12 cm containing the same amount of gas will be _______ ×10−6 bar
To solve the problem, we will use the relationship between pressure and volume for a gas, which is derived from the ideal gas law. Here are the steps to find the pressure of the second balloon:
Step 1: Understand the relationship between pressure and volume
According to the ideal gas law, for a fixed amount of gas at constant temperature, the product of pressure (P) and volume (V) is constant. Therefore, we can write:
P1V1=P2V2
Since the number of moles and the temperature are constant, we can also say that:
P∝1V
This means that pressure is inversely proportional to volume.
Step 2: Calculate the volume of both balloons
The volume V of a sphere is given by the formula:
V=43πr3
- For the first balloon with radius r1=3 cm:
V1=43π(3)3=43π(27)=36πcm3
- For the second balloon with radius r2=12 cm:
V2=43π(12)3=43π(1728)=2304πcm3
Step 3: Set up the ratio of pressures
Using the relationship P1V1=P2V2 and substituting the volumes we calculated:
P1⋅(36π)=P2⋅(2304π)
We can cancel π from both sides:
P1⋅36=P2⋅2304
Step 4: Solve for P2
Now, we can express P2 in terms of P1:
P2=P1⋅362304
Step 5: Substitute the known values
Given P1=48×10−3 bar:
P2=48×10−3⋅362304
Step 6: Simplify the expression
Calculating the values:
P2=48⋅362304×10−3
Calculating 48⋅36=1728:
P2=17282304×10−3
Now simplifying 17282304=34=0.75:
P2=0.75×10−3 bar
Step 7: Convert to the required format
To express this in terms of 10−6 bar:
P2=0.75×10−3=750×10−6 bar
Final Answer
The pressure of the second balloon is:
750xx10−6 bar
According to the ideal gas law, for a fixed amount of gas at constant temperature, the product of pressure (P) and volume (V) is constant. Therefore, we can write:
P1V1=P2V2
Since the number of moles and the temperature are constant, we can also say that:
P∝1V
This means that pressure is inversely proportional to volume.
The volume V of a sphere is given by the formula:
V=43πr3
- For the first balloon with radius r1=3 cm:
V1=43π(3)3=43π(27)=36πcm3
V2=43π(12)3=43π(1728)=2304πcm3
Using the relationship P1V1=P2V2 and substituting the volumes we calculated:
P1⋅(36π)=P2⋅(2304π)
We can cancel π from both sides:
P1⋅36=P2⋅2304
Now, we can express P2 in terms of P1:
P2=P1⋅362304
Given P1=48×10−3 bar:
P2=48×10−3⋅362304
Calculating the values:
P2=48⋅362304×10−3
Calculating 48⋅36=1728:
P2=17282304×10−3
Now simplifying 17282304=34=0.75:
P2=0.75×10−3 bar
To express this in terms of 10−6 bar:
P2=0.75×10−3=750×10−6 bar
The pressure of the second balloon is:
750xx10−6 bar
Topper's Solved these Questions
Similar Questions
A shopkeer has one spherical laddoo of radius 5 cm. With the same amount of material. How many laddoos of radius 2.5 cm cam be made ?
View SolutionThe radius of spherical balloon is increasing at the rate of 5 cm per second. At what rate is the surface of the balloon increasing, when the radius is 10 cm?
View Solution
Knowledge Check
A spherical balloon of volume 4.00×103cm3 contains helium at a pressure of 1.20×105Pa. How many moles of helium are in the balloon if the average kinetic energy of the helium atoms is 3.60×10−22J?
A3.32molB2.16molC4.12molD2.8molA spherical balloon of volume V contains helium at a pressure P. How many moles of helium are in the balloon if the average kinetic energy of the helium atoms is →K?
A2PV→KNAB3PVKNAC5PV3KNAD3PVKNAA balloon contains 2.0 g of hydrogen gas. A second balloon contains 4.0 g of helium gas. Both balloons are at the same temperature and pressure. Pick the false statement from the following list.
AThe number of hydrogen molecules is the same as the number of helium atoms in each balloonBThe density of the helium in its balloon is greater than the density of the hydrogen in its balloonCThe volume of each balloon is the sameDThe average speed of the molecules/atoms in each balloon is the same
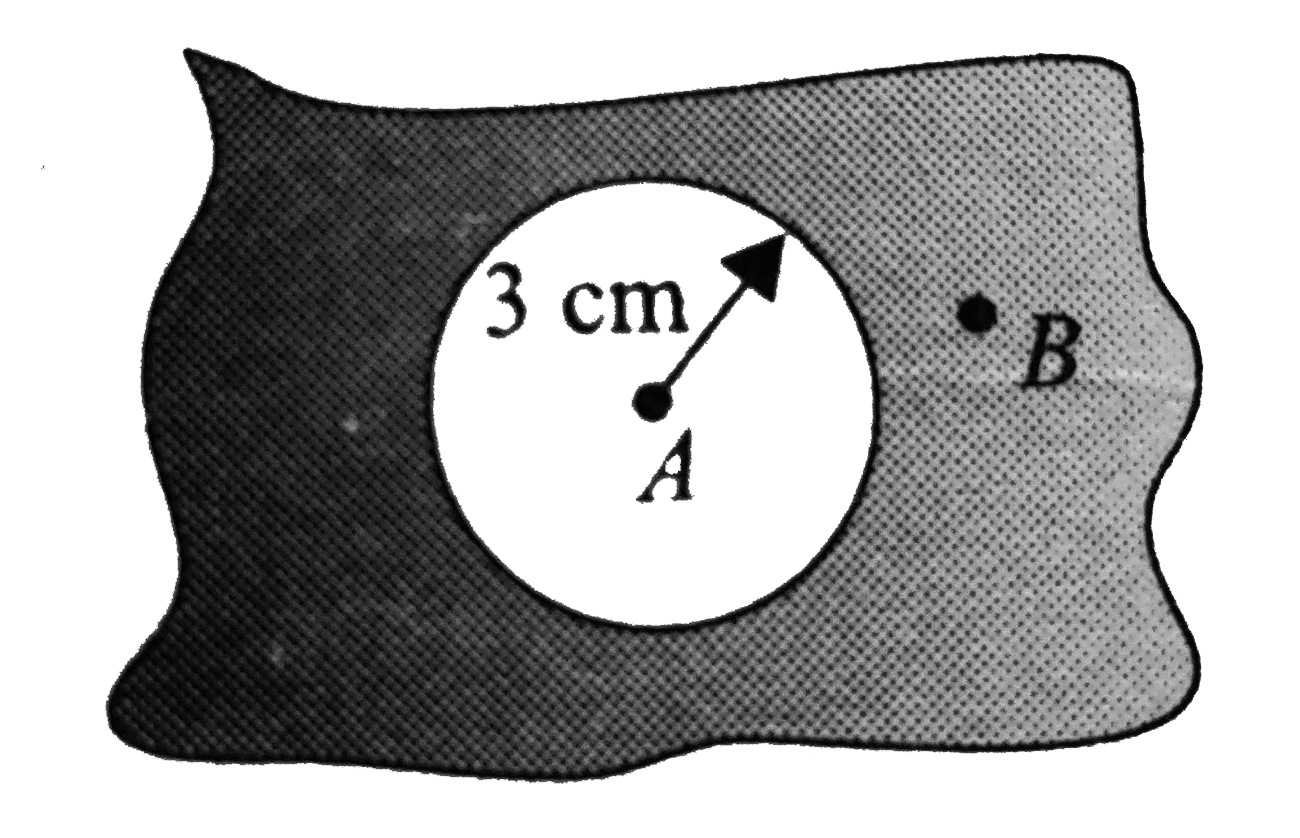
Figure shows a point charge of 0.5×10−6 C at the center of the spherical cavity of radius 3 cm of a piece of metal. The electric field at
View SolutionThe root mean square speed of gas molecules at a temperature 27K and pressure 1.5 bar is 1×104cm/sec If both temperature and pressure are raised three times calculate the new rms speed of gas molecules .
View SolutionA balloon contains 1500m3 of helium at 27∘C and 4 atmospheric pressue. The volume of helium at −3∘Ctemprature and 2 atmospheric pressure will be
View SolutionA vessel contains a gas under a pressure of 5×105pa. If 3/5 of the mass of the gas is flown out, What will be the gas pressure if the temperature being maintained constant,
View SolutionA sample of a given mass of gas at a constant temperature occupies 95cm3 under a pressure of 9.962×104Nm−2. At the same temperature its volume at a pressure of 10.13 xx 10^(4) Nm^(-2) is
View Solution
