A sample of an ideal gas undergoes on isothermal expansion. If dQ, dU and dW represent the amount of heat supplied, the change in internal energy and the work done respectively,then
dQ=+ve,dU=+ve,dW=+ve
dQ=+ve,dU=0,dW=+ve
dQ=+ve,dU=+ve,dW=0
dQ=−ve,dU=−ve,dW=−ve
The correct Answer is:B
To solve the problem regarding the isothermal expansion of an ideal gas, we can follow these steps:
1. Understand the Process:
- The gas undergoes isothermal expansion. This means that the temperature (T) of the gas remains constant throughout the process.
2. Internal Energy Change (dU):
- For an ideal gas, the internal energy (U) depends only on the temperature. Since the temperature is constant during an isothermal process, the change in internal energy (dU) is zero.
dU=0
3. Work Done (dW):
- During expansion, the gas does work on the surroundings. The work done by the gas during an isothermal expansion can be expressed as:
dW=PdV
- Since the volume is increasing (expansion), the work done (dW) is positive.
4. Heat Supplied (dQ):
- According to the first law of thermodynamics, we have:
dQ=dU+dW
- Substituting the values we found:
dQ=0+dW
- Therefore, since dW is positive, we conclude that:
dQ=dW>0
- This indicates that the heat supplied to the system is also positive.
5. Final Relations:
- From the above deductions, we can summarize:
- dU=0
- dW>0
- dQ>0
Conclusion:
The relations for the isothermal expansion of an ideal gas are:
- dU=0
- dW>0
- dQ>0
- The gas undergoes isothermal expansion. This means that the temperature (T) of the gas remains constant throughout the process.
- For an ideal gas, the internal energy (U) depends only on the temperature. Since the temperature is constant during an isothermal process, the change in internal energy (dU) is zero.
dU=0
- During expansion, the gas does work on the surroundings. The work done by the gas during an isothermal expansion can be expressed as:
dW=PdV
- Since the volume is increasing (expansion), the work done (dW) is positive.
- According to the first law of thermodynamics, we have:
dQ=dU+dW
- Substituting the values we found:
dQ=0+dW
- Therefore, since dW is positive, we conclude that:
dQ=dW>0
- This indicates that the heat supplied to the system is also positive.
- From the above deductions, we can summarize:
- dU=0
- dW>0
- dQ>0
The relations for the isothermal expansion of an ideal gas are:
- dU=0
- dW>0
- dQ>0
Topper's Solved these Questions
- THERMODYNAMICSBOOK - AAKASH INSTITUTECHAPTER - THERMODYNAMICSEXERCISE - ASSIGNMENT (SECTION -B) (Objective Type Questions)
23 Videos
- THERMODYNAMICSBOOK - AAKASH INSTITUTECHAPTER - THERMODYNAMICSEXERCISE - ASSIGNMENT (SECTION -C) (Previous Year Questions)
54 Videos
- THERMODYNAMICSBOOK - AAKASH INSTITUTECHAPTER - THERMODYNAMICSEXERCISE - EXERCISE
19 Videos
- THERMAL PROPERTIES OF MATTERBOOK - AAKASH INSTITUTECHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Assignment (Section-J) Akash Challengers Questions
7 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH INSTITUTECHAPTER - UNITS AND MEASUREMENTSEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
Similar Questions
A sample of ideal gas (γ=1.4) is heated at constant pressure. If an amount of 140 J heat is supplied to the gas, then change in internal energy of the gas
View SolutionAn ideal gas is taken from state 1 to state 2 through optional path A,B,CandD as shown in the PV diagram. Let Q,WandU represent the heat supplied, work done and change in internal energy of the gas respectively.
Then,
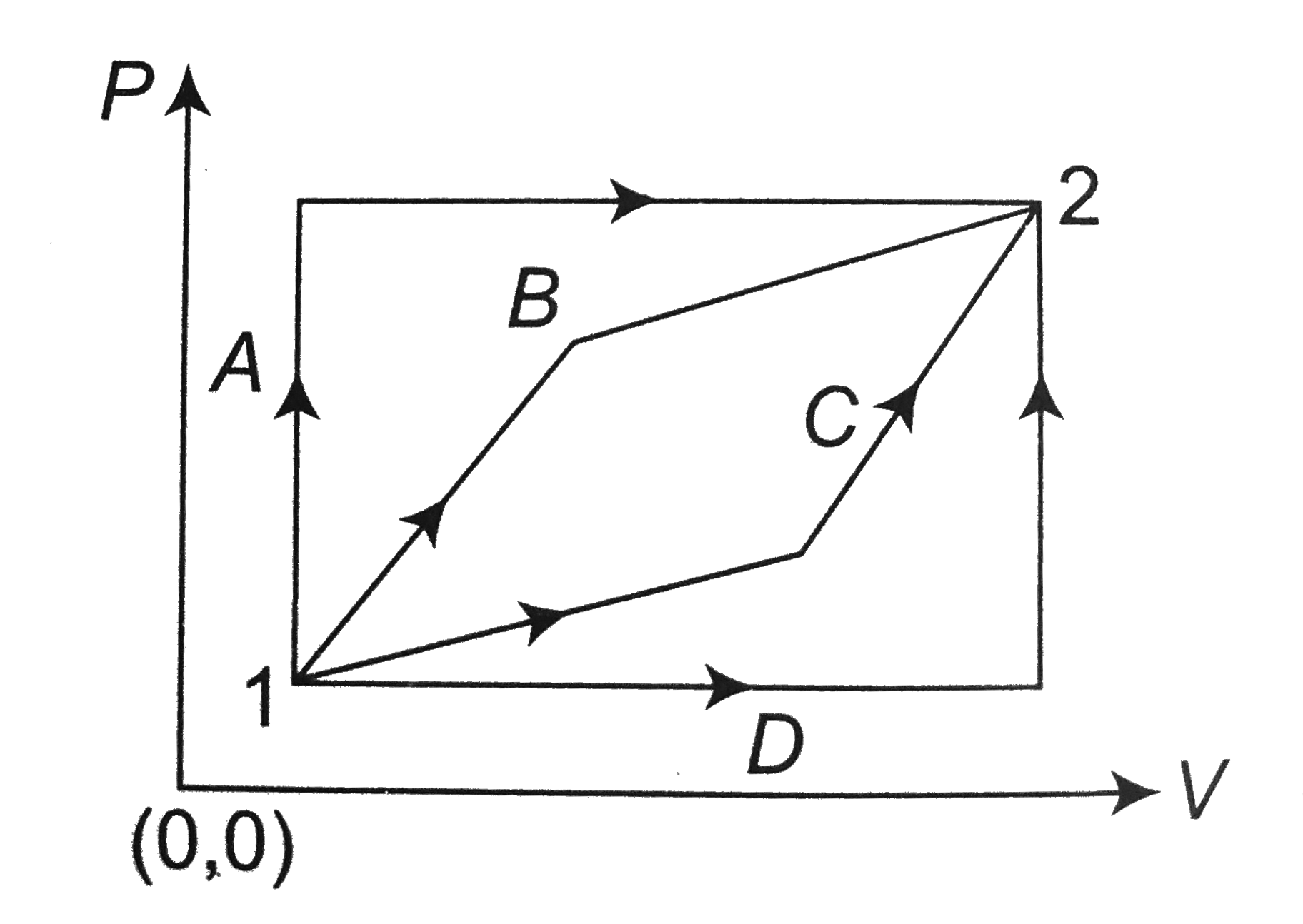
View Solution
Knowledge Check
In an isothermal expansion change in internal energy and work done by the gas are respectively
A(-) ve, (-) veB0, (+) veC0, (-) veD(+) ve, (-) veIn an isothermal expansion change in internal energy and work done by the gas are respectively
A(-)ve, (-) yeB0, (+)veC0,(-)veD(+) ve, (-) ve1 mole of an ideal gas undergoes an isothermal reversible expansion form 10 atm to 1 atm at 300 K. What will be the work done ?
A+57.44 JB−57.44 JC−5744 JD+5744 J
Certain amount of heat supplied to an ideal gas under isothermal conditions will result in
View SolutionChange in internal energy in an isothermal process for ideal gas is
View SolutionAn ideal monoatomic gas undergoes a process in which its internal energy U and density ρ vary as Uρ= constant. The ratio of change in internal energy and the work done by the gas is
View SolutionIn an adiabatic expansion of 2 moles of a gas, the change in its internal energy was found to be –100J. The work done in this process is :
View SolutionDuring an adiabatic expansion of 2 moles of a gas, the change in internal energy was found –50 J . The work done during the process is
View Solution