Tow cells of emf E1 and E2(E1>E2) are connected individually to a potentiometer and their corresponding balancing length are 625 cm and 500 cm, then the ratio E1E2 is .
5:4
3:1
5:1
4:5
The correct Answer is:A
To solve the problem, we need to find the ratio of the EMFs E1E2 using the balancing lengths measured on a potentiometer.
1. Understanding the Potentiometer Principle:
- When two cells are connected to a potentiometer, the EMF of each cell is proportional to the balancing length measured on the potentiometer.
- The relationship can be expressed as:
E1=ΦL1andE2=ΦL2
where Φ is the potential gradient, L1 is the balancing length for E1, and L2 is the balancing length for E2.
2. Setting Up the Ratio:
- From the above equations, we can derive the ratio of the EMFs:
E1E2=ΦL1ΦL2
- The Φ cancels out, leading to:
E1E2=L1L2
3. Substituting the Values:
- We know from the problem statement that:
- L1=625cm
- L2=500cm
- Substituting these values into the ratio gives:
E1E2=625500
4. Simplifying the Ratio:
- To simplify 625500:
625500=625÷125500÷125=54
5. Final Result:
- Therefore, the ratio of the EMFs is:
E1E2=54
Final Answer:
E1E2=54
- When two cells are connected to a potentiometer, the EMF of each cell is proportional to the balancing length measured on the potentiometer.
- The relationship can be expressed as:
E1=ΦL1andE2=ΦL2
where Φ is the potential gradient, L1 is the balancing length for E1, and L2 is the balancing length for E2.
- From the above equations, we can derive the ratio of the EMFs:
E1E2=ΦL1ΦL2
- The Φ cancels out, leading to:
E1E2=L1L2
- We know from the problem statement that:
- L1=625cm
- L2=500cm
- Substituting these values into the ratio gives:
E1E2=625500
- To simplify 625500:
625500=625÷125500÷125=54
- Therefore, the ratio of the EMFs is:
E1E2=54
E1E2=54
Similar Questions
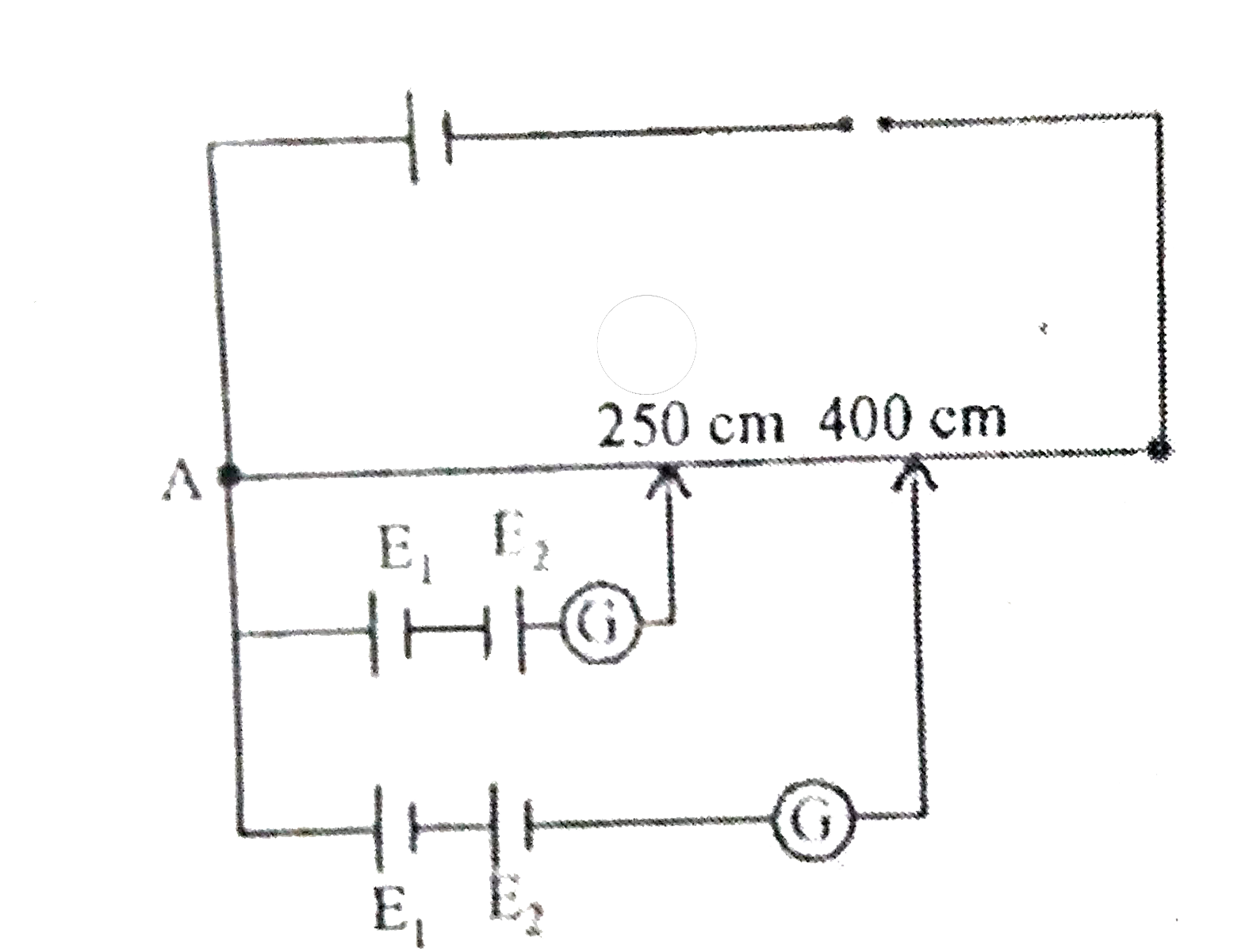
Two primary cells of emfs E1 and E2 are connected to the potentiometer wire AB as shown in the figure . If the balancing lengths for the two combinations of the cells are 250 cm and 400 cm , find the ratio of E1 and E2 .
View SolutionTwo cells of emf E1 and E2(E1>E2) are connected as shown in the figure below. When a potentiometer is used to measure potential difference between the points A and B, the balancing length of the potentiometer wire is 300 cm. But the same potentiometer for the potential difference between points A and C, gives the balancing length 100 cm. Find E1E2.

View Solution
Knowledge Check
Two cells of emf E1 and E2(E1>E2) are connected in series to potentiometer for balancing length 625 cm . When polarity of E2 is reversed then balancing length becomes 125 cm. Then the ratio E1E2 is
A3:2B3:1C5:1D2:1Two cells of emfs E1 and (E2(E1>E2) are connected as shows in Fig. 6.45.
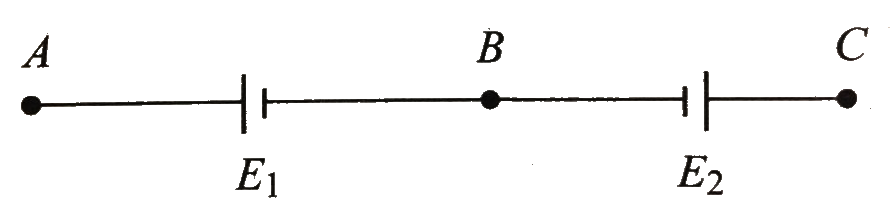
When a potentiometer is connected between A and B, the balancing length of the potentiometer wire is 300cm. On connecting the same potentiometer between A and C, the balancing length is 100cm. The ratio E1/E2 isA3:1B1:3C2:3D3:2Two cells of E.M.F. E1 and E2(E2>E1) are connected in series in the secondary circuit of a potentiometer experiment for determination of E.M.F. The balancing length is found to be 825 cm. Now when the terminals to cell E1 are reversed, then the balancing length is found to be 225 cm. The ratio of E1 and E2 is
A2:3B4:7C7:4Dnone of these
Two batteries of e.m.f. E1 and E2 and internal resistance r1 and r2 are connected in parallel. Determine their equivelent e.m.f.
View SolutionTwo cells of e.m.f.'s E1andE2 where E1>E2, are connected in series so as to assist each other, the balancing length is 2.7m . When the cells are connected in series so as to oppose each other, the balancing length is found to be 0.3m . What is the ratio of their e.m.f.'s ?
View SolutionTwo cells of emf E1 and E2 are to be compared in a potentiometer (E1>E2) . When the cells are used in series correctly , the balancing length obtained is 400 cm. When they are used in series but E2 is connected with reverse polarities, the balancing obtaiend is 200cm. Ratio of emf of cells is
View SolutionIn the above question, if the balancing length for a cell of emf E is 60cm, the value of E will be
View SolutionTow cells of e.m.f E1 and E2 are joined in series and the balancing length of the potentiometer wire is 625 cm. If the terminals of E1 are reversed , the balancing length obtained is 125 cm. Given E2>E1,the ratio E1:E2 willbe
View Solution