An alloy consists of rubidium and one of the other alkali metals. A sample of 4.6g of the alloy when allowed to react with water, liberates 2.241dm3 of hydrogen at STP.
Relative atomic masses: AT(Li)=7,AT(Na)=23,AT(K)=39,AT(Rb)=85.5,AT(Cs)=1.33
What composition in % by mass has the alloy?
The correct Answer is:Concerning the molar masses of alkali metals, only lithium can come into consideration, i.e. the alloy consists of rubidum and lithium.
n(Rb)+n(Li)=0.2mol
m(Rb)+m(Li)=4.6g
n(Rb)M(rb)+n(Li)M(Li)=4.6g<br>n(Rb)M(Rb)M(Rb)+(0.2−n(Rb))M(Li)=4.6
n(Rb).85.5+(0.2−n(Rb))7=4.6
n(Rb)=0.0408 mol
n(Li)=0.1592 mol
%Rb=0.0408mol×85.5gmol−14.6g×100=76\
Step by step video, text & image solution for An alloy consists of rubidium and one of the other alkali metals. A sample of 4.6g of the alloy when allowed to react with water, liberates 2.241dm^(3) of hydrogen at STP. Relative atomic masses: A_(T)(Li)=7,A_(T)(Na)=23,A_(T)(K)=39,A_(T)(Rb)=85.5,A_(T)(Cs)=1.33 What composition in % by mass has the alloy? by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CHEMICAL EQUILIBRIUMBOOK - RESONANCECHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Advanced Level Problems (Part-3)(Stage-2)
4 Videos
- CHEMICAL BONDINGBOOK - RESONANCECHAPTER - CHEMICAL BONDINGEXERCISE - Inorganic chemistry (Chemistry Bonding)
49 Videos
- D & F-BLOCK ELEMENTS & THEIR IMPORTANT COMPOUNDS BOOK - RESONANCECHAPTER - D & F-BLOCK ELEMENTS & THEIR IMPORTANT COMPOUNDS EXERCISE - Match the column
1 Videos
Similar Questions
An alloy consists of rubidium and one of the other alkali metals. A sample of 4.6g of the alloy when allowed to react with water, liberates 2.241dm3 of hydrogen at STP.
Relative atomic masses: AT(Li)=7,AT(Na)=23,AT(K)=39,AT(Rb)=85.5,AT(Cs)=1.33
Which alkali metal is the component of the alloy?
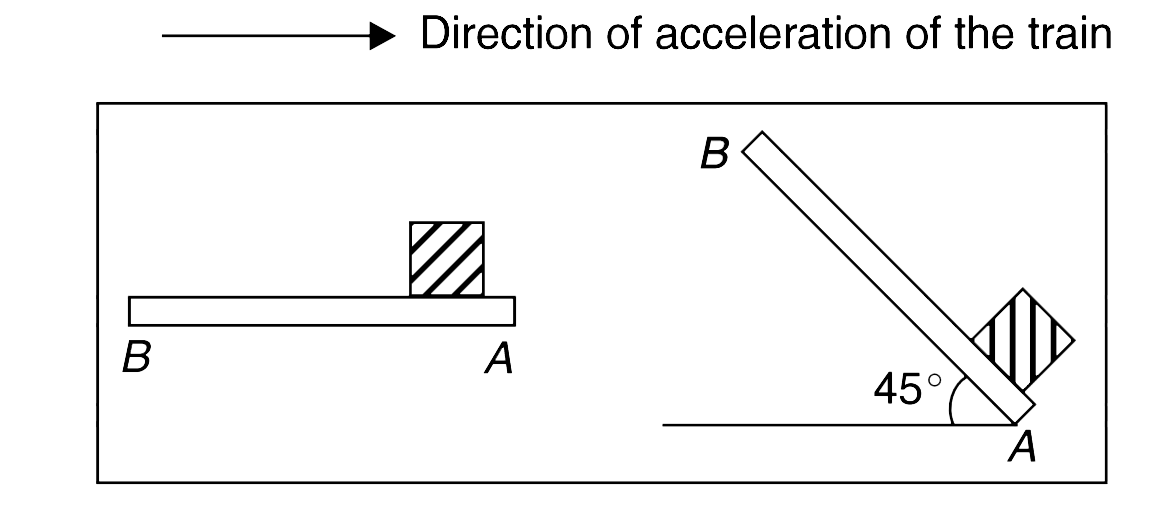
View SolutionAn experimenter is inside a uniformly accelerated train. Train is moving horizontally with constant acceleration a0. He places a wooden plank AB in horizontal position with end A pointing towards the engine of the train. A block is released at end A of the plank and it reaches end B in time t1. The same plank is placed at an inclination of 45∘ to the horizontal. When the block is released at A it now climbs to B in timet2. It was found that t2t1=254. What is the coefficient of friction between the block and the plank?
View Solution
Knowledge Check
Adenine is a substituted form of a heterocyclic nitrogenous base Purine. Adenine is found in both polymers DNA and RNA. Adenine is composed of the elements carbon, hydrogen and nitrogen and has molecular mass of 13.5aμ. Upon analysis, it was found that the ratio of % (by w.t.) of carbon to that of total % (by wt) of hydrgoen and nitrogen was found to be 4:5 respectively. The ratio of number of N atoms to hydrogen atoms is 1:1.
Now, answer the following questions:
The amount of glucose (C6H12O6) obtained when all the carbon in 36mg of adenine is converted into glucose is :A0.22m moleB0.26m moleC48mgD40mgA cubical box of side 1m contains helium gas (atomic weight 4) at a pressure of 100N/m2. During an observation time of 1second, an atom travelling with the root - mean - square speed parallel to one of the edges of the cube, was found to make 500hits with a particular wall, without any collision with other atoms . Take R=253j/mol−Kandk=1.38×10−23J/K.
(a) Evaluate the temperature of the gas.
(b) Evaluate the average kinetic energy per atom.
( c) Evaluate the total mass of helium gas in the box.A0.6 gB0.3 gC0.4 gD0.7 gA cubical box of side 1m contains helium gas (atomic weight 4) at a pressure of 100N/m2. During an observation time of 1second, an atom travelling with the root - mean - square speed parallel to one of the edges of the cube, was found to make 500hits with a particular wall, without any collision with other atoms . Take R=253j/mol−Kandk=1.38×10−23J/K.
(a) Evaluate the temperature of the gas.
(b) Evaluate the average kinetic energy per atom.
( c) Evaluate the total mass of helium gas in the box.A6 gB4 gC8 gD12 g
Hydrogen Atom and Hydrogen Molecule
The observed wavelengths in the line spectrum of hydrogen atom were first expressed in terms of a series by johann Jakob Balmer, a Swiss teacher. Balmer's empirical empirical formula is
1λ=RH(122−1n2), N=3,4,5
RH=Mee48ε20h3c=109.678cm−1
Here,
RH is the Rydberg Constant, me is the mass of electron. Niels Bohr derived this expression theoretically in 1913. The formula is easily generalized to any one electron atom//ion.
Determine the loest energy and the radius of the Bohr orbit of the muonic hydrogen atom. Ignore the motion of the nucleus in your calculation. The radius of the Bohr orbit of a hydrogen atom
(called the Bohr radius,a0=ε0h2mee2∏is0.53Å)
The classical picture of an ''orbit'' in Bohr's theory has now been replaced by the quantum mechanical nation of an 'orbital'. The orbital ψ1σ1s(r) for the ground state of a hydrogen atom is given by
ψ1s(r)=1√∏a30era0
where r is the distance of the electron from the nucleus and a0 is the Bohr radius.
View SolutionA harmonic wave is travelling on string 1. At a junction with string 2 it is partly reflected and partly transmitted . The linear mass density of the second string is four times that of the first string , and that the boundary between the two strings is at x=0 . If the expression for the incident wave is yi=Aicos(k1x−ω1t).
What are the expressions for the transmitted and the reflected waves in terms of Ai,K1andω1?
(b) Show that the average power by the incident wave is equal to the sum of the average power carried by the transmitted and reflected waves .
View Solutiona. If the velocity of a body is zero, does it mean that its acceleration is also zero ? (Yes//No)
b. If the acceleration of a body is zero does it mean that its velocity is also zero ? (Yes//No)
c. If a body travels with uniform acceleration a1 for a time t2 thenthe average acceleration is given by
aav=a1t1+a2t2t1+t2(Yes/No)<br>d.Ifabody⋆tsomrestandmoveswithuniformae≤rationthedisplacemectsofthebodyis1 s,<br>2 s, 3 s,etc.,are∈therationof(1:4:9),etc.(True/False)
e. For a body moving whith uniform acceleration, the displacent of the bosy in successive seconds is in the ration of 1:3:5:7.........................(True//False) .
View SolutionA harmonic waves is travelling on string 1. At a junction with string 2 it is partly reflected and partly transmitted. The linear mass density of the second string is four time that of the first string. And that the boundry between the two strings is at x=0. If tje expression for the incident wave is, yi=Aicos(k1x−ω1t)
What are the expression for the transmitted and the reflected waves in terms of Ai,k1 and ω1 ?
View SolutionA mixture of two solid elements with a mass of 1.52g was treated with an excess of hydrochloric acid. A volume of 0.896dm3 of a gas was liberated in this process and 0.56g of a residue remained which was undissolved in the excess of the acid.
In another experiment, 1.52g of the same mixture were allowed to react with an excess of a 10% sodium hydroxide solution. In this case 0.896dm3 of a gas were also evolved but 0.96g of an undissolved residue remained.
In the third experiment, 1.52g of the initial mixture were heated to high temperature without acess of the air. In this way a compound was formed which was totally soluble in hydrochloric acid and 0.448dm3 of an unknown gas were released. All the gas obtained was introduced into a one litre closed vessel filled with oxygen. After the reaction of the unknown gas with oxygen the pressure in the vessel decreased by approximately ten times (T=const).
Write chemical equations for the above reactions and prove their correctness by calculations. In solving the problem consider that the volumes of gases were measured at STP and round up the relative atomic masses to whole numbers.
View Solution
