A particle is executing vertical SHM about the highest point of a projectile. When the particle is at the mean position, the projectile is fired from the ground with velocity u at an angle θ with the horizontal.The projectile hits the oscillating particle .Then, the possible time period of the particle is
usinθg
2usinθg
2usinθ3g
all the above
The correct Answer is:D
To solve the problem, we need to analyze the motion of both the projectile and the particle executing vertical Simple Harmonic Motion (SHM). Here’s a step-by-step solution:
Step 1: Understand the Motion of the Projectile
The projectile is launched from the ground with an initial velocity u at an angle θ with the horizontal. We can break this velocity into its horizontal and vertical components:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
Step 2: Calculate the Time of Flight of the Projectile
The total time of flight T for a projectile launched at an angle θ is given by the formula:
T=2usinθg
where g is the acceleration due to gravity.
Step 3: Determine the Time to Reach the Highest Point
The time taken to reach the highest point (where the vertical component of velocity becomes zero) is half of the total time of flight:
tup=T2=usinθg
Step 4: Analyze the SHM of the Particle
The particle is executing vertical SHM about the highest point of the projectile. The mean position of the SHM corresponds to the equilibrium position where the particle oscillates.
Step 5: Condition for Collision
For the projectile to collide with the particle executing SHM, the particle must be at its mean position when the projectile reaches its highest point. This means that the time taken for the projectile to reach its highest point must equal the time taken for the particle to complete half of its oscillation.
Step 6: Relate the Time of Flight to the Time Period of SHM
Let Tp be the time period of the particle executing SHM. For the particle to be at the mean position when the projectile reaches its highest point, we have:
tup=Tp2
Substituting tup:
usinθg=Tp2
Step 7: Solve for the Time Period of the Particle
Rearranging the equation gives:
Tp=2usinθg
Step 8: Consider Other Possible Cases
The problem states that there can be multiple scenarios for the collision:
1. The projectile hits the particle at the mean position.
2. The projectile hits the particle when it is at the positive extreme.
3. The projectile hits the particle when it is at the negative extreme.
For these cases, the time period can also be expressed as:
- For the first case, Tp=2usinθg
- For the second case, Tp=2usinθ3g
- For the third case, Tp=3usinθ2g
Conclusion
The possible time periods of the particle can be:
1. Tp=2usinθg
2. Tp=2usinθ3g
3. Tp=3usinθ2g
The projectile is launched from the ground with an initial velocity u at an angle θ with the horizontal. We can break this velocity into its horizontal and vertical components:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
The total time of flight T for a projectile launched at an angle θ is given by the formula:
T=2usinθg
where g is the acceleration due to gravity.
The time taken to reach the highest point (where the vertical component of velocity becomes zero) is half of the total time of flight:
tup=T2=usinθg
The particle is executing vertical SHM about the highest point of the projectile. The mean position of the SHM corresponds to the equilibrium position where the particle oscillates.
For the projectile to collide with the particle executing SHM, the particle must be at its mean position when the projectile reaches its highest point. This means that the time taken for the projectile to reach its highest point must equal the time taken for the particle to complete half of its oscillation.
Let Tp be the time period of the particle executing SHM. For the particle to be at the mean position when the projectile reaches its highest point, we have:
tup=Tp2
Substituting tup:
usinθg=Tp2
Rearranging the equation gives:
Tp=2usinθg
The problem states that there can be multiple scenarios for the collision:
1. The projectile hits the particle at the mean position.
2. The projectile hits the particle when it is at the positive extreme.
3. The projectile hits the particle when it is at the negative extreme.
- For the first case, Tp=2usinθg
- For the second case, Tp=2usinθ3g
- For the third case, Tp=3usinθ2g
The possible time periods of the particle can be:
1. Tp=2usinθg
2. Tp=2usinθ3g
3. Tp=3usinθ2g
Topper's Solved these Questions
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - SECTION (II) : (SUBJECTIVE )
33 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - SECTION(II) : MCQ ( SINGLE CORRECT)
47 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - NUMERICAL BASED QUESTIONS
3 Videos
- HEAT AND TEMPERATUREBOOK - FIITJEECHAPTER - HEAT AND TEMPERATUREEXERCISE - NUMERICAL BASES QUESTIONS
1 Videos
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - COMPREHENSION-III
2 Videos
Similar Questions
Knowledge Check
A particle is fired from a point on the ground with speed u making an angle θ with the horizontal.Then
Athe radius of curvature of the projectile at the highest point is u2cos2θgBthe radius of curvature of the projectile at the highest point is u2sin2θgCat the point of projection magnitude of tangential acceleration is gsinθDat the point of projection magnitude of tangential acceleration is gcosθA particle is fired with velocity u making angle θ with the horizontal.What is the change in velocity when it is at the highest point?
AucosθBuCusinθDucosθ−uA projectile is projected with a velocityv0 at an angle θ with the horizontal as shown in figure. The angular momentum of particle about the origin:
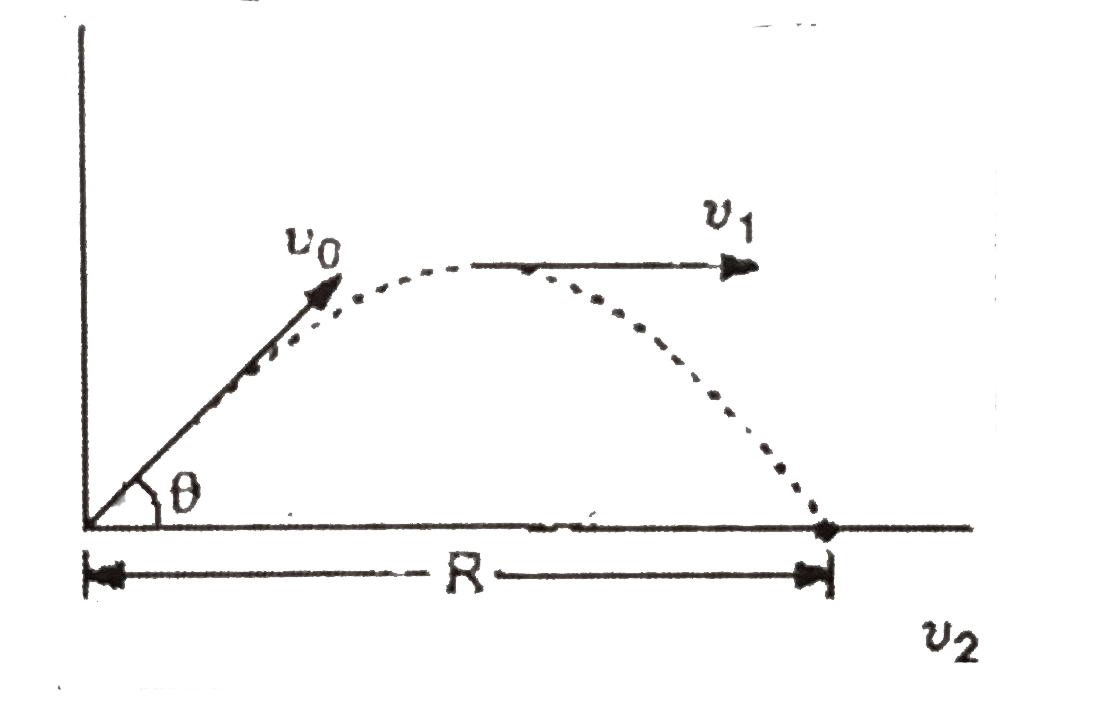 Ais zero when particle is at the originBis −mv3sin2θcosθ2gˆk when particle is at the highest point of trajectoryCis −2mv2sin2θcosθgˆk when particle is just about to hit groundDdownward force of gravity exerts a torque in −z direction.
Ais zero when particle is at the originBis −mv3sin2θcosθ2gˆk when particle is at the highest point of trajectoryCis −2mv2sin2θcosθgˆk when particle is just about to hit groundDdownward force of gravity exerts a torque in −z direction.
A projectile is fired from horizontal at an angle θ . What are the horizontal and vertical acceleration at the highest point ?
View SolutionA projectile is projected from horizontal with velocity (u) making an angle 45∘ with the horizontal direction. Find the distance of the highest point of the projectile from its starting point.
View SolutionA projectile is thrown with a velocity u0 at an angle θ with the horizontal. The ratio of the rate of change of speed w.r.t. time, at highest point to that at the point of projection, is
View SolutionA particle is fired with velocity u making angle θ with the horizontal.What is the change in velocity when it is at the highest point?
View SolutionA particle of mass 'm' is projected from ground with velocity u making angle θ with the vertical. The de-Broglie wave length of the particle at the highest point is:
View Solution

