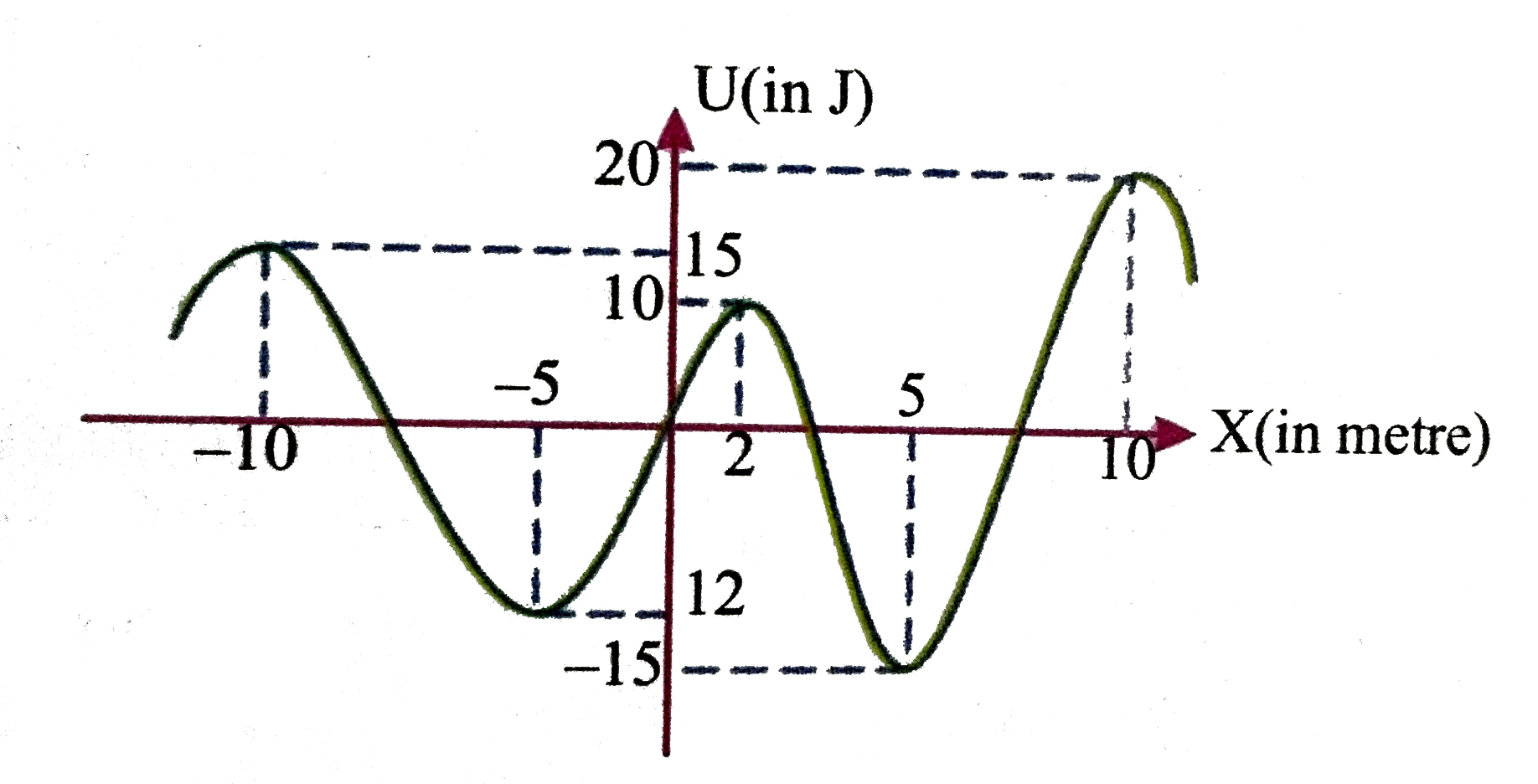
The variation of potential energy of a particle of mass m = 5kg to its x-coordinate . The particle moves under the effect of this conservative force only along the x-axis. Then, choose the correct option.
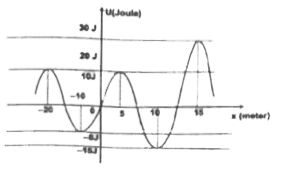
If the particle is released at the origin, then it will move towards x-axis.
At x=10 particle is in unstable equilibrium
If the particle is released at x=5+Δx, where Δx→0(Δx>0) then its maximum speed is √10m/sec.
At x= 15m, the particle is in stable equilibrium.
The correct Answer is:C
Topper's Solved these Questions
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - SECTION (II) : (SUBJECTIVE )
33 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - SECTION(II) : MCQ ( SINGLE CORRECT)
47 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - NUMERICAL BASED QUESTIONS
3 Videos
- HEAT AND TEMPERATUREBOOK - FIITJEECHAPTER - HEAT AND TEMPERATUREEXERCISE - NUMERICAL BASES QUESTIONS
1 Videos
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - COMPREHENSION-III
2 Videos
Similar Questions
In figure, the variation of potential energy of a particle of mass m=2kg is represented w.r.t its x-coordinate. The particle moves under the effect of the conservative force along the x-axis. Which of the following statements is incorrect about the particle?
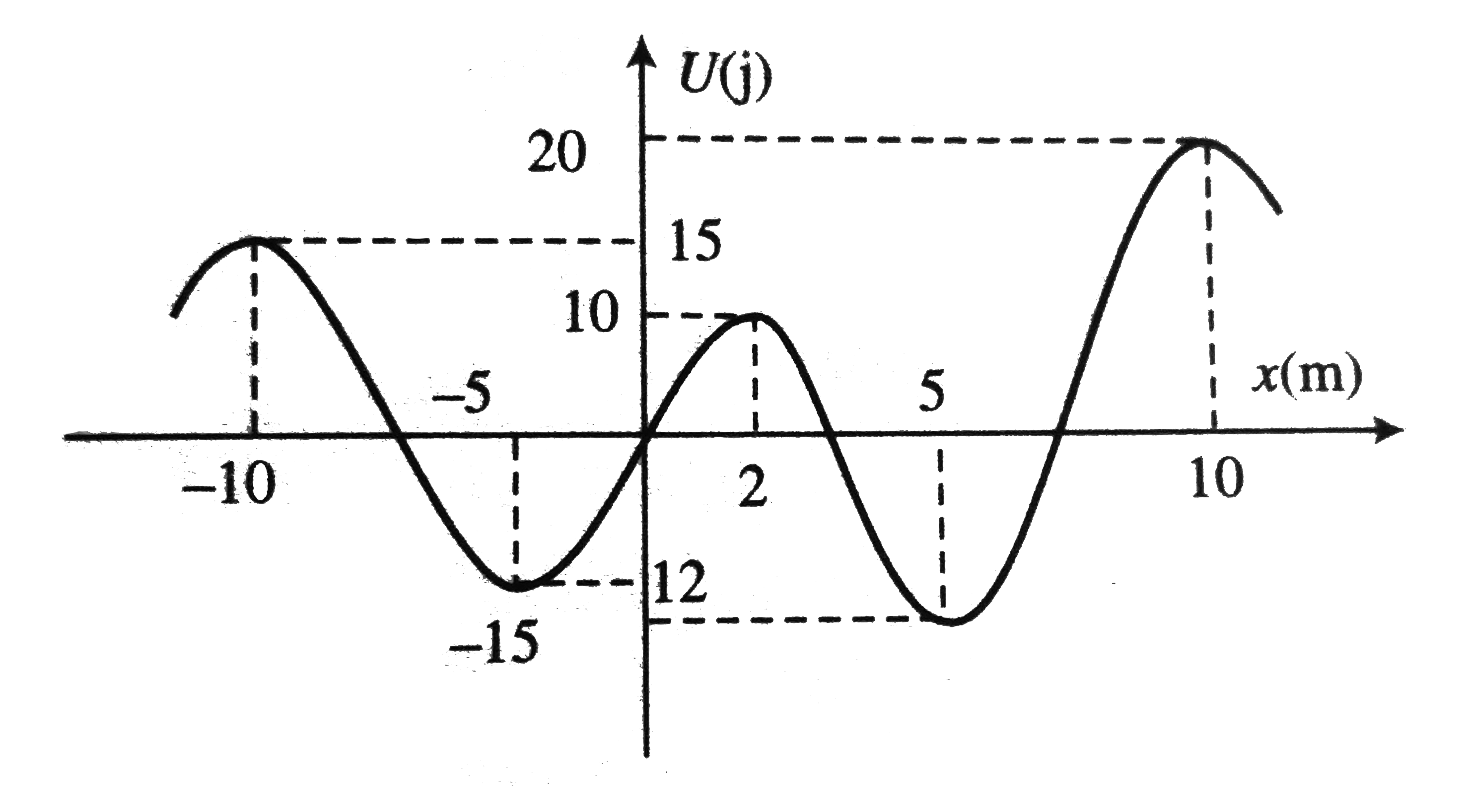
View SolutionIn the figure the variation of potential energy of a particle of mass m=2kg is represented w.r.t. its x-coordinate. The particle moves under the effect of this conservative force along the x-axis.
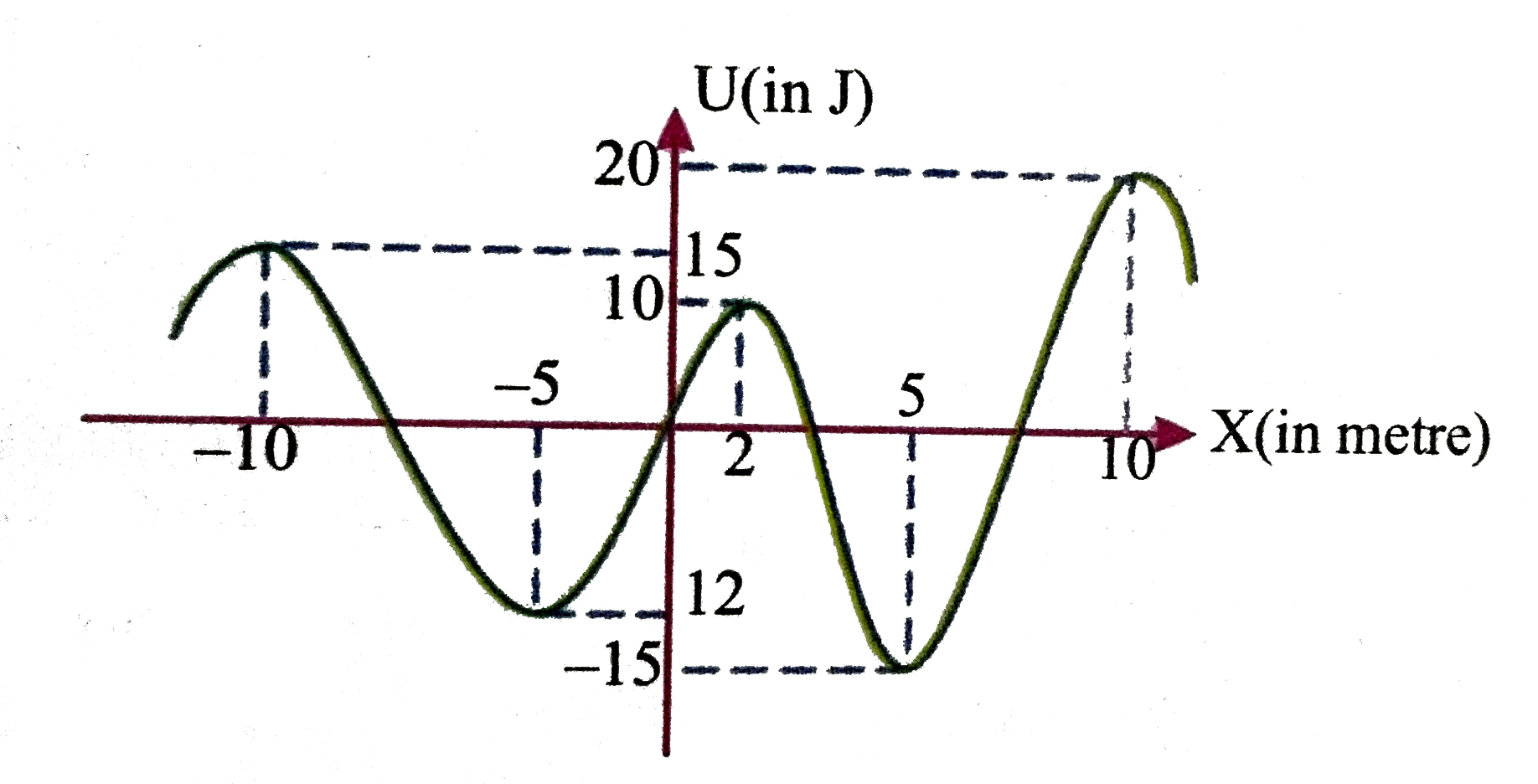
x=−5m and x=10m position of the particle are respectively of
View Solution
Knowledge Check
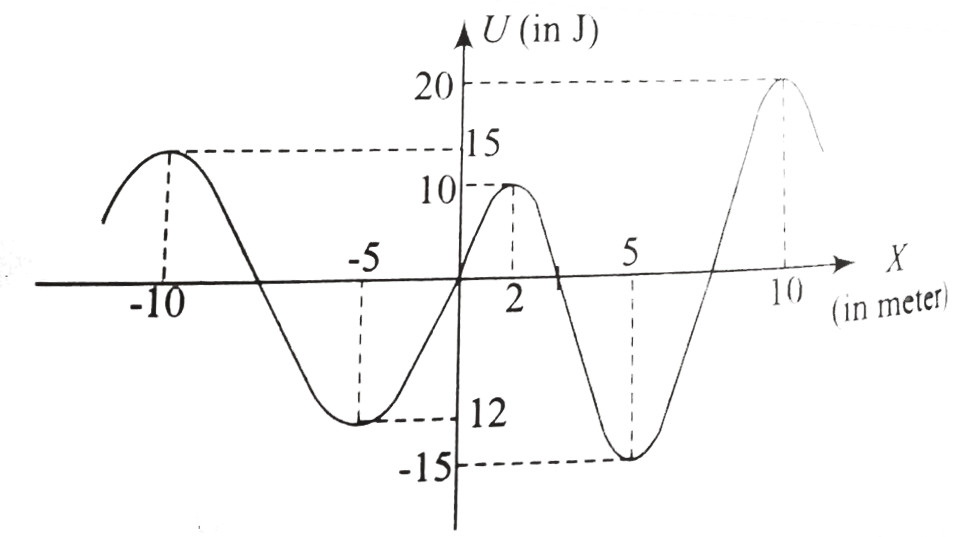
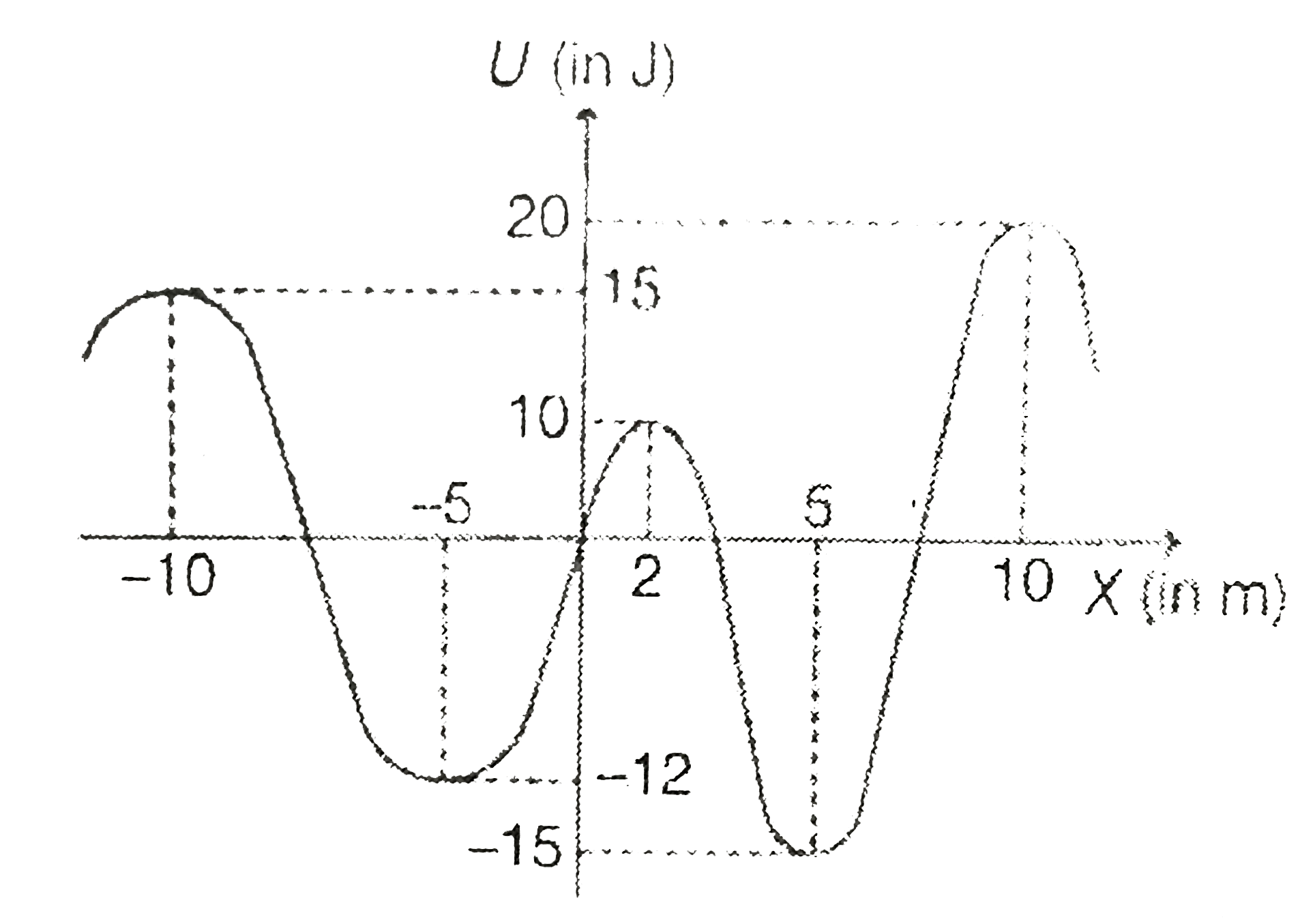
In the figure the variation of potential energy of a particle of mass m=2kg is represented w.r.t. its x-coordinate. The particle moves under the effect of this conservative force along the x-axis. Which of the following statements are correct about the particle:AIf it is released at the origin it will move in negative x-axisBIf it is released at x=2+△ where △→0 then its maximum speed will be 5ms and it will perform oscillatory motionCIf initially x=−10m and →u=√6ˆims then it will cross x=10mDx=−5m and x=+5 m are unstable equilibrium positions of the particle.In the figure the variation of potential energy of a particle of mass m=2kg is represented w.r.t. its x-coordinate. The particle moves under the effect of this conservative force along the x-axis.
If the particle is released at the origin thenAit will move towards positive x-axis.Bit will move towards negative x-axis.Cit will remain stationary at the origin.Dits subsequent motion cannot be decided due to lack of information.In figure, the variation of potential energy of a particle of mass m=2kg is represented w.r.t its x-coordinate. The particle moves under the effect of the conservative force along the x-axis. Which of the following statements is incorrect about the particle?
 A(a) If it is released at the origin, it will move in negative x-axis.B(b) If it is released at x=2+Δ, where Δ→0, then its maximum speed will be 5ms−1 and it will perform oscillatory motion.C(c) If initially x=−10 and →u=√6ˆi, then it will cross x=10.D(d) x=−5 and x=+5 are unstable equilibrium positions of the particle.
A(a) If it is released at the origin, it will move in negative x-axis.B(b) If it is released at x=2+Δ, where Δ→0, then its maximum speed will be 5ms−1 and it will perform oscillatory motion.C(c) If initially x=−10 and →u=√6ˆi, then it will cross x=10.D(d) x=−5 and x=+5 are unstable equilibrium positions of the particle.
In the figure, the variation of potential energy of a particle of mass m = 2kg is represented with respect to its x-coordinate. The particle moves under the effect of this conservative force along the X-axis.
If the particle is released at x=2+Δ where Δ→0 (it is positive), then its maximum speed in subsequent motion will be
View SolutionIn the figure, the variation of potential energy of a particle of mass m = 2kg is represented with respect to its x-coordinate. The particle moves under the effect of this conservative force along the X-axis.
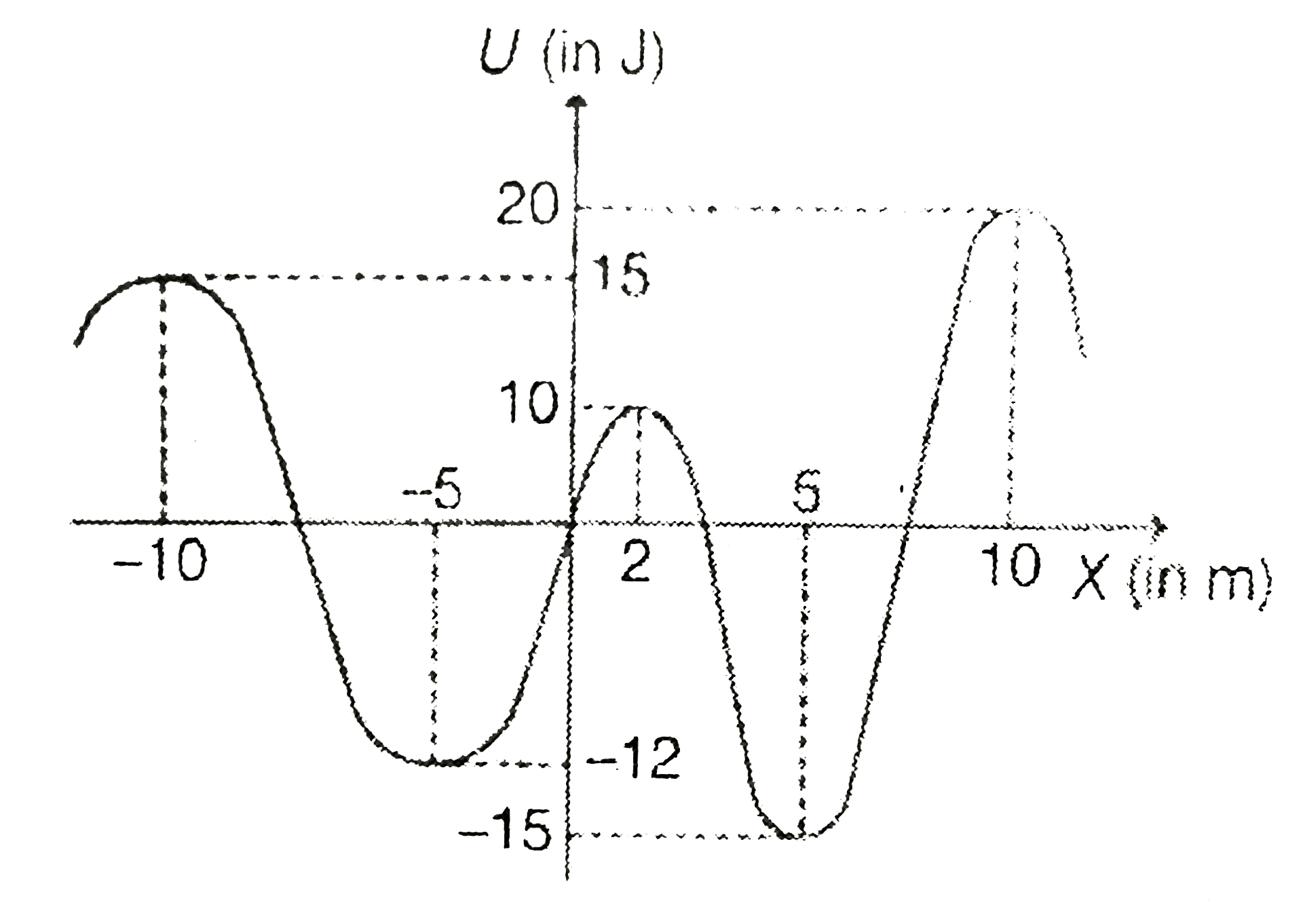
If the particle is released at the origin, then
(A)it will move towards positive x-axis
(B)it will move towards negative x-axis
(C)it will remain stationary at the origin
(D)Either of (a) or (b)
View SolutionIn the figure, the variation of potential energy of a particle of mass m = 2kg is represented with respect to its x-coordinate. The particle moves under the effect of this conservative force along the X-axis.

If the particle is released at the origin, then
View SolutionIn the figure the variation of potential energy of a particle of mass m=2kg is represented w.r.t. its x-coordinate. The particle moves under the effect of this conservative force along the x-axis.

x=−5m and x=10m position of the particle are respectively of
View SolutionIn the figure, the variation of potential energy of a particle of mass m = 2kg is represented with respect to its x-coordinate. The particle moves under the effect of this conservative force along the X-axis.

If the particle is released at x=2+Δ where Δ→0 (it is positive), then its maximum speed in subsequent motion will be
View Solution
