Write a rational function f with a slant asymptote y=x+4, a vertical asymptote at x = 5 and one of the zeros at x = 2.
The graph of f has a slant asymptote y=x+4 and a vertical asymptote at x=5 , hence f(x) may be written as f(x)=(x+4)+a(x−5),
where a is a constant to be determined using the fact that f(2)=0 since f has a zero at x=2.
f(2)=(2+4)+a2−5=0∴a=18
Hence f(x) is given by f(x)=(x+4)+18x−5=x2−x−2x−5
Check the characteristics of the graph of f shown below.
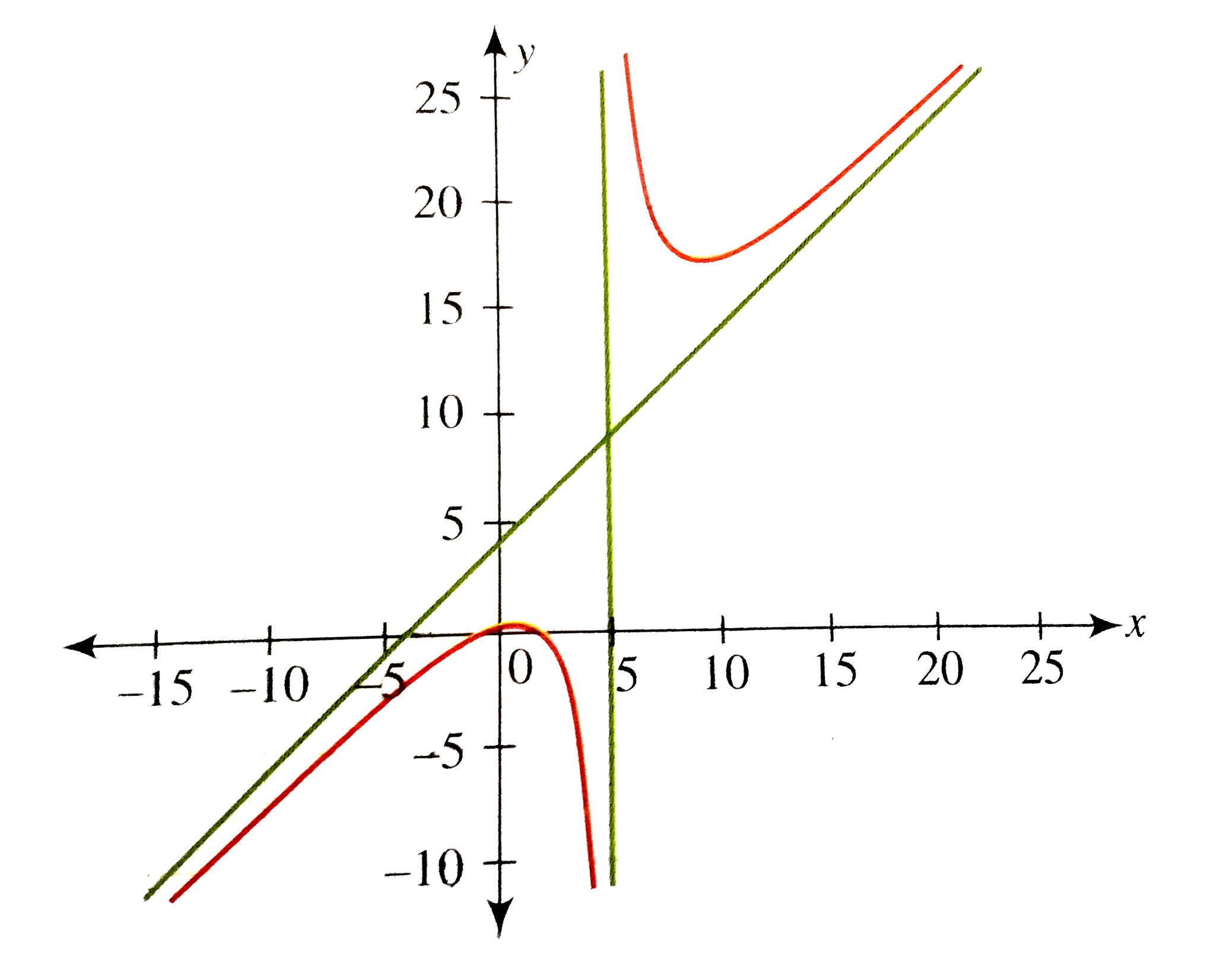
Topper's Solved these Questions
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercise
13 Videos
- GRAPHICAL TRANSFORMATIONSBOOK - CENGAGECHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise
22 Videos
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
Similar Questions
Write a possible rational function h with a hole at x = 5, a vertical asymptote at x = -1, a horizontal asymptote at y = 2 and x-intercept at x = 2.
View SolutionWrite a rational function g with vertical asymptotes at x = 3 and x = -3, a horizontal asymptote at y = -4 and with no x-intercept.
View Solution
Knowledge Check
Equation of asymptotes of xy=7x+5y are
Ax=7, y=5Bx=5, y=7Cxy=35DNone of theseLet f:A→Bf(x)=x+abx2+cx+2, where A represent domain set and B represent range set of function f(x) a,b,c ∈R,f(−1)=0andy=1 is an asymptote of y=f(x)andy=g(x) is the inverse of f(x).
Area bounded between the curves y=f(x)andy=g(x) is:A2√5+ln(3−√55+√5)B2√5+2ln(3+√53−√5)C3√5+4ln(3−√53+√5)D3√5+2ln(3−√53+√5)Let f:A→Bf(x)=x+abx2+cx+2, where A represent domain set and B represent range set of function f(x) a,b,c ∈R,f(−1)=0andy=1 is an asymptote of y=f(x)andy=g(x) is the inverse of f(x).
Area bounded between the curves y=f(x)andy=g(x) is:A2√5+ln(3−√55+√5)B2√5+2ln(3+√53−√5)C3√5+4ln(3−√53+√5)D3√5+2ln(3−√53+√5)
Write a possible rational function f that has a vertical asymptote at x = 2, a horizontal asymptote y = 3 and a zero at x = -5. Also draw the graph of the function.
View SolutionFind all the vertical asymptotes and holes of (x+3)(x−7)(2x−5).
View SolutionFind the slant asymptote of (2x+3)2x+3
View SolutionFind the equation of the hyperbola whose asymptotes are 3x=±5y and the vertices are (±5,0)
View SolutionIf length of perpendicular drawn from points of a curve to a straight line approaches zero along an infinite branch of the curve, the line is said to be an asymptote to the curve. For example , y-axis is an asymptote to y=lnx& x-axis is an asymptote to y=e−x.
If limx→0f(x)=e (a finite number) then y=e is an asymptote to y=f(x) . Similarly if limx→0f(x)=α, then y=α is also an asymptote.
If limx→af(x)=∞ or limx→af(x)=−∞, then x=a is a an asymptote to y=f(x).
Number of asymptotes parallel to co -ordinate aces for the function f(x)=(x+1)(x+2)(x−1)(x−2) is equal to :
View Solution
