Question

Draw the graph of $y={\left|x\right|}^{1/2}$ from $-1\le x\le 1$.

Explanation

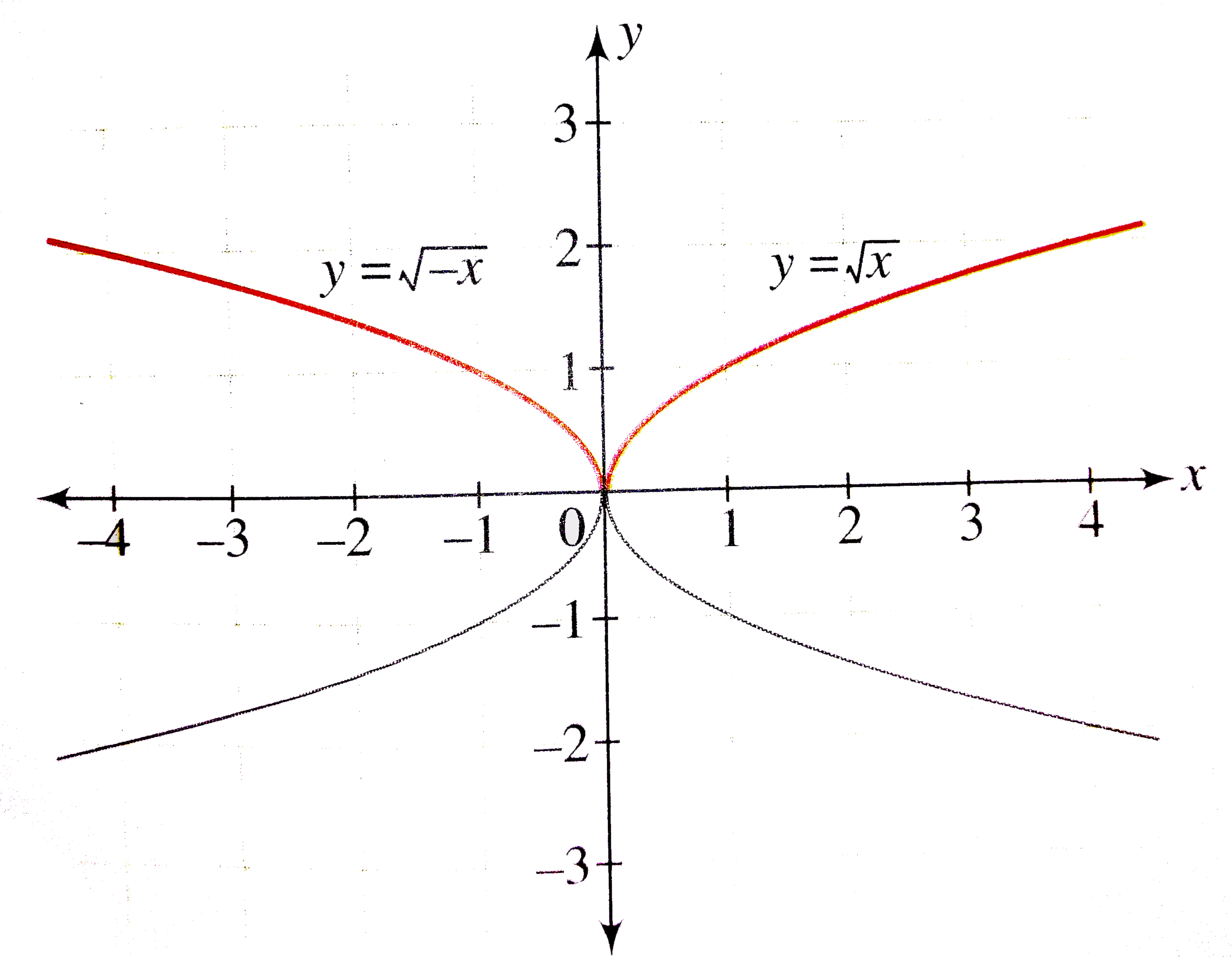
We have `y=f(x) = |x|^(1//2), -1 le x le 1`
`" "={{:( sqrt (-x)",",, x lt 0), (sqrtx",",,x ge 0):}`
`rArr" "y^(2)= -x` if `-1 le x le 0 and y^(2) = x` if `0 le x le 1`
[Here y should be taken always +ve, as by definition, y is a+ve square root.]
Clearly, `y^(2) =-x` represents the upper half of the left-handed parabola (upper half as y is +ve) and `y^(2), = x` represents the upper half of the right-handed parabola.
Thus, the graph of `y=f(x)` is as follows.