If the point $(a, a)$ is placed in between the lines $| x + y | = 4,$ then find the values of $a .$

Video Solution

Text Solution

Verified by Experts

We have lines x+y-4=0 and x+y+4=0
Point (a,a) lies on the line y=x.

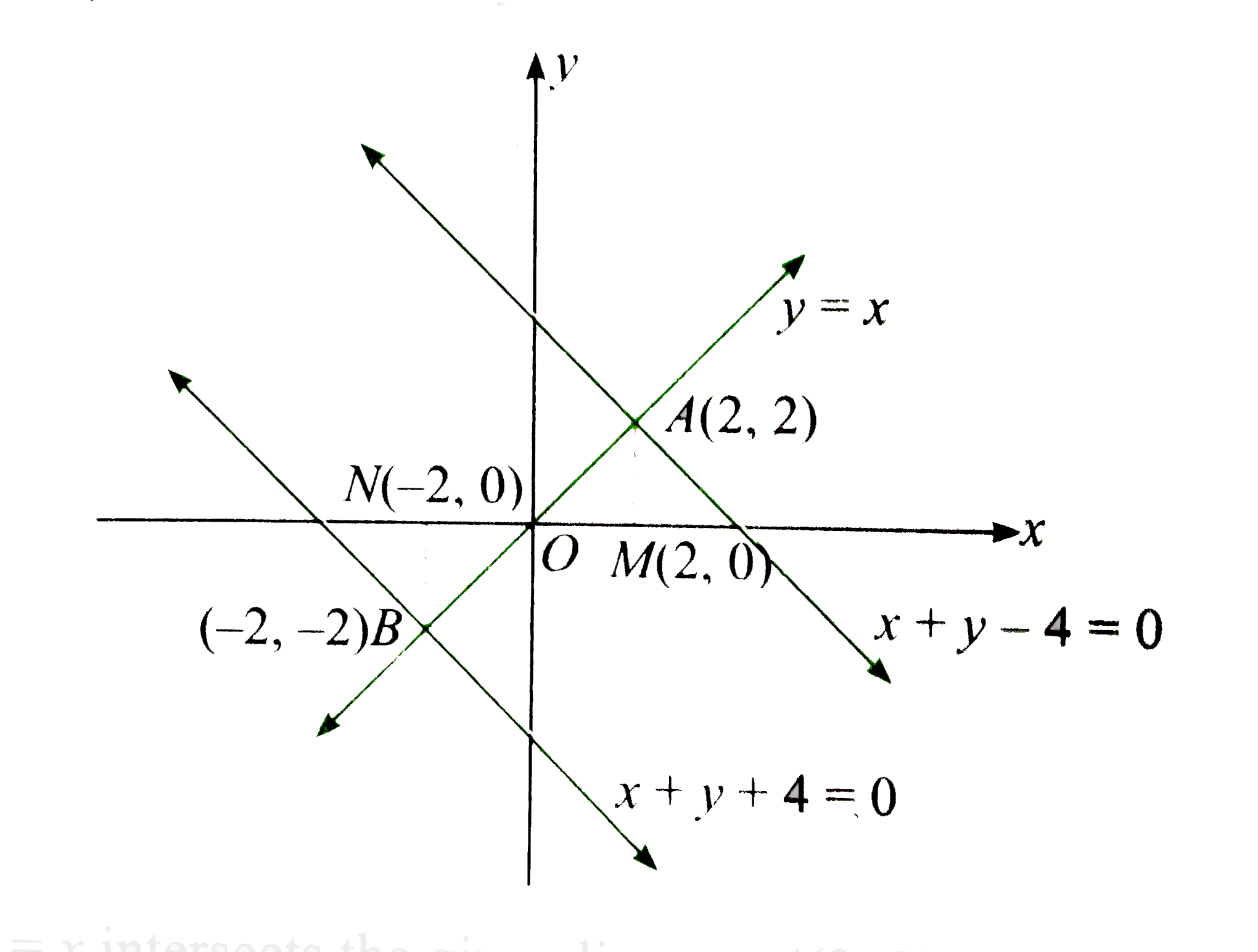
Line y=x intersects the given lines at A(2,2) and B(-2,-2).
So, if (a,a) lies on the line y=x between point A and B, we must have $- 2 < a < 2 .$

Updated on:21/07/2023

|

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.1
23 Videos
STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.2
4 Videos
STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos

Similar Questions

Knowledge Check

If the point $(α, α)$ falls between the lines $| x + y | = 4$ , then
A $| α | = 4$
B $| α | = 2$
C $| α | < 2$
D $| α | < 4$
If the point (a , a) falls between the lines |x + y| = 2. then .
A|a| =2
B|a| =1
C $| a | < 1$
D $| a | < \frac{1}{2}$
If the point (a,a) falls between the line|x+y|=4, then
A $| a | = 2$
B $| a | = 3$
C $| a | < 2$
D $| a | < 3$

CENGAGE-STRAIGHT LINES-JEE Advanced Previous Year

Home
Ask Doubt
Profile