The vertex A of $Δ A B C$ is (3,-1). The equation of median BE and angle bisector CF are x-4y+10=0 and 6x+10y-59=0, respectively. Equation of AC is

A

5x+18y=37

B

15x+8y=37

C

15x-8y=37

D

15x+8y+37=0

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

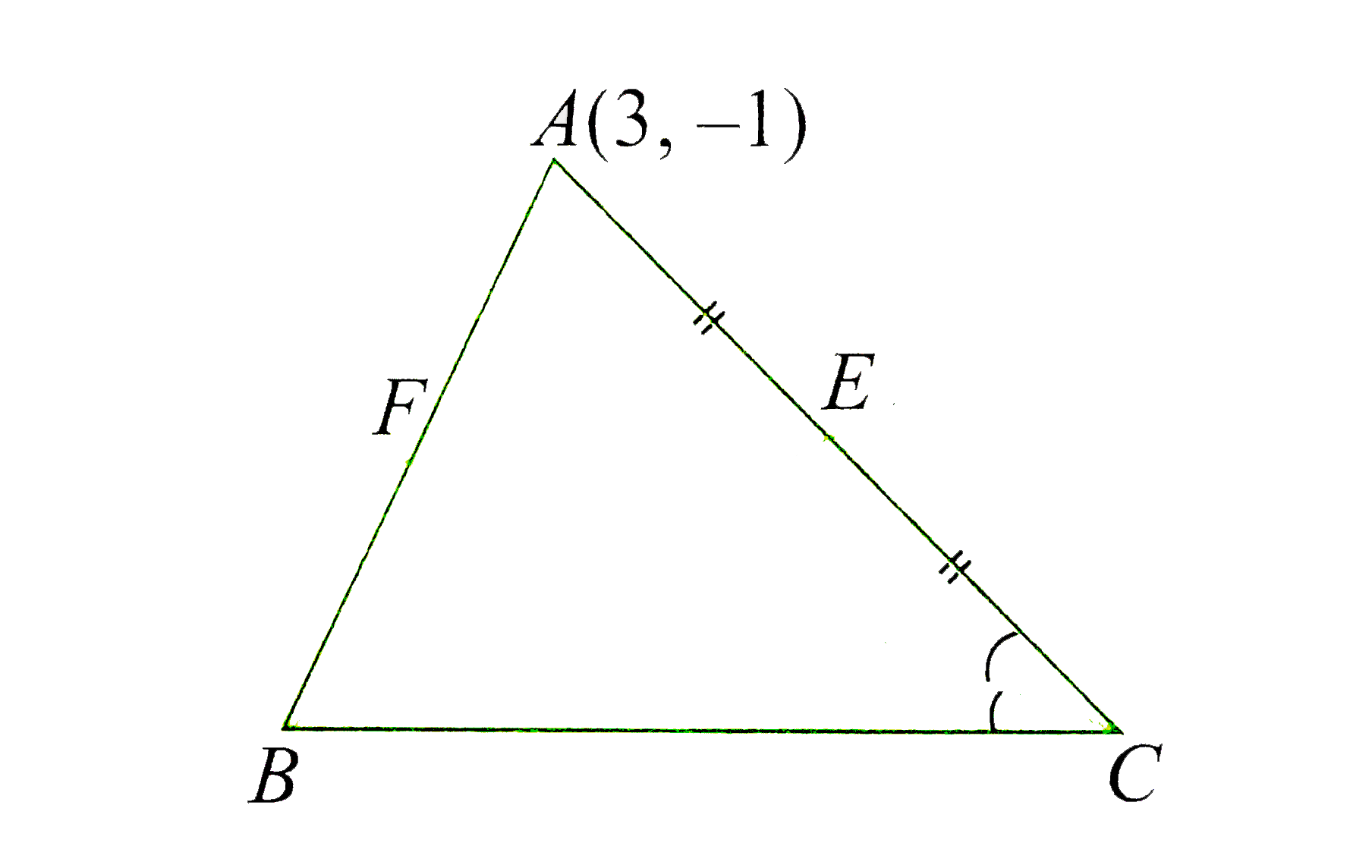
Point E is on the median x-4y+10=0.
$∴ E \equiv (x_{1}, \frac{x_{1} + 10}{4})$
Point C is on the bisector 6x+10y-59=0.
$∴ C \equiv (x_{2}, \frac{59 - 6 x_{2}}{10})$
Midpoint of AC is E.
$∴ \frac{x_{2} + 3}{2} = x_{1}$
$\Rightarrow x_{2} = 2 x_{1} - 3 (1)$
$and \frac{\frac{59 - 6 x_{2}}{10} - 1}{2} = \frac{x_{1} + 10}{4}$
$\Rightarrow \frac{49 - 6 (2 x_{1} - 3)}{20} = \frac{x_{1} + 10}{4}$
$\Rightarrow 49 - 12 x_{1} + 18 = 5 x_{1} + 50$
$\Rightarrow 17 x_{1} = 17$
$∴ x_{1} = 1$
Coordinates of E are $(1, \frac{11}{4})$ .
Equation of AC is
$(y + 1) = \frac{\frac{11}{4} + 1}{- 2} = (x - 3)$
$\Rightarrow - 8 y - 8 = 15 x - 45$
$\Rightarrow 15 x + 8 y = 37$

|

Updated on:21/07/2023

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In $Δ A B C$ equation of the right bisectors of the sides Ab and AC are $x + y = 0 and x - y = 0$ respectively .If A = (5,7) then equation of side BC is
A $7 y = 5 x$
B $5 x = y$
C $5 y = 7 x$
D $5 y = x$
Question 2 - Select One
Let A, B and C be the vertices of a triangle, equation of perpendicular bisectors of AB and AC are $x - y + 3 = 0$ and $x + 2 y + 12 = 0$ respectively If co-ordinates of A are (2,3), then equation of BC is
A $y = 5 x + 3$
B $y = 3 x + 5$
C $5 x + y + 3 = 0$
D $3 x + y + 5 = 0$
Question 3 - Select One
In a triangle ABC , if the equation of sides AB,BC and CA are $2 x - y + 4 = 0, x - 2 y - 1 = 0 and x + 3 y - 3 = 0$ respectively ,
The equation of external bisector of angle B is
A $x - y - 1 = 0$
B $x - y + 1 = 0$
C $x + y - 5 = 0$
D $x + y + 5 = 0$

CENGAGE-STRAIGHT LINES-Exercise (Single)

Home
Ask Doubt
Profile