If AD, BE and CF are the altitudes of ΔABC whose vertex A is (-4,5). The coordinates of points E and F are (4,1) and (-1,-4), respectively. Equation of BC is

A

3x-4y+28=0

B

4x+3y+28=0

C

3x-4y-28=0

D

x+2y+7=0

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

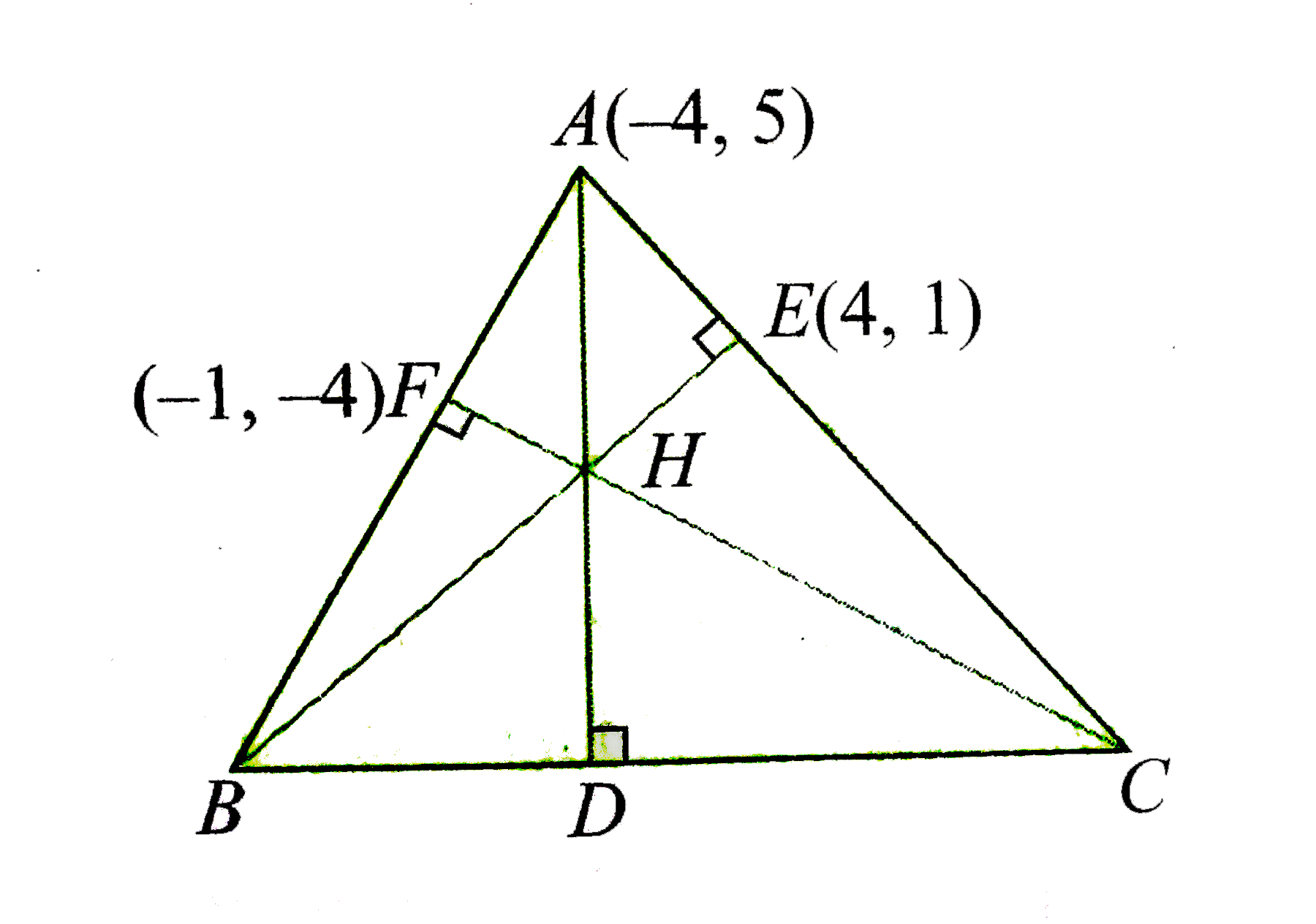
ocr_image
Slope of AB =5−(−4)−4+1=−3
∴ Equation of AB≡3x+y+7=0 (1)
Slope of CF=13
∴ Equation of CF≡x−3y−11=0 (2)
Slope of AC=5−1−4−4=−12
∴ Equation of AC≡x+2y−6=0 (3)
Slope of BE≡2
∴ Equation of BE≡2x−y−7=0 (4)
Solving (1) and (4), we get B≡(0,−7).
Solving (2) and (3), we get C≡(8,−1).
Slope of BC=−7+10−8=34
∴ Equation of BC≡3x−4y−28=0

Updated on:21/07/2023
|
Class 12MATHSSTRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • If AD,BE and CF are the altitudes of a triangle ABC whose vertex A is the point (−4,5). The coordinates of the points E and F are (4,1) and (−1,−4) respectively, then equation of BC is

    A3x−4y−28=0
    B4x+3y−28=0
    C3x−4y+28=0
    Dx+2y+7=0

  • If AD, BE, CF are the altitudes of ΔABC whose orthocentre is H, then C is the orthocentre of :

    A△ABH
    B△BDH
    C△ABD
    D△BEA

  • If A(4, 2), B(6, 5) and C(1, 4) be the vertices of ΔABC and AD is a median, then the coordinates of D are

    A(52,3)
    B(5,72)
    C(72,92)
    Dnone of these