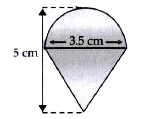
Rasheed got a playing top (lattu) as his birthday present, which surprisingly had no colour on it. He wanted to colour it with his crayons. The top is shaped like a cone surmounted by a hemisphere. The entire top is 5 cm in height and the diameter of the top is 3.5 cm. Find the area he has to colour.
To solve the problem of finding the area Rasheed has to color on his playing top (lattu), which is shaped like a cone surmounted by a hemisphere, we will follow these steps:
Step 1: Understand the dimensions
- The total height of the top (lattu) is given as 5 cm.
- The diameter of the top is given as 3.5 cm, so the radius (r) is half of the diameter:
r=3.5 cm2=1.75 cm
Step 2: Calculate the height of the cone
- The height of the hemisphere is equal to its radius, which is 1.75 cm.
- Therefore, the height of the conical part (h) can be calculated as:
h=Total height−Height of hemisphere=5 cm−1.75 cm=3.25 cm
Step 3: Calculate the slant height of the cone
- The slant height (l) of the cone can be calculated using the Pythagorean theorem:
l=√h2+r2
Substituting the values:
l=√(3.25 cm)2+(1.75 cm)2
l=√10.5625+3.0625=√13.625≈3.69 cm
Step 4: Calculate the surface area of the cone
- The lateral surface area (A_cone) of the cone is given by the formula:
Acone=πrl
Substituting the values:
Acone=π×1.75 cm×3.69 cm
Acone≈3.14×1.75×3.69≈20.39 cm2
Step 5: Calculate the surface area of the hemisphere
- The surface area (A_hemisphere) of the hemisphere is given by the formula:
Ahemisphere=2πr2
Substituting the values:
Ahemisphere=2π(1.75 cm)2
Ahemisphere=2π×3.0625 cm2≈2×3.14×3.0625≈19.13 cm2
Step 6: Calculate the total area to be colored
- The total area (A_total) to be colored is the sum of the areas of the cone and the hemisphere:
Atotal=Acone+Ahemisphere
Atotal≈20.39 cm2+19.13 cm2≈39.52 cm2
Final Answer
Thus, the area Rasheed has to color is approximately:
Area to be colored≈39.52 cm2
- The total height of the top (lattu) is given as 5 cm.
- The diameter of the top is given as 3.5 cm, so the radius (r) is half of the diameter:
r=3.5 cm2=1.75 cm
- The height of the hemisphere is equal to its radius, which is 1.75 cm.
- Therefore, the height of the conical part (h) can be calculated as:
h=Total height−Height of hemisphere=5 cm−1.75 cm=3.25 cm
- The slant height (l) of the cone can be calculated using the Pythagorean theorem:
l=√h2+r2
Substituting the values:
l=√(3.25 cm)2+(1.75 cm)2
l=√10.5625+3.0625=√13.625≈3.69 cm
- The lateral surface area (A_cone) of the cone is given by the formula:
Acone=πrl
Substituting the values:
Acone=π×1.75 cm×3.69 cm
Acone≈3.14×1.75×3.69≈20.39 cm2
- The surface area (A_hemisphere) of the hemisphere is given by the formula:
Ahemisphere=2πr2
Substituting the values:
Ahemisphere=2π(1.75 cm)2
Ahemisphere=2π×3.0625 cm2≈2×3.14×3.0625≈19.13 cm2
- The total area (A_total) to be colored is the sum of the areas of the cone and the hemisphere:
Atotal=Acone+Ahemisphere
Atotal≈20.39 cm2+19.13 cm2≈39.52 cm2
Thus, the area Rasheed has to color is approximately:
Area to be colored≈39.52 cm2
Topper's Solved these Questions
- SURFACE AREAS AND VOLUMESBOOK - NCERTCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - EXERCISE 13.5
7 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERTCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - EXERCISE 13.2
8 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERTCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Exercise 13.3
9 Videos
- STATISTICSBOOK - NCERTCHAPTER - STATISTICSEXERCISE - EXERCISE 14.1
9 Videos
- TRIANGLESBOOK - NCERTCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLES
14 Videos
Similar Questions
Rasheed got a playing top (lattu) as his birthday present, which surprisingly had no colour on it. He wanted to colour it with his crayons. The top is shaped like a cone surmounted by a hemisphere. The entire top is 5 cm in height and the diameter of the top is 3.5 cm. Find the area he has to colour.
View SolutionRasheed got a playing top (lattu) as his birthday present,which surprisingly had no colour on it. He wanted to colour it with his crayons. The top is shaped like a cone surmounted by a hemisphere (see figure).

The entire top is 5 cm in height and the diameter of the top is 3.5 cm. Find the area he has to colour. (takeπ = 22/7)
View Solution
Knowledge Check
A toy is in the form of a cone surmounted by a hemisphere whose radius is 3.5cm . If the total height of the toy is 15.5cm then find its total surface area and the Volume
A214.5cm2and240cm3B240cm2and214.5cm3C214.5cm2and243.83cm3D243.83cm2and214.5cm3
Rasheed got a playing top (lattu) as his birthday present, which surprisingly had no colour on it. He wanted to colour it with his crayons. The top is shaped like a cone surmounted by a hemisphere (see figure). The entire top is 5 cm in height and the diameter of the to is 3.5 cm. What will be the radius of hemispherical portion having diameter 3.5cm ?
View SolutionA manufacturer involved ten children in colouring playing top (lattu) which is shaped like a cone surmounted by a hemisphere. The entire top is 5 cm in height and the diameter or of top is 3.5 cm. Find the are they had to paint if 50 playing tops were given to them . (Take π=227 )
(a) How is child labour an abuse for the society ?
(b) What steps can be taken to abolish child labour ?
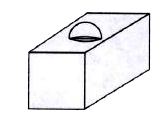
View SolutionThe decorative block is made of two solids — a cube and a hemisphere. The base of the block is a cube with edge 5 cm, and the hemispherefixed on the top has a diameter of 4.2 cm. Find the total surface area of the block.
View SolutionThe base of the block is a cube of side 6 cm and the hemisphere fixed on the top has a diameter of 3.5 cm. Find the total surface area of the block. (use π=227)
View SolutionThe area of top face of a book, in the shape of a square, is 225 cm2. The thickness of the book is 5 cm. Find its volume.
View SolutionA shuttle cock used for playing badminton has the shape of a frustum of a cone mounted on a hemisphere. The diameters of the ends of the frustum are 5 cm and 2 cm; the height of the entire shuttle cock is 7 cm. Find the external surface area.
View SolutionThe area of top face of a book, in the shape of a square, is 225 cm2. The thickness of the book is 5 cm. Find its volume.
View Solution
