Let ABCD be a rectangle and P be any point in its plane. Show that AP2+PC2=PB2+PD2.
To show that AP2+PC2=PB2+PD2 for a rectangle ABCD and a point P in its plane, we will use coordinate geometry. Let's assume the rectangle ABCD is positioned in the Cartesian plane as follows:
- A(0,0)
- B(a,0)
- C(a,b)
- D(0,b)
Let P be any point in the plane with coordinates P(x,y).
Step 1: Calculate AP2
The distance AP can be calculated using the distance formula:
AP2=(x−0)2+(y−0)2=x2+y2
Step 2: Calculate PC2
Next, we calculate the distance PC:
PC2=(x−a)2+(y−b)2
Expanding this gives:
PC2=(x2−2ax+a2)+(y2−2by+b2)=x2+y2−2ax−2by+a2+b2
Step 3: Calculate PB2
Now, we calculate the distance PB:
PB2=(x−a)2+(y−0)2=(x−a)2+y2
Expanding this gives:
PB2=(x2−2ax+a2)+y2=x2+y2−2ax+a2
Step 4: Calculate PD2
Lastly, we calculate the distance PD:
PD2=(x−0)2+(y−b)2=x2+(y−b)2
Expanding this gives:
PD2=x2+(y2−2by+b2)=x2+y2−2by+b2
Step 5: Combine PB2 and PD2
Now, we combine PB2 and PD2:
PB2+PD2=(x2+y2−2ax+a2)+(x2+y2−2by+b2)
This simplifies to:
PB2+PD2=2x2+2y2−2ax−2by+a2+b2
Step 6: Combine AP2 and PC2
Now, we combine AP2 and PC2:
AP2+PC2=(x2+y2)+(x2+y2−2ax−2by+a2+b2)
This simplifies to:
AP2+PC2=2x2+2y2−2ax−2by+a2+b2
Conclusion
Since both AP2+PC2 and PB2+PD2 simplify to the same expression, we have shown that:
AP2+PC2=PB2+PD2
Thus, the statement is proved.
- B(a,0)
- C(a,b)
- D(0,b)
Similar Questions
Let ABCD be a rectangle and P be any point in its plane. Show that AP2+PC2=PB2+PD2.
View SolutionIn Figure, ABCD and PQRC are rectangles and Q is the mid-point of AC . Prove that DP=PC (ii) PR=12 AC
View SolutionThe coordinates of A,B,C are (6,3),(−3,5),(4,−2) , respectively, and P is any point (x,y) . Show that the ratio of the area of PBC to that of ABC is |x+y−2|7.
View SolutionIn ABC,D is the mid-point of AB,P is any point of BC.CQPD meets AB in Q . Show that ar(BPQ)=12ar(ABC). TO PROVE : ar(BPQ)=12ar(ABC) CONSTRUCTION : Join CD.
View SolutionLet a,b,c,d and p be any non zero distinct real numbers such that (a2+b2+c2)p2−2(ab+bc+cd)p+(b2+c2+d2)=0. Then :
View SolutionIf the angles A,B,C of △ABC are in A.P., and its sides a, b, c are in Gp., show that a2,b2,c2 are in A.P.
View SolutionABCD is a parallelogram. P is a point on AD such that AP=13 AD and Q is a point on BC such that: CQ=13BC. Prove that AQCP is a parallelogram.
View SolutionIn Figure, P is a point in the interior of a parallelogram ABCD . Show that ar(APB)+ar(PCD)=12ar(^(gm)ABCD) aR(APD)+ar(PBC)=ar(APB)+ar(PCD)
View Solutionp AND q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar(APB)=ar (BQC).
View SolutionIn Fig. 9.32, ABCD is a parallelogram and BC is produced to a point Q such thatAD = CQ. If AQ intersect DC at P, show that ar (BPC) = ar (DPQ).
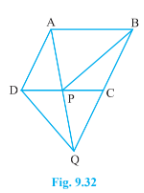
View Solution
