Prove that the image of point P(cosθ,sinθ) in the line having slope tan(α/2) and passing through origin is Q(cos(α−θ),sin(α−θ)).
Text Solution
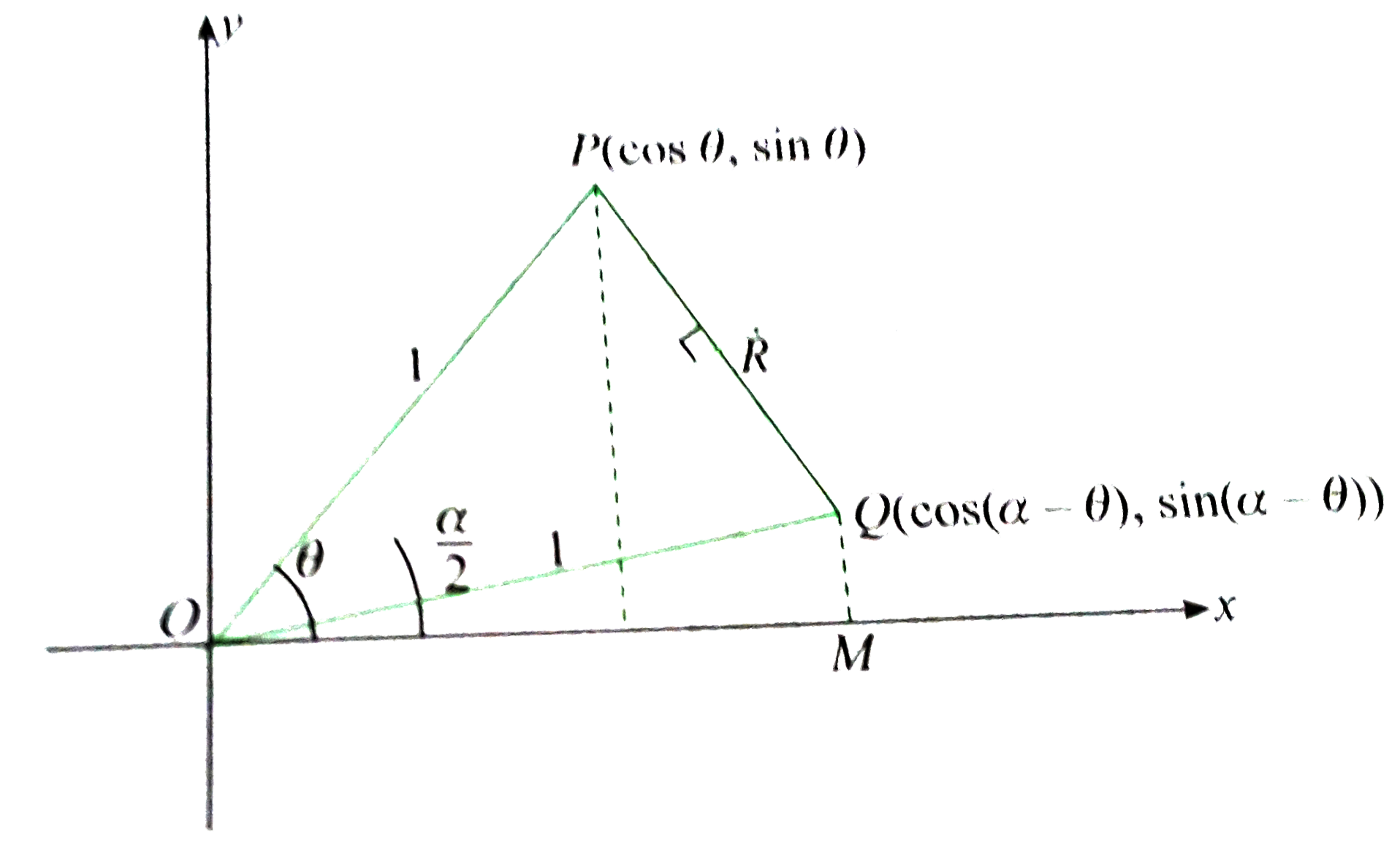
Clearly, OP=OQ=1
Now, we have to prove that Q is the image of P in the line OR which has slope tan (α/2).
Triangle POQ is isosceles triangle.
If Q is the image of P in line OR, then OR is the perpendicular bisector of PQ.
We have to prove that ∠QOM=α−θ.
∠ROQ=∠POR=θ−(α/2)
∴∠QOM=∠ROM−∠ROQ
=(α/2)−(θ−(α))=α−θ
|
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.1
6 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.2
8 Videos
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGECHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Knowledge Check
If 3cosθ+4sinθ=Asin(θ+α), then values of A and α are
A5⋅cos−1(35)B5,sin−1(35)C7,sin−1(45)DNone of theseIf sinθ=3sin(θ+2α), then the value of tan(θ+α)+2tanα is
A3B2C−1D0
sin(3θ+α)+sin(3θ−α)+sin(α−θ)−sin(α+θ)=cosα
View SolutionIf cos(θ−α),cosθ,cos(θ+α) are in H.P. then cosθ.sec(α2)=
View Solutionsin(3θ+α)+sin(3θ−α)+sin(α−θ)−sin(α+θ)=cosα
View SolutionIf tanθ=cosα−sinαcosα+sinα and θ is acute then θ+α =
View SolutionProve that : 2sin2θ+4cos(θ+α)sinαsinθ+cos2(α+θ) is independent of θ.
View SolutionIf tanα=sinθ−cosθsinθ+cosθ then sinα
View Solution
