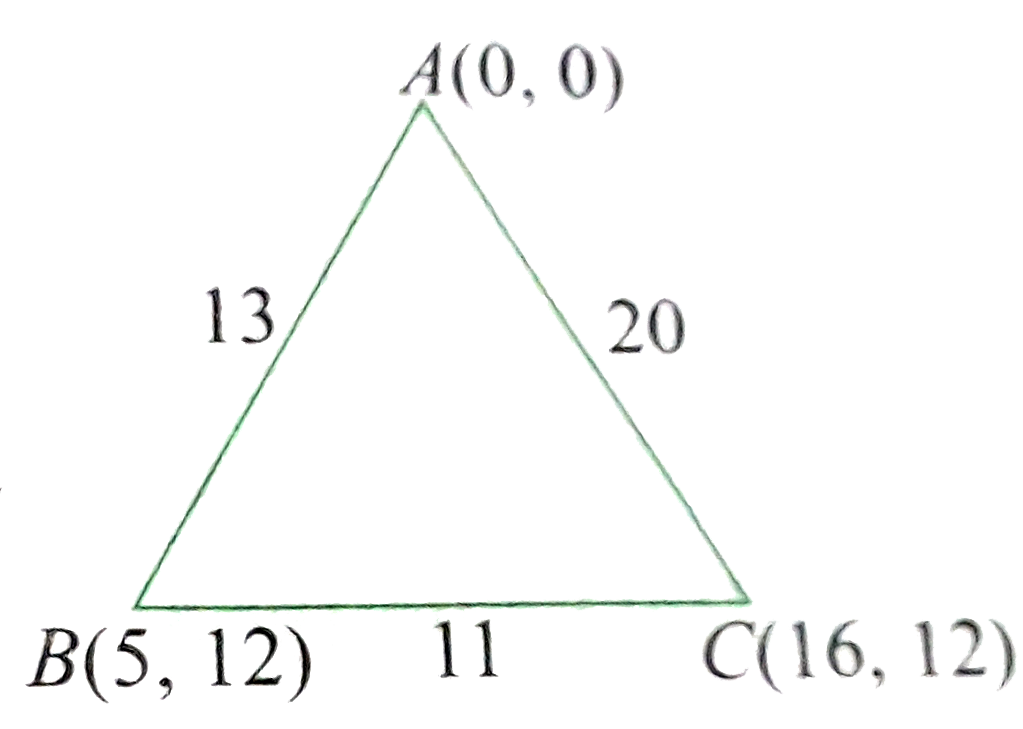
Consider the triangle whose vetices are (0,0) , (5,12) and (16,12).

Text Solution

Verified by Experts

The correct Answer is:a to s;b to p;c to q;d to s

(a). Centroid is $(\frac{0 + 5 + 16}{3}, \frac{0 + 12 + 12}{3}) \equiv (7, 8)$
(b). Let the coordinates of circumcenter be $O (x, y)$ therefore,
$O A = O B = O C$
$∴ x^{2} + y^{2} = {(x - 5)}^{2} + {(y - 12)}^{2} = {(x - 16)}^{2} + {(y - 12)}^{2}$
$∴ x^{2} + y^{2} = {(x - 5)}^{2} + {(y - 12)}^{2}$
or $10 x + 24 y = 169$
and ${(x - 5)}^{2} + {(y - 12)}^{2} = {(x - 16)}^{2} + {(y - 12)}^{2}$
or $2 x = 21$
Solving, we get
$x = \frac{21}{2}, y = \frac{8}{3}$
(c). $I \equiv (\frac{0 \times 11 + 5 \times 20 + 16 \times 13}{13 + 20 + 11}, \frac{0 \times 11 + 12 \times 20 + 13 \times 12}{13 + 20 + 11}) \equiv (7, 9)$
(d). $I_{2} \equiv (\frac{- 5 \times 20 + 13 \times 16 + 11 \times 0}{- 20 + 13 + 11}, \frac{- 12 \times 20 + 0 \times 11 + 13 \times 12}{- 20 + 13 + 11}) \equiv (27, - 21)$ .

|

Updated on:21/07/2023

Class 12 MATHS THREE DIMENSIONAL GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The orthocentre of the triangle whose vertices are (5,-2),(-1,2) and (1,4) is
A(1/5,14/5)
B(14/5,1/5)
C(1/5,1/5)
D(14/5,14/5)

CENGAGE-COORDINATE SYSYEM -Exercise (Matrix)

Home
Ask Doubt
Profile