The locus of the midpoint of a line segment that is drawn from a given external point P to a given circle with center O (where O is the orgin) and radius r is a straight line perpendiculat to PO a circle with center P and radius r a circle with center P and radius 2r a circle with center at the midpoint PO and radius r2.
Step by step video & image solution for The locus of the midpoint of a line segment that is drawn from a given external point P to a given circle with center O (where O is the orgin) and radius r is a straight line perpendiculat to P O a circle with center P and radius r a circle with center P and radius 2r a circle with center at the midpoint P O and radius r/2dot by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If the angle between two tangents drawn from an external point P to a circle of radius a and center O , is 60∘, then find the length of OP.
A2aB4aC6aD8a
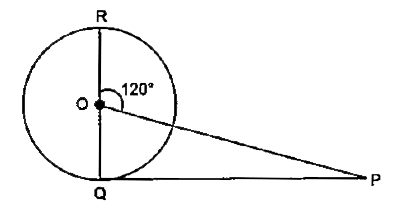
PQ is a tangent drawn from an external point P to a circle with center O, ∠QOR is the diameter of the circle. If ∠POR=120∘. What is the measure of ∠OPQ?
View SolutionIf angle between two tangents drawn from a point P to a circle of radius 'a' and the centre O is 90∘ then prove that OP=√a
View SolutionInterior the part of the plane consisting of those points P for which OP<r is called the interior of the circle with centre O and radius r.
View SolutionFrom an external point two tangents PA and PB are drawn to the circle with center O. Prove that OP is perpendicular bisector of AB.
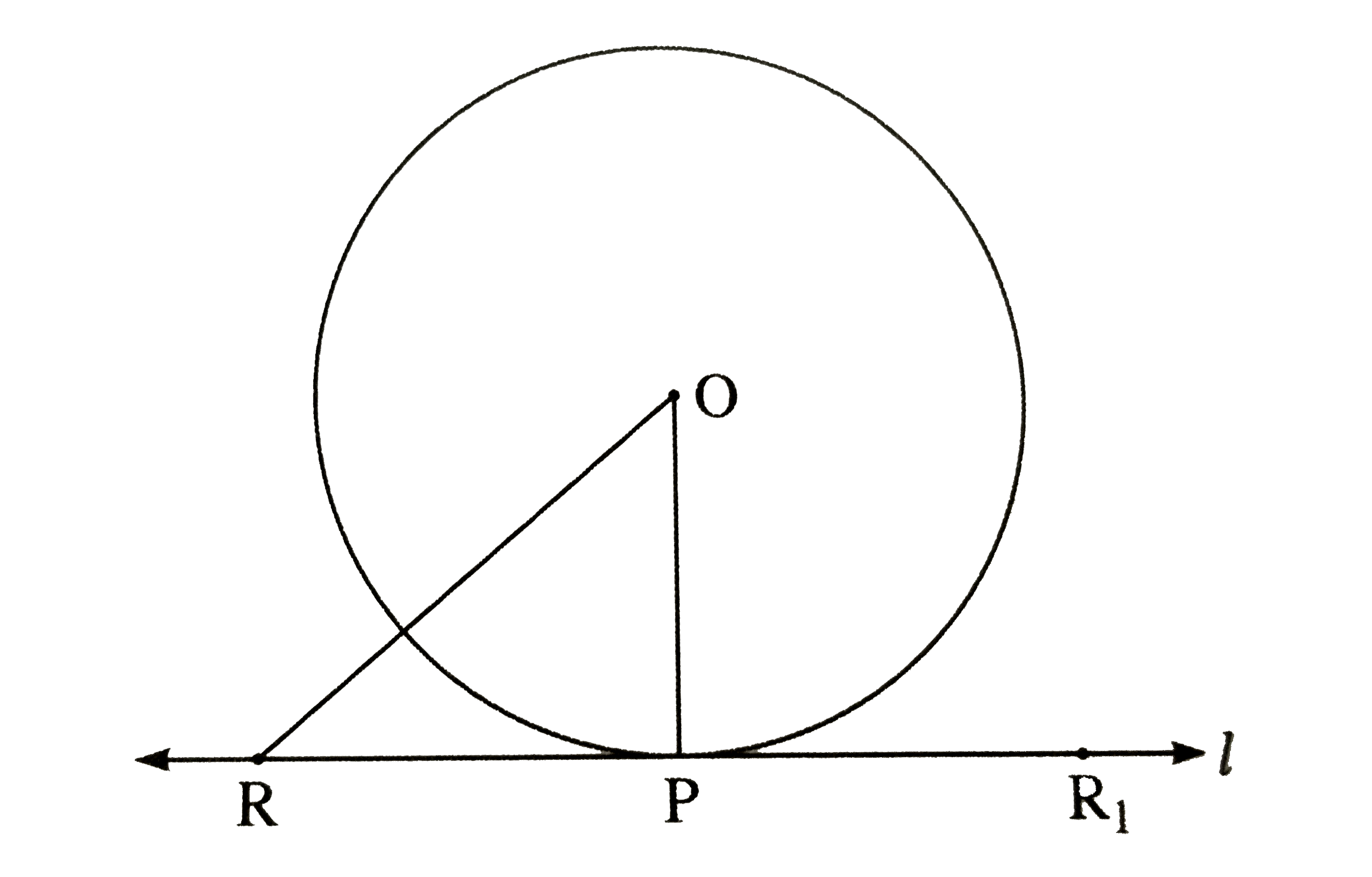
View SolutionLine l touches a circle with centre O at point P. If radius of the circle is 9 cm, answer the following:
What is d(O,P)=? Why?
View SolutionIf |z|<1,then1+2z lies (A) on or inside circle having center at origin and radius 2 (B) outside the circle having center at origin and radius 2 (C) inside the circle having center at (1,0) and radius 2 (D) outside the circle having center at (1,0) and radius 2.
View SolutionThe line join of the points (2,3) and (−1,5) subtends a right angle at P. If the locus of P is a circle then its radius is
View Solution
