A particle moves in the x-y plane under the action of a force,
F=K⎡⎢⎣x(x2+y2)32ˆi+y(x2+y2)32ˆj⎤⎥⎦ where, K is a
constant. Work done by the force when the particel moves from (0,a) to (a, 0) along a circular path of radius a about the origin is Solution in Telugu
2Kπa
Kπa
Kπ2a
0
The correct Answer is:D
According to the equestion,

Force on a particle moves in the x -y plane,
F=k[x(x2+y2)3/2ˆi+y(x2+y2)3/2ˆj]
Now , putting , x = r cos θ, y = r sin θ [ ∵ From figure, ]
F = k ⎡⎢⎣rcosθ(r2cos2θ+r2sin2θ)3/2ˆi+rsinθ(r2cos2θ+r2sin2θ)3/2ˆj⎤⎥⎦
∴ sin2θ+cos2θ=1
F = k [rr3(cosθˆi+sinθˆj)]
F = kr2[cosθˆi+sinθˆj]
∴ Work done , w = F × s (∵s=0 , for circle )
Now, we can say that the direction of force is along the radius of circle, Hence, the work done by this force will be zero.
Similar Questions
A force vec(F)=(-y hat(i)+ x hat(j))N acts on a particle as it moves in an anticlockwise circular motion in x-y plane. The centre of the circle is at the origin. If the work done by the force is 32 pi J in one complete revolution then asSigmaing x, y to be in meters, find the radius of the path.
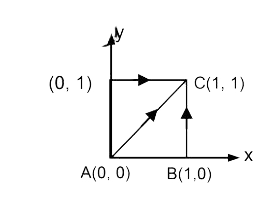
View SolutionA particle of mass m moves from A to C under the action of force →F=2xyˆi+y2ˆj , along different paths as shown in figure.
 08:41View Solution
08:41View SolutionThe work done by a force F=2(x+4y)hat i+8xhat j N on a particle moving from origin to (4m,2m,0) along the path x^(2)=8y is 10n .Find the value of n
04:37View Solutionबल →F=x2ˆi+y2ˆj के द्वारा चित्र में दिखाए गए पथ कर किया गया कार्य होगा-
 03:30View Solution
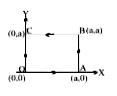
03:30View Solutionएक बल, K[x(x2+y2)3/2ˆi+y(x2+y2)3/2ˆj] (K एक उचित विमा का स्थिरांक है) m द्रव्यमान के द्वारा एक कण को (a,0) बिंदु से (0,a ) बिंदु तक a त्रिज्या के एक वृत्तीय पथ पर ले जाता है, जिसका केंद्र x-y तल का मूल बिंदु है । इस बल द्वारा किया गया कार्य निम्न है:
06:41View SolutionA body of mass 1kg begins to move under the action of a time dependent force →F=(2tˆi+3t2ˆj)N, where ˆi and ˆj are unit vectors along x-and y-axes. What power will be developed by the force at the time t ?
04:31View SolutionThe force vec(F)=A(y^(2)hat(i)+2x^(2)hat(j)) The work done in the path shown by A, where A is a constant and x and y are in meters:
05:04View Solution

