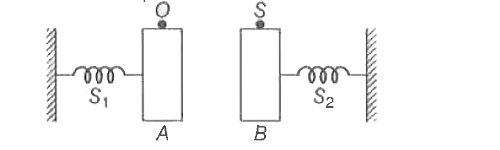
A source S emitting sound of frequency 288 Hz is fixed on block B which is attached to the free end of a spring S2 and an observer O is on block A which is attached to the free end of spring S1 as shown in the figure. The blocks A and B are simultaneously displaced towards each other through a distance of 0.5 m and then left to oscillate. If the angular velocity of each blocks is 40rads−1, then the maximum frequency observed by the observer is (speed of sound in air is 340 ms−1)
 Solution in Telugu
Solution in Telugu
288 Hz
310 Hz
324 Hz
256 Hz
The correct Answer is:C
Frequency is maximum, when both O and .S. are moving towards each other with maximum speeds.
Now, maximum speed of O = maximum speed of S
[and these two occur together].
=vm=aω=0.5×40=20ms−1
So, maximum frequency
= fmax=f(vsound+vobservervsound−vobserver)=280×(340+20340−20)
= 324 Hz
Topper's Solved these Questions
Similar Questions
A nucleus of deuterons or deuterium is a bound atomic system best described by
View SolutionThe pressure on a circular plate is measured by measuring the force on the plate and the radius of the plate. If the errors in measurement of the force and the radius are 5% and 3% respectively, the percentage of error in the measurement of pressure is
View SolutionA source S emitting sound of 300 Hz is fixed of block A which is attached to free end of a spring S_A as shown in the figure. The detector D fixed on block B attached to the free end of spring S_B detects this sound. The blocks A and B are simultaneously displaced towards each other through of 1.0 m and then left to vibrate. Find the maximum and minimum frequencies of sound detected by D if the vibrational frequency of each block is 2 Hz (velocity of sound is 340 m/s).
View SolutionOne end of a spring is rigidly fixed. A block attached to the free end of the spring is pulled through a distance x_(0) . On releasing the block, its amplitude of motion cannot exceed +-x_(0) . Why?
View SolutionA block of mass m is attached to two unstretched springs of spring constant k , each as shown. The block is displaced towards right through a distance x and is released The speed of the block as it passes through the mean position will be
View SolutionA source S emitting sound of 300 Hz is fixed on block A which is attache to free end of a spring S_(A) as shown in figure. The detector D fixed on block B attached to free end of spring S_(B) detects this sound. The blocks A and B simultaneously displaced towards each other thrugh a distance of 2.0m and then left to vibrate. If the product of maximum and minimum freuquencies of sound detected by D is K + 10^(4) sec^(-2) . Find K . Given the vibrational frequencies of each block is 5//pi Hz . speed of sound in air = 300 m//s
View SolutionCalculate the period of oscillation of a block of mass 0.5 kg attached to a spring (spring constant 100Nm^(-1) ) at one end.
View SolutionA source S emitting sound of frequency 300Hz is fixed on block A which is attached to the free end of a spring SA as shown in figure. The detector D fixed on block B attached to free end of spring SB detects this sound. The blocks A and B are simultaneously displaced towards each other through a distance of 1.0m and then left to vibrate. The maximum and minimum frequencies of sound detected by D, if the vibrational frequency of each block is 2Hz are (Velocity of sound v=340m/s)
View SolutionA block weighing 10.0 N is attached to the lower end of a verticle spring (k = 200.0 N//m) , the other end of which is attached to a ceiling. The block oscillates vertically and has a kinetic energy of 2.00 J as it passes through the point at which the spring is unstretched. What is the amplitude of the oscillation and the maximum kinetic energy of the block as it oscillates?
View SolutionA source 'S' emitting a sound of frequency 300 Hz is fixed on block ‘A’ while detector is fixed on block 'B' detects the sound. The block 'A' and 'B' are simultaneously displaced towards each other through a distance of 1 m and then left to vibrate. If the velocity of sound in air is 340ms^(-1) and S_A , S_B are identical the frequency of block A is 2Hz. Then
View SolutionA source .S. emitting sound of 300Hz is fixed on block .A. which is attached to free end on spring SA as shown in the figure. The detector .D. fixed on block .B. attached to the free end of spring SB detects this sound. The blocks .A. and "B. are simulataneously displaced towards eachother through a distance of lm and then left to vibrate. Find the maximum and minimum frequencies of sound detected by .D. if the vibrational frequency of each block is 2Hz (speed of sound 340ms−1)
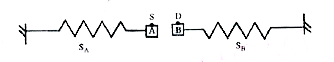
View SolutionA source .S. emitting a sound of frequency 300 Hz is fixed on block .A. while detector is fixed on block .B. detects the sound. The block .A. and .B. are simultaneously displaced towards each other through a distance of 1 m and then left to vibrate. If the velocity of sound in air is 340ms−1 and S , SA,SB are identical the frequency of block A is 2Hz. Then
View SolutionA block of mass m is attached to two unstretched springs of spring constant k , each as shown. The block is displaced towards right through a distance x and is released The speed of the block as it passes through the mean position will be
View Solution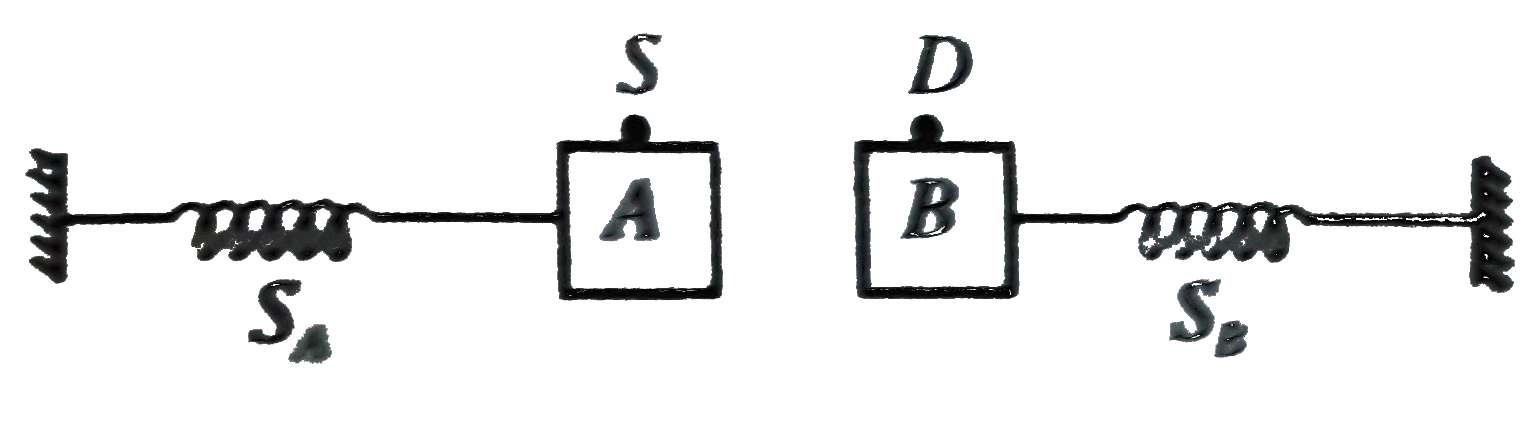
A source S emitting sound of 300 Hz is fixed of block A which is attached to free end of a spring SA as shown in the figure. The detector D fixed on block B attached to the free end of spring SB detects this sound.
The blocks A and B are simultaneously displaced towards each other through of 1.0 m and then left to vibrate. Find the maximum and minimum frequencies of sound detected by D if the vibrational frequency of each block is 2 Hz (velocity of sound is 340 m/s).
View SolutionA source S emitting sound of 300 Hz is fixed on block A which is attache to free end of a spring S_(A) as shown in figure. The detector D fixed on block B attached to free end of spring S_(B) detects this sound. The blocks A and B simultaneously displaced towards each other thrugh a distance of 2.0m and then left to vibrate. If the product of maximum and minimum freuquencies of sound detected by D is K + 10^(4) sec^(-2) . Find K . Given the vibrational frequencies of each block is 5//pi Hz . speed of sound in air = 300 m//s
View Solution
