What should be the minimum value of the refractive index of right angled isosceles prism so that the prism can deviate a ray through 180∘ by total internal reflection? 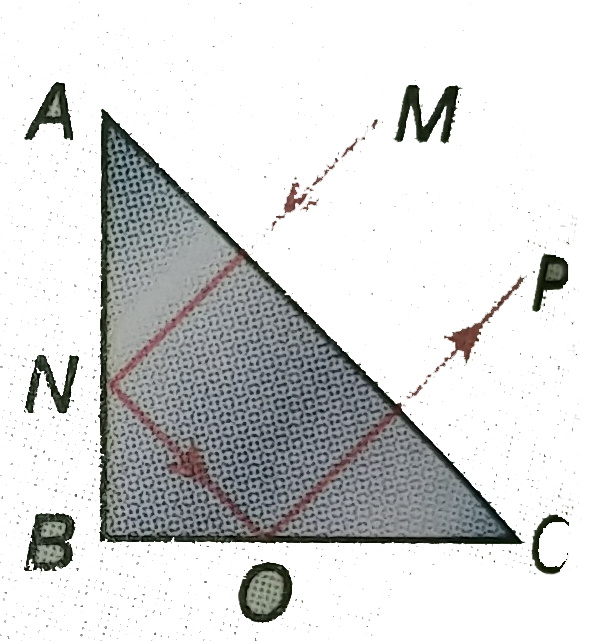
ABC is right angled isosceles triangle, whose ∠ABCis90∘andAB=BC.
Here, light ray is incident along MN on the side AB and is reflected back along NO. Now, the reflected ray NO is incident on side BC and gets reflected along the path OP.
Therefore, light rays suffer total internal reflection when incident on sides AB and BC.
It is clearly seen that the value of incident angle ic on sides AB and BC is 45∘ or less than 45∘.
i.e. ic≤45∘
or, sin−11μ≤45∘or,1μ≤sin45∘or,μ≥√2
Therefore, the refractive index of the material of the prism is at least √2 so that the prism can deviate a ray through 180∘ by total internal reflection.
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFRACTION OF LIGHTEXERCISE - HIGHER ORDER THINKING SKILL (HOTS QUESTION)
25 Videos
- REFRACTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFRACTION OF LIGHTEXERCISE - SECTION RELATED QUESTION
18 Videos
- REFLECTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFLECTION OF LIGHTEXERCISE - INTEGER ANSWER TYPE
3 Videos
- SEMICONDUCTORS AND ELECTRONICS BOOK - CHHAYA PUBLICATIONCHAPTER - SEMICONDUCTORS AND ELECTRONICS EXERCISE - CBSE SCANNER
31 Videos
Similar Questions
What is the minimum value of the refractive index for a 90∘−45∘−45∘ prism which is used to deviate a beam through 90∘ by total internal reflection ?
View SolutionWhat should be the minimum value of refractive index of a prism, refracting angle A, so that there is no emergent ray irrespective of the angle of incidence?

View Solution
Knowledge Check
What is the minimum value of the refractive index for a 90∘−45∘−45∘ prism which is used to deviate a beam through 90∘ by total internal reflection ?
 A3/2B√3C√2D5/3
A3/2B√3C√2D5/3A total reflecting right angled isosceles prism can be used to deviate a ray of light through :
A30∘B60∘C75∘D90∘What should be the minimum value of refractive index of a prism of refractive angle A, so that there is no emergent ray irrespective of angle of incidence?
AsinA2BcosA2CcosecA2DsecA2
What should be the minimum value of refractive index of a prism, refracting angle A, so that there is no emergent ray irrespective of the angle of incidence?

View Solution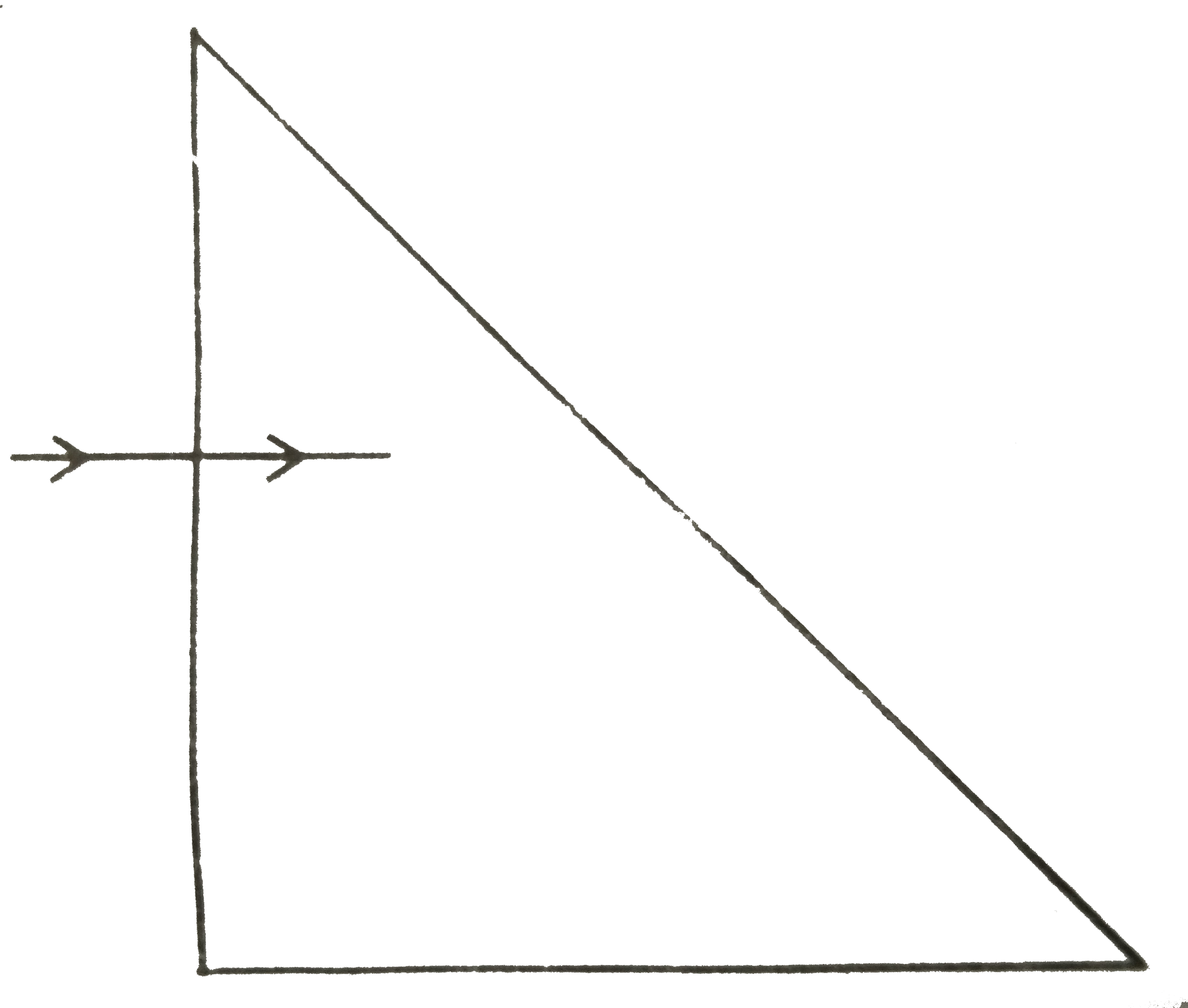
A ray of ligh is incident normally on one of the faces of right
angled isosceles prism as shhown above. It undergoes total
internal reflection from hypotenuse. Which on of the
following is the minimum refractive index of the material of
the prism?
View SolutionThe refractive index of the material of a prism of refracting angle 74∘ is 1.66 for a certain monochromatic ray. What should be the minimumangle of incidence of this ray on the prism so that no total internal reflection takes place as they come out of the prism.
View SolutionThe refractive index of a prism is μ. Find the maximum angle of the prism for which a ray incident on it will be transmitted through other face without total internal reflection.
View SolutionThe refractive index of a prism is μ. Find the maximum angle of the prism for which a ray incident on it will be transmitted through other face without total internal reflection.
View Solution
