A diverging beam of rays from a point source S , making a divergent angle , α are incident on a glass slab (as shown in Fig. 2.82). The angle of incidence of the extreme rays on the two sides is the same. If thickness of the glass slab is t and refractive index n, then the angle of divergence of the emergent rays will be
zero
−α
sin−1(1)n
2sin−1(1)n
The correct Answer is:B
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFRACTION OF LIGHTEXERCISE - EXERCISE Very Short Answer or two Question (Mark1)
55 Videos
- REFRACTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFRACTION OF LIGHTEXERCISE - EXERCISE Short Answer Type Question - 1
16 Videos
- REFRACTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFRACTION OF LIGHTEXERCISE - NCERT EXEMPLAR QUESTIONS WITH ANSWER HINT
2 Videos
- REFLECTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFLECTION OF LIGHTEXERCISE - INTEGER ANSWER TYPE
3 Videos
- SEMICONDUCTORS AND ELECTRONICS BOOK - CHHAYA PUBLICATIONCHAPTER - SEMICONDUCTORS AND ELECTRONICS EXERCISE - CBSE SCANNER
31 Videos
Similar Questions
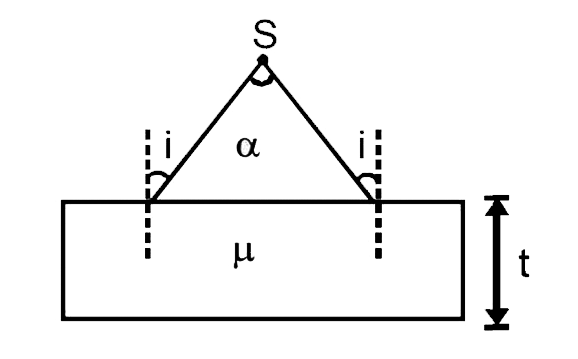
A diverging beam of light from a point source S having devergence angle α, falls symmetrically on a glass slab as shown. The angles of incidence of the two extreme rays are equal. If the thickness of the glass slab is t and the refractive index n, then the divergence angle of the emergent beam is
View SolutionA ray of light is incident perpendicularly on a glass slab. The angle of incidence is 900.
View Solution
Knowledge Check
A diverging beam of light from a point source S having divergence angle α, falls symmetrically on a glass slab as shown. The angles of incidence of the two extrem rays are equal. If the thickness of the glass slab is t and the refractive index n, then the divergence angle of the emergent beam is
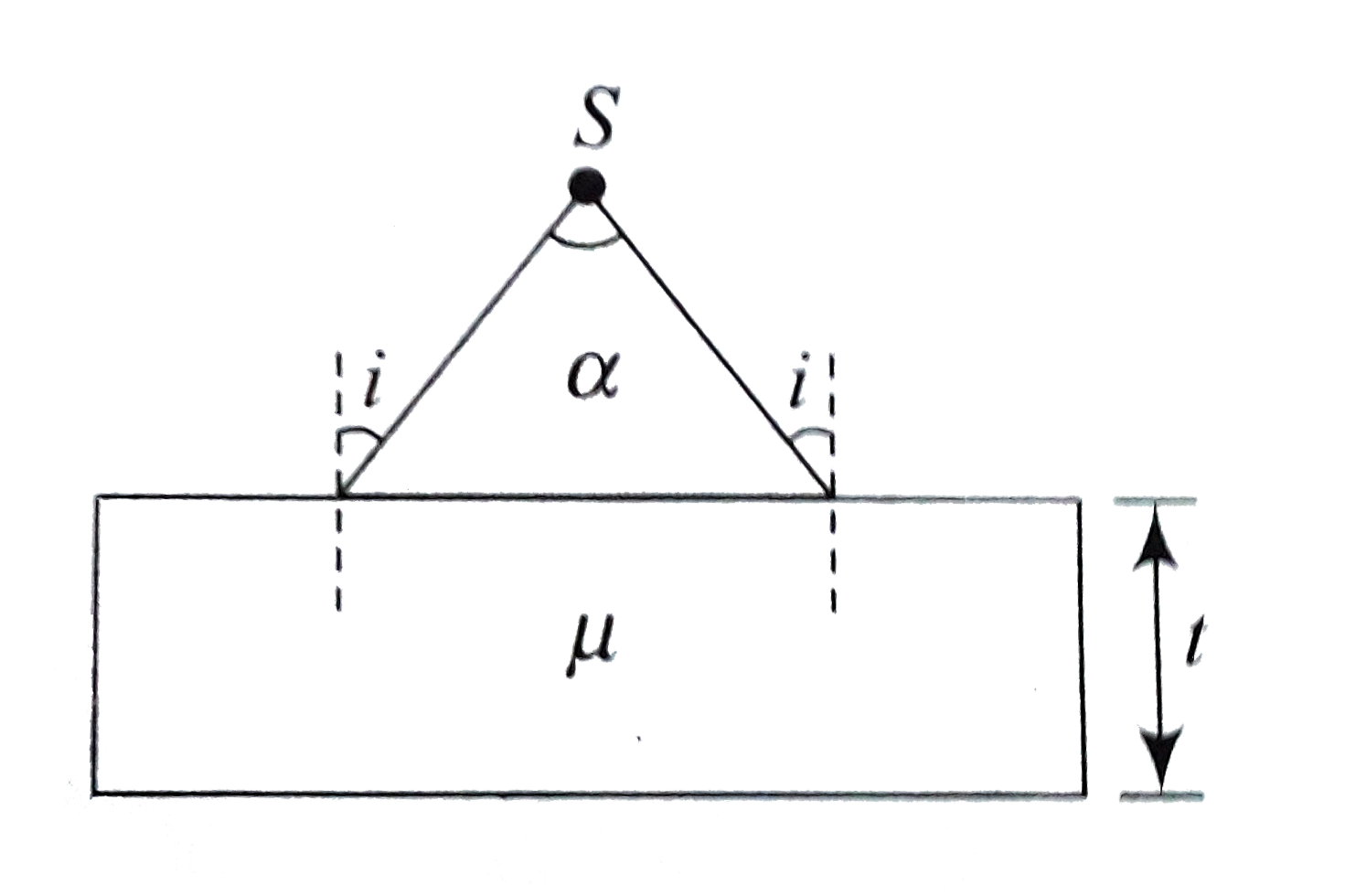 AzeroBαCsin−1(1n)D2sin−1(1n)
AzeroBαCsin−1(1n)D2sin−1(1n)
A diverging beam of light from a point source S having devergence angle α, falls symmetrically on a glass slab as shown. The angles of incidence of the two extreme rays are equal. If the thickness of the glass slab is t and the refractive index n, then the divergence angle of the emergent beam isAzeroBαCsin−1(1n)D2sin−1(1n)A ray of light passes throught a plane glass slab of thickness t and refractive index μ=1.5. The angle between incident ray and emergent ray will be
A0∘B30∘C45∘D60∘
A light ray is incident on a plane glass slab of thickness ‘t’ at an angle of incidence ‘i’ as shown in the figure. If μ is the refractive index of glass. Then find time taken by the light ray to travel through the slab.
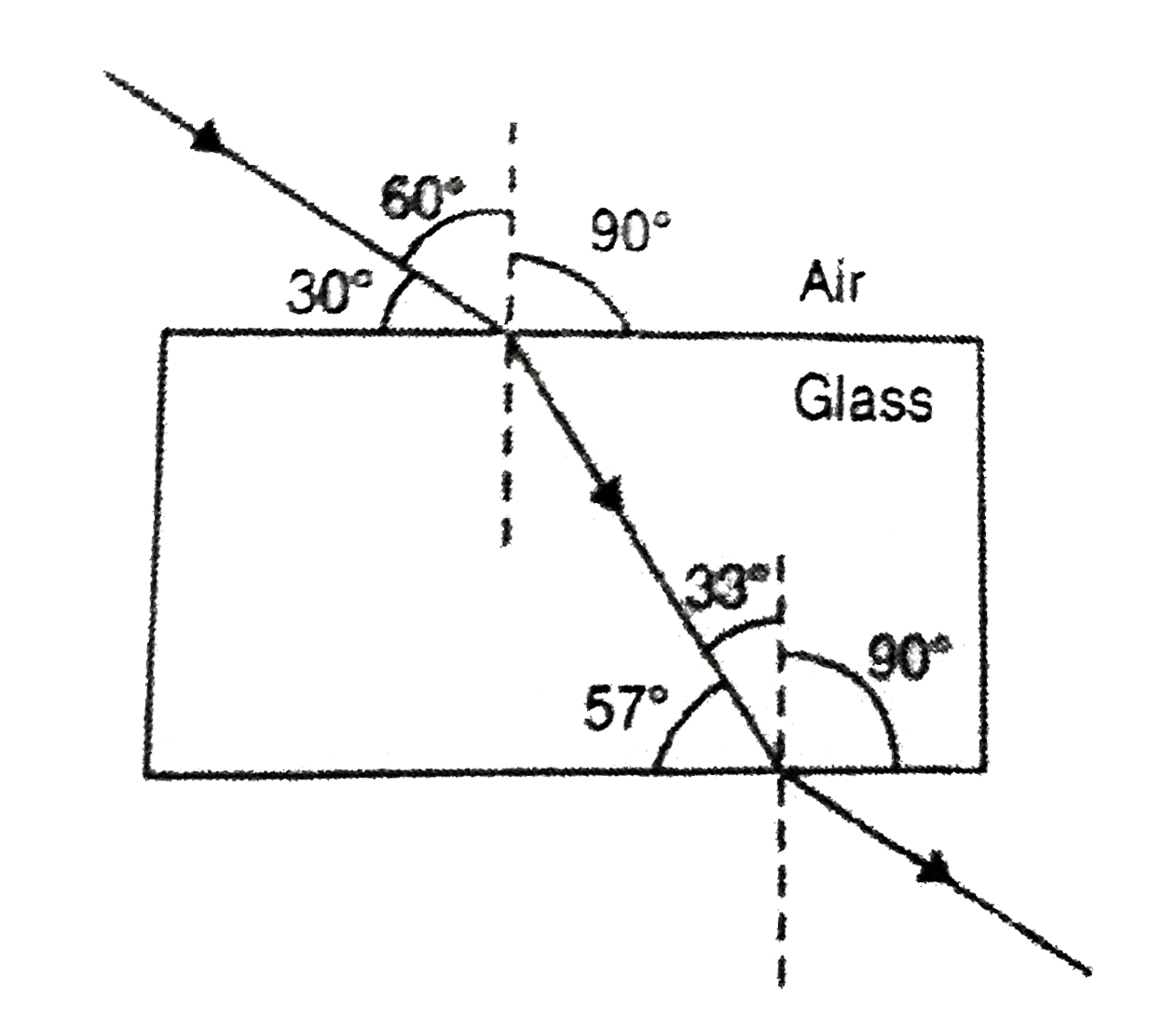
View SolutionA ray of light is incident on a parallel-sided glass slab as shown in the figure. The angle of refraction for this ray of light is likely to be :
View SolutionA ray of light is incident on a glass slab of thickness t, at an angle i,r is the angle of refraction in the glass slab. Distance travelled in the glass slab is
View SolutionThe angle between the incident and the emergent ray from a glass slab is
View SolutionA ray of light is incident on a glass slab at an angle of 45∘. If refractive index of glass be 1.6, what is the angle of refraction ?
View Solution
