Angle between the vectors is . By resolving them into mutually perpendicular components show that the magnitude of the resultant vector is . Solution in Bengali
Step by step video & image solution for Angle between the vectors vecA and vecB is theta. By resolving them into mutually perpendicular components show that the magnitude of the resultant vector is (A^2+B^2+2AB cos theta )^(1//2). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- VECTORBook:CHHAYA PUBLICATIONChapter:VECTORExercise:PROBLEM SET-I
Explore 59 Videos
- VECTORBook:CHHAYA PUBLICATIONChapter:VECTORExercise:PROBLEM SET-II
Explore 34 Videos
- VECTORBook:CHHAYA PUBLICATIONChapter:VECTORExercise:EXERCISE (Short Answer Type Questions-I)
Explore 11 Videos
- THERMOMETRYBook:CHHAYA PUBLICATIONChapter:THERMOMETRYExercise:EXAMINATION ARCHIEVE - WBJEE
Explore 1 Video
- VISCOSITY AND SURFACE TENSIONBook:CHHAYA PUBLICATIONChapter:VISCOSITY AND SURFACE TENSIONExercise:EXERCISE (CBSE SCANNER)
Explore 9 Videos
Similar Questions
Resultant of vectors 3P and 2P is vector R. When the first vector is doubled, R also doubles. Find the angle between the two vectors.
View SolutionResultant of two forces P and Q is √3Q. The resultant is inclined at 30∘ with P . Show that, either P =Q or P=2Q.
View SolutionCan the magnitude of the resultant of two vectors of equal magnitude be equal to the magnitude of each vector ? Explain.
View SolutionA scientist proposes a new temperature scale in which the ice point is 25X (X is the new unit of temperature) and the steam point is 305X. The specific heat capacity of water in this new scale is (in J⋅kg−1⋅X−1)
View SolutionState and prove Bernoulli's theorem.
View SolutionvecA and vecB are two vectors of equal magnitude and theta is the angle between them.The angle between vecA or vecB with their resultant is
View Solutionयदि दो सदिश →A व →B किसी समानान्तर चतुर्भुज की दो आसन्न भुजाओं से निरूपित किये जाते है, तो सिद्ध कीजिए कि इन सदिशों के परिणामी का परिमाण R=√A2+B2+2ABcosθ तथा परिणामी की सदिश →A से दिशा α=tan−1[BsinθA+Bcosθ] होगी | जहाँ A व B क्रमशः सदिशों →A व →B के परिमाण है व उनके बीच का कोण θ है |
View SolutionIf →a,→b are unit vectors and θ is the angle between them, show that
sinθ2=12∣∣→a−→b∣∣
View SolutionIf →a,→b are unit vectors and θ is the angle between them, show that
cosθ2=12∣∣→a+→b∣∣
View SolutionIf →a,→b are unit vectors and θ is the angle between them, show that
tanθ2=∣∣→a−→b∣∣∣∣→a+→b∣∣
View SolutionIf →a and →b are unit vector and θ is the angle between then, then ∣∣∣→a−→b2∣∣∣ is:
View Solutionचित्र में दिखाये गये दो सदिशों →A और →B के बीच का कोण θ है। इनके परिणामी सदिश का परिमाण तथा दिशा उनके परिमाणों तथा θ के पद मे निकालिए।
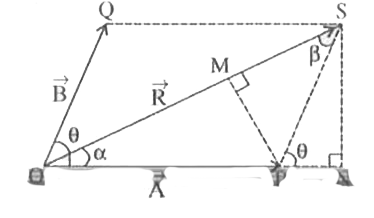
View Solutionयदि दो इकाई सदिशो →a और →b के मध्य का कोण θ तब सिद्ध किजिए कि
sinθ2=12∣∣→a−→b∣∣.
View Solutionयदि →a व →b दो इकाई सदिश हैं तथा इनके बीच का कोण θ है , तब ∣∣∣cosθ2∣∣∣ का मान है
View SolutionThe angle between two vectors →A=→B is θ. The resultant of →Aand→B making an angle θ2 with →A. Then:
View Solution
