A round table is rotated with an angular velocity of 10 rad⋅s−1 about its axis. Two blocks of mass m1=10kg and m2=5 kg , connected to each other by a weightless inextensible string of length 0.3 m, are placed along a diameter of the table. the coefficient of friction between the table and m1 is 0.5, while there is no frication between m2 and the table. Mass m1 is at a distance of 0.124 m from the centre of the table. The masses are at rest with respect to the table. (i) Calculate the frictional force on m1. (ii) What should be the minimum angular speed of the table so that masses will slip from the table? (iii) how should these masses be kept so that the string remains taut but no frictional force acts on m1 ?
The top view of the table is shown in
according to the problem,
AB = 0.3 m:
OA = 0.124 m
So, OB = 0.3 - 0.124
= 0.176 m
Centrifugal force acting on mass m1 along the direction OA,
m1ω2r=10×(10)2×0.124=124 N
Again, centrifugal force acting on mass m2 along the direction OB,
m2ω2r=5×(10)2×0.176=88 N
So, the resultant force along the direction OA
= 124 - 88 = 36 N
In spite of this resultant force, the two masses remain stationary with respect to the table. Hence, the frictional with respect to the table. Hence, the frictional force is equal and opposite to this force.
(ii) Reaction force of the table on mass m1
= weight of the mass = m1g=10×9.8=98N
As the coefficient of friction is 0.5 , the limiting frictional force = 0.5×98 49 N.
If the minimum angular velocity of the table is ω, the resultant of the two opposite centrifugal forces acting on the two masses
= m1ω2r1−m2ω2r2=ω2(10×0.124−5×0.176)
=ω2(1.124−0.88)=0.36ω2 N
According to the problem, resultant of the two centrifugal forces = limiting frictional force
∴ 0.36ω2=49 or,ω2=490036
or, ω=706=11.67rad⋅s−1
If there is no frictional force acting on the masses, the resultant of the two centrifugal forces should be zero. In that case, if mass m1 is placed at a distance r from the centre, then the distance of m2 becomes (0.3 - r).
So, for any value of ω.
m1ω2r=m2ω2(0.3−r) or,0.3−r=m1m2r
or, 0.3 - r = 2r or, 3r = 0.3 or, r = 0.1 m

Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - CHHAYA PUBLICATIONCHAPTER - CIRCULAR MOTIONEXERCISE - SECTION RELATED QUESTIONS
15 Videos
- CIRCULAR MOTIONBOOK - CHHAYA PUBLICATIONCHAPTER - CIRCULAR MOTIONEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
18 Videos
- CALORIMETRYBOOK - CHHAYA PUBLICATIONCHAPTER - CALORIMETRYEXERCISE - EXAMINATION ARCHIVE
5 Videos
- DOPPLER EFFECT IN SOUND BOOK - CHHAYA PUBLICATIONCHAPTER - DOPPLER EFFECT IN SOUND EXERCISE - CBSE SCANNER
2 Videos
Similar Questions
Like linear velocity, is angular velocity a vector quantity? If so, then how can we determine its directon?
View SolutionWhy is a centripetal force necessary for a uniform circular motion ?
View SolutionA 10 Watt electric heater is used to heat a container filled with 0.5 kg of water. It is found that the temperature of water and the container rises by 3, K in 15 minutes. The container is then emptied, dried and filled with 2 kg of oil. The same heater now raises the temperature of container-oil system by 2''K in 20 minutes. Assuming that there is no heat loss in the process and the specific heat of water as 4200 J . kg−1.K−1, the specific heat of oil in the same unit is equal to
View SolutionA train standing at the outer singal of a railway station blows a whistle of frequency 400 Hz in still air. (i) What is frequency of the whistle for a platform observer when the train (a) approaches the platform with a speed of 10 m/s, (b) recedes from the platform with a speed of 10 m/s. (ii) What is the speed of sound in each case if the speed of sound in still air is 340 m/s.
View SolutionThe block each of mass 1kg are placed as shown .They are connected by a string which passes over a smooth (massless) pulley.
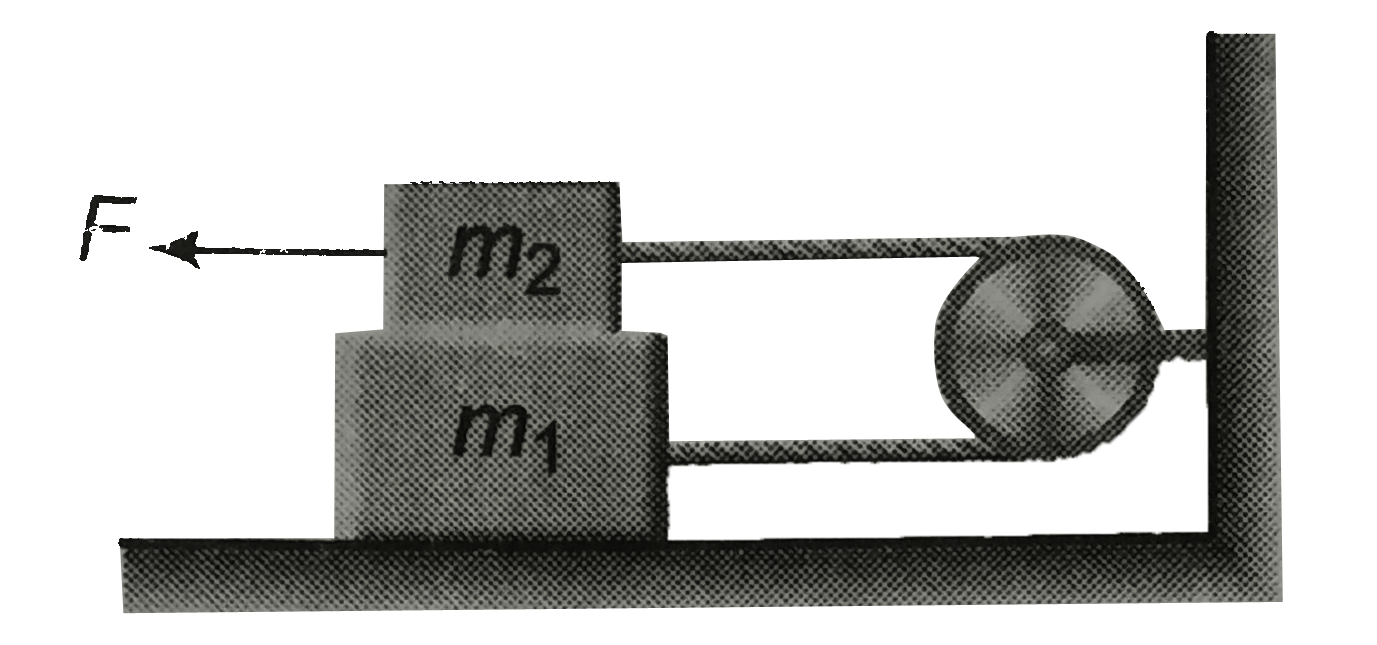
There is no friction between m1 and the ground.The coefficient of friction between m1 andm2 is 0.2A force F is applied to m2. Which of the fpllowing statement is/are correct?
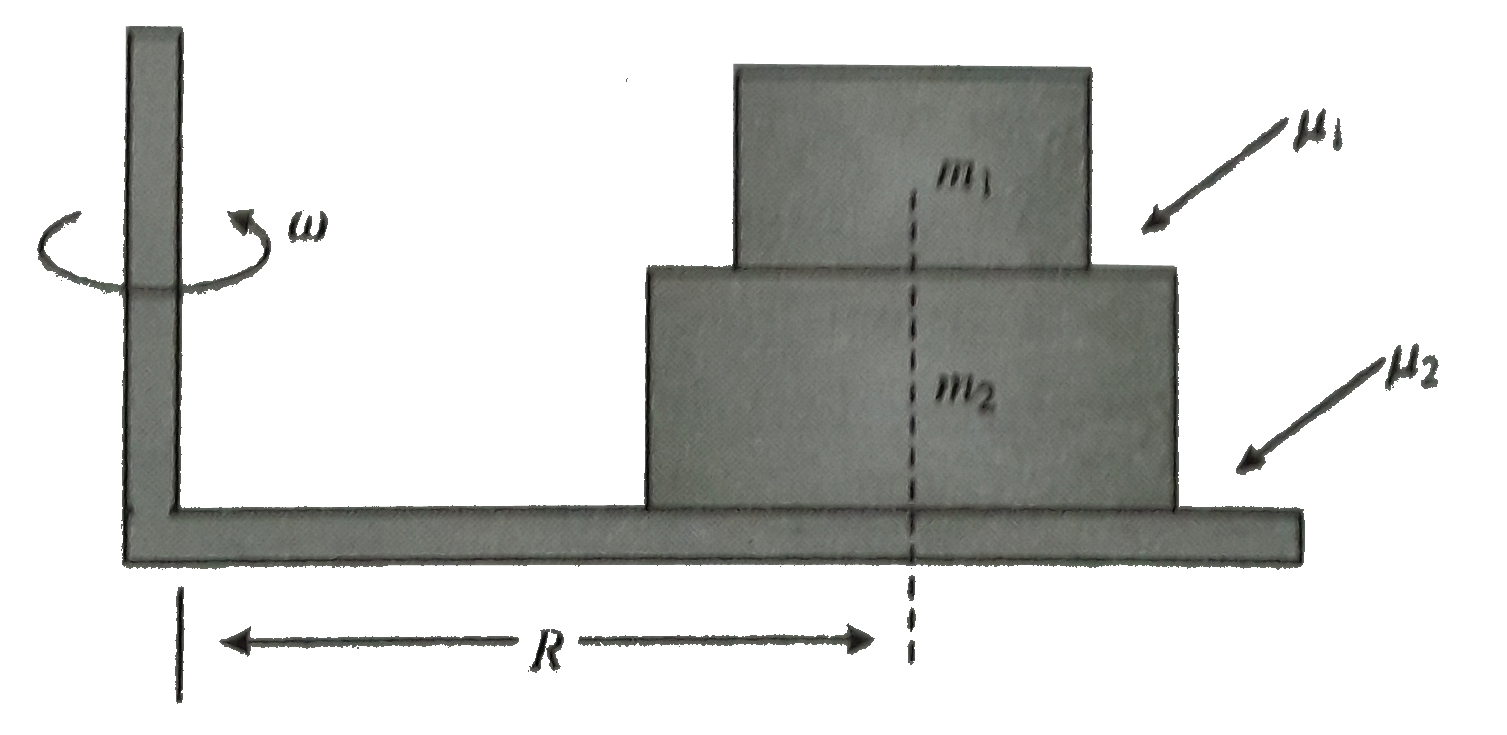
View SolutionThere are two blocks of masses m1 and m2,m1 is placed on m2 on a table which is rotating with an angular velocity ω about the vertical axis . The coefficent of friction between the block is μ1 and between m2 and table is μ2(μ1<μ2) if block are placed at distance R from the axis of ratation , for relative sliding between the surface in contact , find the
a. friction force at the contacting surface
b. maximum angular speed ω
View SolutionTwo blocks of mass m_(1)=10kg and m_(2)=5kg connected to each other by a massless inextensible string of length 0.3m are placed along a diameter of the turntable. The coefficient of friction between the table and m_(1) is 0.5 while there is no friction between m_(2) and the table. the table is rotating with an angular velocity of 10rad//s . about a vertical axis passing through its center O . the masses are placed along the diameter of the table on either side of the center O such that the mass m_(1) is at a distance of 0.124m from O . the masses are observed to be at a rest with respect to an observed on the tuntable (g=9.8m//s^(2)) . (a) Calculate the friction on m_(1) (b) What should be the minimum angular speed of the turntable so that the masses will slip from this position? (c ) How should the masses be placed with the string remaining taut so that there is no friction on m_(1) .
View SolutionTwo blocks with masses M1 and M2 of 10kg and 20kg respectively are placed as in fig. μs=0.2 between all surfaces, then tension in string and acceleration of M2 block will be:

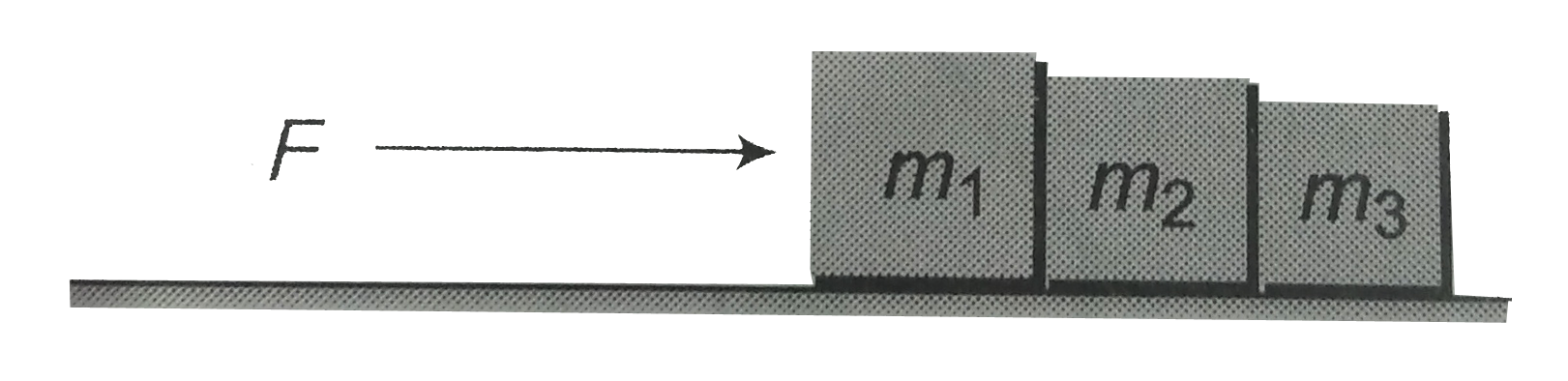
View SolutionThree block of masses m1,m2 and m3 kg are placed in contact with each other on a frictionless table. A force F is applied on the heaviest mass m1, the acceleration of m2 will be
View Solutionm1=10kg व m2=5kg के दो ब्लॉक 0.3m की द्र्व्यमाणहीन अविस्तर्य डोरी द्वारा एक दूसरे से संयोजित है, एक घूमने योग्य टेबल के व्यास के अनुदिश स्थित है । टेबल व m1 के मध्य घर्षण गुणांक 0.5 है जबकि m2 व टेबल के मध्य कोई घर्षण नहीं है । टेबल इसके केंद्र से गुजर रही ऊर्ध्वाधर अक्ष के पारित 10rad/sec कोणीय वेग से घूर्णन कर रही है । दोनों कण द्रव्यमान केंद्र O के एक और टेबल के व्यास के अनुदिश इस प्रकार रखे हुए है की m1,O से 0.124m की दुरी पर है । दोनों द्रव्यमान घूर्णन टेबल पर प्रेक्षक के सापेक्ष विरामावस्था में प्रेक्षित होते है।
(i) m1 पर घर्षण बल की गणना कीजिये।
(ii) घूर्णन टेबल की न्यूनतम कोणीय चाल क्या होनी चाहिए ताकि द्रव्यमान इस स्थिति से फिसले।
(iii) डोरी को तनी हुई रहने के लिए द्रव्यमान को कहा स्थित होना चाइये ताकि m1 द्रव्यमान पर कोई घर्षण बल कार्यरत नहीं हो।
View SolutionA block of mass m_(1)=2 kg is kept on a smooth horizontal table. Another block of mass m_(2)=1 kg is connected to m_(1) by a light inextensible string passing over a smooth pulley as shown in the figure. If the blocks are released, what will be the (a) acceleration of each block (b) tension in the string?
View Solutionm1=10kg व m2=5kg के दो ब्लॉक 0.3m की द्र्व्यमाणहीन अविस्तर्य डोरी द्वारा एक दूसरे से संयोजित है, एक घूमने योग्य टेबल के व्यास के अनुदिश स्थित है । टेबल व m1 के मध्य घर्षण गुणांक 0.5 है जबकि m2 व टेबल के मध्य कोई घर्षण नहीं है । टेबल इसके केंद्र से गुजर रही ऊर्ध्वाधर अक्ष के पारित 10rad/sec कोणीय वेग से घूर्णन कर रही है । दोनों कण द्रव्यमान केंद्र O के एक और टेबल के व्यास के अनुदिश इस प्रकार रखे हुए है की m1,O से 0.124m की दुरी पर है । दोनों द्रव्यमान घूर्णन टेबल पर प्रेक्षक के सापेक्ष विरामावस्था में प्रेक्षित होते है।
(i) m1 पर घर्षण बल की गणना कीजिये।
(ii) घूर्णन टेबल की न्यूनतम कोणीय चाल क्या होनी चाहिए ताकि द्रव्यमान इस स्थिति से फिसले।
(iii) डोरी को तनी हुई रहने के लिए द्रव्यमान को कहा स्थित होना चाइये ताकि m1 द्रव्यमान पर कोई घर्षण बल कार्यरत नहीं हो।
View SolutionThere blocks of masses m1,m2 and m3 are connected by massless strings as shown in figure on a frictionless table. They are pulled with a force T3=50 N. If m1=10 kg, m2=6 kg and m3=2 kg, the tension T2 will be

View SolutionThe block each of mass 1kg are placed as shown .They are connected by a string which passes over a smooth (massless) pulley.

There is no friction between m1 and the ground.The coefficient of friction between m1 andm2 is 0.2A force F is applied to m2. Which of the fpllowing statement is/are correct?
View SolutionMass of three blocks according to the image shown m_(1), m_(2) And m_(3) Friction-free rope is laid on a table. For them T_(3) = 40 N Is drawn by force. If m_(1) = 10 kg, m_(2) = 6kg And m_(3) = 4 kg Stress then T_(2) Will happen -
View Solution
