A small cube (mass = m ) is on the inner curved surface of a funnel rotating about its own axis. Its wall inclines at an angle $θ$ with the horizontal. The cube is kept at a distance r from the vertical axis of rotation of the funnel. If the coefficient of friction between the funnel and the cube is $μ$ , for what frequency of rotation of the funnel, will the cube remain at rest on the surface of the funnel? It is implied that the cube will roll down if the funnel remains stationary.

Text Solution

Verified by Experts

The correct Answer is: $\frac{1}{2 π} \sqrt{\frac{g (sin θ \pm μ c o n θ)}{r (cos θ \pm μ sin θ)}}$

|

Updated on:21/07/2023

Class 11 PHYSICS CIRCULAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A hollow cylinder of radius $R$ rotates about its axis which is vertical. A block remains in contact with the inner wall if the frequency of rotation is $f$ hertz, but falls at lower frequaencies. The coefficient of friction between the block and the cylinder is
A $\frac{g}{2 π^{2} f^{2} R}$
B $\frac{g}{4 π^{2} f^{2} R}$
C $\frac{g}{π^{2} R}$
D $\frac{2 g}{π^{2} f^{2} R}$
Question 2 - Select One
A very small cube of mass 2kg is placed on the surface of a funnel as shown in figure. The funnel is rotating about its vertical axis of symmetry with angular velocity $ω$ . The wall of funnel makes an angle $37^{\circ}$ with horizontal. The distance of cube from the axis of rotation is 20 cm and friction coefficient is $μ$ (Take $g = 10 m / s^{2}$ )

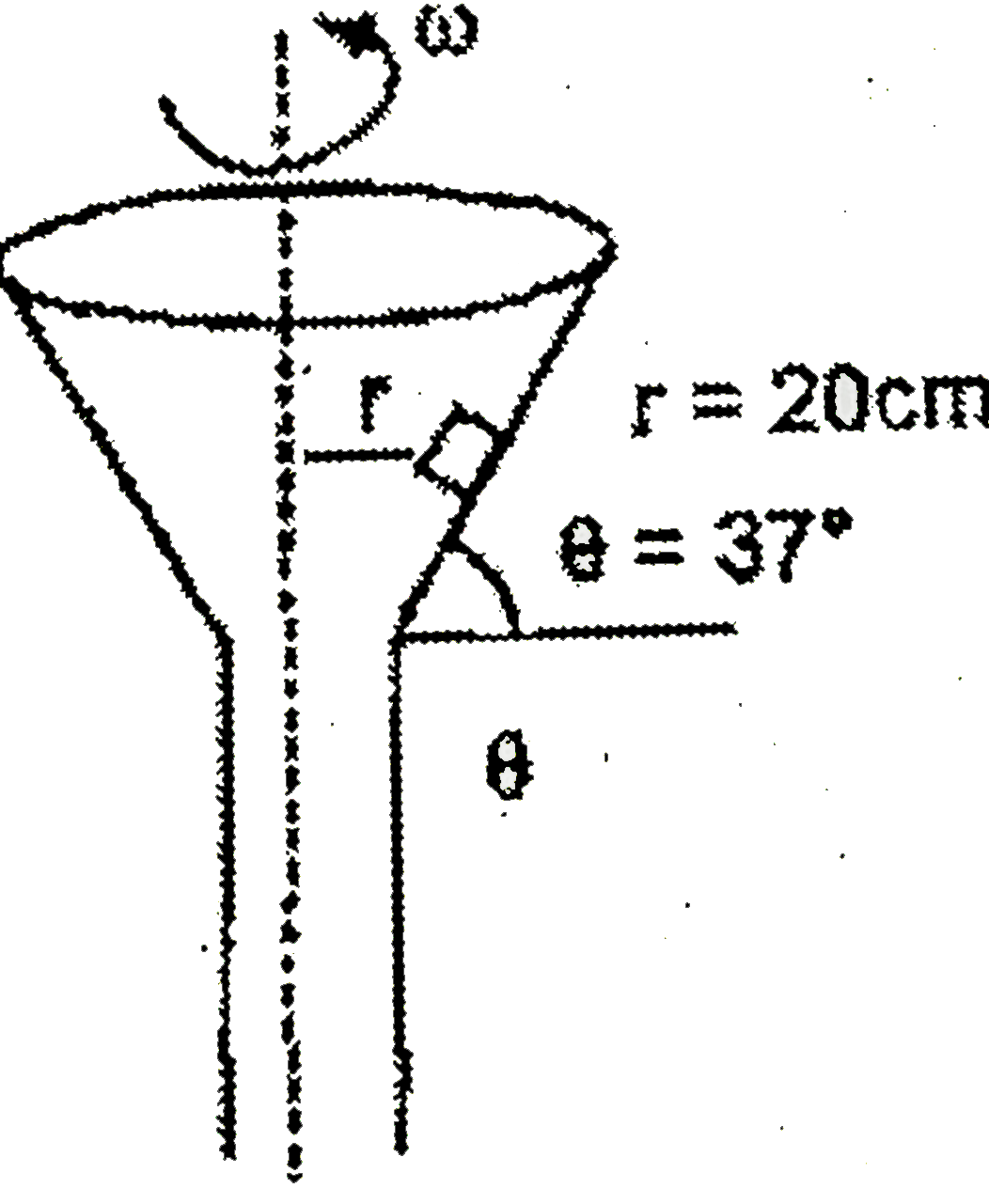
For what value of $ω$ , there would be no frictional force acting between the surfaces :
A5 rad/sec
B $\sqrt{\frac{75}{2}}$ rad/sec
C $\sqrt{6}$ rad/sec
D $\sqrt{40}$ rad/sec
Question 3 - Select One
In thistle funnel experiment, during osmosis the level of the solution in the funnel
AIncreases
BDecreases
CRemains same
DFirst increases then decreases

CHHAYA PUBLICATION-CIRCULAR MOTION-HOTS NUMERICAL PROBLEMS

Home
Ask Doubt
Profile