A tank, closed tightly with a lid, is completely filled with water and is rigidly fixed on a car. The car moves with a constant acceleration of 20cm⋅s−2. Determine the pressure at a point 10 cm below the lid and at a distance of 10 cm from the front wall of the tank.
Let us consider a vertical disc of unit area kept at a depth h from the upper surface of the tank. The disc is at a distance x from the front wall of the car and its width is dx. Let the pressures at distances x and (x + dx) be p and (p + dp) respectively.

∴ p+dp−p=ρ⋅dx⋅a
[ρ = density of the liquid, ρdx = mass of the liquid displaced by the disc, and a = acceleration of the car]
or, dp=aρdx
∴ ∫dp=aρ∫dx
or, p=aρx+c [c = integration constant]
Now, if x = 0, then p = hρg.
∴ hρg=c
∴ p=aρx+hρg=ρ(ax+hg)
∴ The pressure at a point 10 cm below the lid and at a distances of 10 cm from the front wall of the tank,
p=1(20×10+10×980)=200+9800
=10000dyn⋅cm−2
Topper's Solved these Questions
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - SECTION RELATED QUESTIONS
12 Videos
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
37 Videos
- FRICTION BOOK - CHHAYA PUBLICATIONCHAPTER - FRICTION EXERCISE - CBSE SCANNER
7 Videos
- KINETIC THEORY OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - KINETIC THEORY OF GASESEXERCISE - CBSE Scanner
9 Videos
Similar Questions
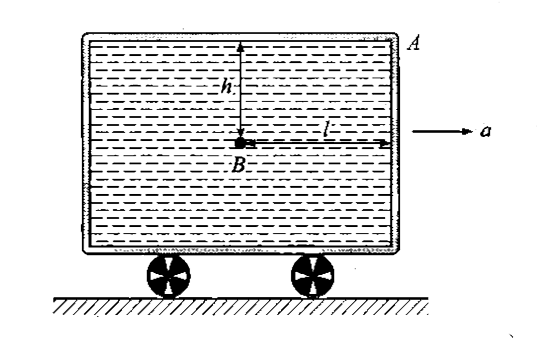
A closed tank filled with water is mounted on a cart. The cart moves with an acceleration 'a' on a plane plane road. What is the difference in pressure between point B and A shown in figure
View SolutionA car moving with constant acceleration crosses two points A and B with velocities 10 m/s and 20 m/s in its path. Find the velocity of the car midway between A and B. (in m/s).
View Solution
Knowledge Check
A cart supports a cubic tank filed with a liquid up to the top. The cart moves with a constant acceleration a in the horizontal direction. The tank is tightly closed. Assume that the liquid when in motion with uniform acceleration. The pressure at a point at a depth h and distance I from the front wall is :
AdghBdlaCdgh+ dlaDdgh - dlaWhat angle θ to the horizon will be formed by the surface of petrol in the tank of a motor car moving horizontally with a constant acceleration of 2.44 m/s2 ?
Aθ=14∘Bθ=45∘Cθ=30∘Dθ=0A particle is moving with a constant linear velocity 5 cm/s, on the circumference of circle of radius 10 cm. The centripetal acceleration of the particle will be
A0B2.5 cm/s2C2 cm/s2D0.5 cm/s2
A point moves along a circle having a radius 20cm with a constant tangential acceleration 5cm/s2 . How much time is needed after motion begins for the normal acceleration of the point to be equal to tangential acceleration?
View SolutionA point moves along a circle having a radius 20cm with a constant tangential acceleration 5cm/s2. How much time (in sec) is needed after motion begins for the normal acceleration of the point to be equal to tangential acceleration?
View SolutionA thief's car moving on a straight road with a speed of 80km/h it is followed by a police car moving at a constant speed of 120km/h, crossing a T-point 20s later than the thief's car. At what distance from the T-point the police car will catch the thief's car?
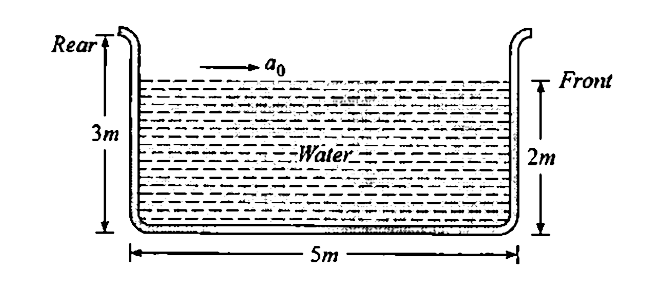
View SolutionAn open reactangular tank with tank with dimensions 5×4m×3m contains water upto a height of 2m is accelerated horizontally along the longer life.
(a) Determine the maximum acceleration that can be given without spilling the water.
(b) If this acceleration is inscreased by 20%. Calculate the percentage of water spilt over.
(c) If initially,the tank is closed at the top and is accelerated horizontally by 9m/s2,find the gauge pressure at the bottom of the front and rear walls of the tank. (g=10m/s2)
View SolutionMercury is completely filled in a rectangular take of height 72 cm. The atmospheric pressure at the place is 72 cm. of Hg. Find the distance in cm of point of application from bottom of tank of the net force , on the inner surface of the side vertical wall of tank.
View Solution
