A flat-bottomed glass tube has a diameter of 4 cm and weighs 30g×g. The centre of gravity of the empty tube is 10 cm above the bottom. Find the amount of water which must be poured into the tube so that when it is floating vertically in a tank of water, the centre of gravity of the system is at the mid-point of the immersed length of the tube.
The correct Answer is:0.1107 kg (approx.)
Topper's Solved these Questions
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - ENTRANCE CORNER (ASSERTION-REASON TYPE)
7 Videos
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - MULTIPLE CORRECT ANSWERS TYPE
4 Videos
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - PROBLEM SET - II
32 Videos
- FRICTION BOOK - CHHAYA PUBLICATIONCHAPTER - FRICTION EXERCISE - CBSE SCANNER
7 Videos
- KINETIC THEORY OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - KINETIC THEORY OF GASESEXERCISE - CBSE Scanner
9 Videos
Similar Questions
A flat bottomed thin-walled glass tube has a diameter of 4 cm and it weights 30 g. the centre of gravity of the empty tube is 10 cm above the bottom, find the amount of water which must be poured into the tube so that when it is floating vertically in a tank of water, the centre of gravity of the tube and its contents is at the midpoint ot the immersed length of the tube.
View SolutionAn empty glass test tube floats vertically in water to a depth of 5 cm. Now, on introducing a 8 cm liquid column into the tube, its depth in water is further increased by 4 cm. Now if the empty test tube is allowed to float vertically in the liquid, 5 cm of the tube is seen in air. Find the total length of the tube.
View Solution
Knowledge Check
A cylindrical tank has a hole of 1 cm in its bottom. If the water is allowed to flow into the tank from a tube above it at the rate of 70cm/sec . then the maximum height up to which water can rise in the tank is
A2.5cmB5cmC10cmD0.25cmA rectangular tank 25 cm long and 20 cm wide contains water to a depth of 5 cm . A metal cube of side 10 cm is placed in the tank so that one face of the cube rests on the bottom of the tank . Find how many litres of water must be poured into the tank so as to just cover the cube ?
A1 LB1.5 LC2LD2.5 LA rectangular tank 25 cm long and 20 cm wide contains water to a depth of 5 cm . A metal cube of side 10 cm is placed in the tank so that one face of the cube rests on the bottom of the tank . Find how many litres of water must be poured into the tank so as to just cover the cube ?
A1 LB1.5 LC2 LD2.5 L
A device used to measure the specific gravity of a liquid is called a hydrogmeter. In a simple hydrometer there is a cylindrical galss tube with some lead-weight at its bottom. The device floats in liquid while remaining vertical. The top part of the tube extends above the liquid and the divisions marked on the tube allows one to directly read the specific gravity of the liquid.
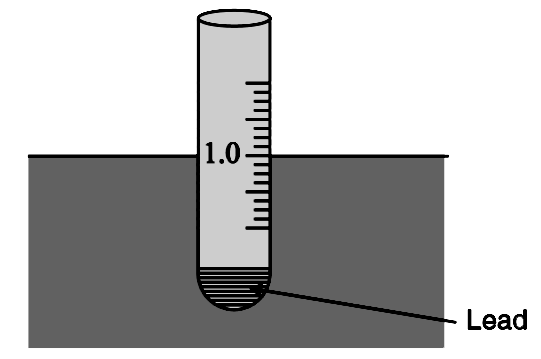
The scale on the tube is calibrated such that in pure water it reads 1.0 at the water surface and a lenght z0 of the tube is submerged. calculate the specific gravity of the liquid if the liquid level is ΔZ above the 1.0 mark. Disregard the curvature of the tube bottom.
View SolutionA test tube consists of a hemisphere and a cylinder of the same radius. The volume of the water required to fill the whole tube is 51596cm3,and42356cm3 of water is required to fill the tube to a level which is 4 cm below the top of the tube. Find the radius of the tube and the length of its cylindrical part.
View SolutionA test tube of thin walls has some lead shots in it at its bottom and the system floats vertically in water,sinking by a length lo=10cm. A liquid of density less than that of water, is poured into the tube till the levels inside and outside the tube are even. If the tube now sinks to a length lo=40cm, the specific gravity of the liquid is___.
View SolutionWater rises upto 10 cm height in a long capillary tube. If this tube is immersed in water so that the height above the water surface is only 8 cm, then
View SolutionWater rises upto 10 cm height in a long capillary tube. If this tube is immersed in water so that the height above the water surface is only 8 cm, then
View Solution
