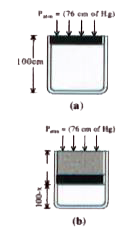
A 100 cm long vertical cylinder,closed at the bottom end , has a movable,frictionless,air tight disattached at its other end. An ideal gas is confined within the cylinder. Initially when the disc between the confined gas and atmosphere is in equilibrium,the length of the gas column is 90 cm. Mercury is poured slowly on the disc. When the disc descends by 32 cm,mercury over it is just about to overflow. Find the atmospheric pressure if the operation took place at a constant temperature of the gas. Neglect the weight or thickness of the disc.
Let the atmospheric pressure be p cmHg and a=area of cross section of the cylinder. Initially in equilibrium, pressure of confined gas = atmospheric pressure p.
Now, volume of enclosed gas V=90acm3
When mercury just overflows from the cylinder,pressure of the gas p1=(p+42) cmHg (Fig.6.4) and volume V1=(90−32)a=58acm3.
As the temperature is constant,according to Boyle.s law
pV=p1V1
or, p×90a=(p+42)×58a
90p=58p+58×42
or,32p=58×42
or, p=58×4232=76.125cmHg

Topper's Solved these Questions
- EXPANSION OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - EXPANSION OF GASESEXERCISE - HIGHER ORDER THINKING SKILLS (HOTS ) QUESTIONS
25 Videos
- EXPANSION OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - EXPANSION OF GASESEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTION)
20 Videos
- ELASTICITY BOOK - CHHAYA PUBLICATIONCHAPTER - ELASTICITY EXERCISE - CBSE SCANNER
10 Videos
- EXPANSION OF SOLIDS AND LIQUIDSBOOK - CHHAYA PUBLICATIONCHAPTER - EXPANSION OF SOLIDS AND LIQUIDSEXERCISE - CBSE SCANNER
2 Videos
Similar Questions
In case of volume expansion of a gas,mention of both the pressure and the temperature is necessary,whereas for expansion of solids and liquids, only the temperature is mentioned.Why?
View SolutionBoth the volume and the pressure of a definite mass of gas are observed to increase. This is possible when the temperature of the gas
View SolutionWhich substances are called elastomers? Given one example.
View SolutionShow that the coefficient of superficial expansion of a rectangular sheet of the solid is twice its coefficient of linear expansion.
View SolutionAn ideal gas is trapped between a mercury column and the closed lower end of a narrow vertical tube of uniform bore. The upper end of the tube is open to atmosphere (atmospheric pressure = 76 cm of Hg). The length of mercury and the trapped gas columns are 20 cm and 43 cm respectively. What will be the length of the gas column when the tube is titled slowly at constant temperature in a vertical plane through an angle of 60^(@) ?
View SolutionA vertical cylinder of total length 100 cm is closed at the lower end and is fitted with a movable frictionless gas tight disc at the other end. An ideal gas is trapped under the disc. Initially the height of the gas column is 90 cm when the disc is in equilibrium between the gas and the atmosphere. Mercury is than slowly poured on the top of the disc and it just starts overflowing when the disc has descended through 32 cm. Find the atmospheric pressure (in cm of Hg). Assume that the temperature of the gas to remain constant and neglect the thickness and weight of the disc. Give your answer excluding the decimal places.

View SolutionA vertical cylinder of total lwgth 100 cm is closed at the lower end and fitted with a movable , frictionless, gas - tight disc ,at the other end. An ideal gas is trapped under the disc of negligible mass. Initially the height of the gas column is 90 cm and the dis is in equlibrium . Mercury is slowly poured on the top of the disc and it just starts overflowing when the disc has descended thorugh 32 cm. find the atmospheric pressure.
View SolutionOne of the limbs of a mercury manometer is connected to a gas cylinder. The mercury level in the limb connected to the gas cylinder is 10 cm higher than that in the other limb. Then the gauge pressure of the gas is ____ cm of Hg.
View SolutionA uniform tube closed at one end, contains a pallet of mercury 4 cm long. When the tube is kept vertically with closed end upwards, the length of air column between closed end and mercury pallet is 10 cm. the tube is inverted so that open end becomes upwards. find the length of trapped air column (in cm) trapped. take atmospheric pressure 76 cm of Hg.
View SolutionA vertical cylinder of height 100 cm contains air at a constant temperature and its top is closed by a frictionless piston at atmospheric pressure (76 cm of Hg) asshown in figure-2.12. If mercury is slowly poured on the piston, due to its weight airis compressed. Find the maximum height of the mercury column which can be put on the piston.
View SolutionAn ideal gas is trapped between a mercury column and the closed lower end of a narrow vertical tube of uniform bore. The upper end of the tube is open to the atmosphere. (Atmospheric pressure is 76 cm of mercury). The length of the mercury and the trapped gas columns are 20 cm and 43 cm, respectively. What will be the length of the gas column when the tube is tilted slowly in a vertical plane through an angle of 60∘. Assume the temperature to be constant.
View SolutionAn ideal gas is trapped between a mercury column and the closed end of a narrow vertical tube of uniform base containing the column. The upper end of the tube is open to the atmosphere. The atmospheric pressure equals 76 cm of mercury. The lengths of the mercury column and the trapped air column are 20 cm and 43 cm respectively. What will be the length of the air column when the tube is tilted slowly in a vertical plane through an angle of 60∘? Assume the temperature to remain constant.
View SolutionA vertical cylinder of height 100cm contains air at a constant temperature and its top is closed by a frictionless piston at atmospheric pressure (76 cm of Hg) as shown figure - a. If mercury is slowly poured on the piston, due to its weight air is compressed. Find the maximum height of the mercury column which can be put on the piston.
View SolutionAn ideal gas is trapped between a mercury column and the closed lower end of a narrow vertical tube of uniform bore. The upper end of the tube is open to the atmosphere. (Atmospheric pressure is 76 cm of mercury). The length of the mercury and the trapped gas columns are 20 cm and 43 cm, respectively. What will be the length of the gas column when the tube is tilted slowly in a vertical plane through an angle of 60^(@) . Assume the temperature to be constant.
View SolutionAn ideal gas is trapped between a mercury column and the closed end of a narrow vertical tube of uniform base containing the column. The upper end of the tube is open to the atmosphere: The atmospheric pressure equals 76 cm of mercury. The lengths of the mercury column and the trapped air column are 20 cm and 43 cm respectively. What will be the length of the air column when the tube is tilted slowly in a vertical plane through an angle of 60°? Assume the temperature to remain constant.
View Solution
