A chamber contains a mass of a gas at pressure .A second chamber contains a mass of the same gas at pressure .IF the two chambers are now connected, what will be the pressure of the gas mixture?
Let = chamber of the 1st chamber =volume of the 2nd chamber
M=molecular weight of the gas
p=final pressure of the gas mixture.
T=Constant temperature before and after mixing of the gases.
We use the relation .
For the 1st chamber ,,
and for the 2nd chamber ,
Using the relation for the gas mixture,we have
Now,
So,
Topper's Solved these Questions
- EXPANSION OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - EXPANSION OF GASESEXERCISE - HIGHER ORDER THINKING SKILLS (HOTS ) QUESTIONS
25 Videos
- EXPANSION OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - EXPANSION OF GASESEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTION)
20 Videos
- ELASTICITY BOOK - CHHAYA PUBLICATIONCHAPTER - ELASTICITY EXERCISE - CBSE SCANNER
10 Videos
- EXPANSION OF SOLIDS AND LIQUIDSBOOK - CHHAYA PUBLICATIONCHAPTER - EXPANSION OF SOLIDS AND LIQUIDSEXERCISE - CBSE SCANNER
2 Videos
Similar Questions
Two chambers containing m1 and m2 kg of gas at pressure p1 and p2 are later connected. What will be pressure of the mixture.
View SolutionA container is divided into two chambers by a partition. The volume of first chamber is 4.5 litre and second chamber is 5.5 litre. The first chamber contain 3.0 moles of gas at pressure 2.0 atm and second chamber contain 4.0 moles of gas at pressure 3.0 atm. After the partition is removed and the mixture attains equilibrium, then, the common equilibrium pressure existing in the mixture is x×10−1 atm. Value of x is___.
View Solution
Knowledge Check
An insulated container of gas has two chambers separated by an insulating partition. One of the chmabers has volume V1 and contains ideal gas at pressure P1 and temperature T1.The other chamber has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partition is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
A(T1T2P1V1+P2V2P1V1T2+P2V2T1BP1V1T1+P2V2T2P1V1+P2V2CP1V1T2+P2V2T1P1V1+P2V2DT1T2(P1V1+P2V2)P1V1T1+P2V2T2A gas is filled in a container at pressure P0. If the mass of molecules is halved and their rms speed is doubled, then the resultant pressure would be
A2P0B4P0CP04DP02Gas at a pressure P0 in contained as a vessel. If the masses of all the molecules are halved and their speeds are doubles. The resulting pressure P will be equal to
A4P0B2P0CP0DP02
Along the line - 1, mass of gas m1 and pressure is p1. Along the line - 2 mass of same gas is m2 and pressure is p2. Choose the correct options.
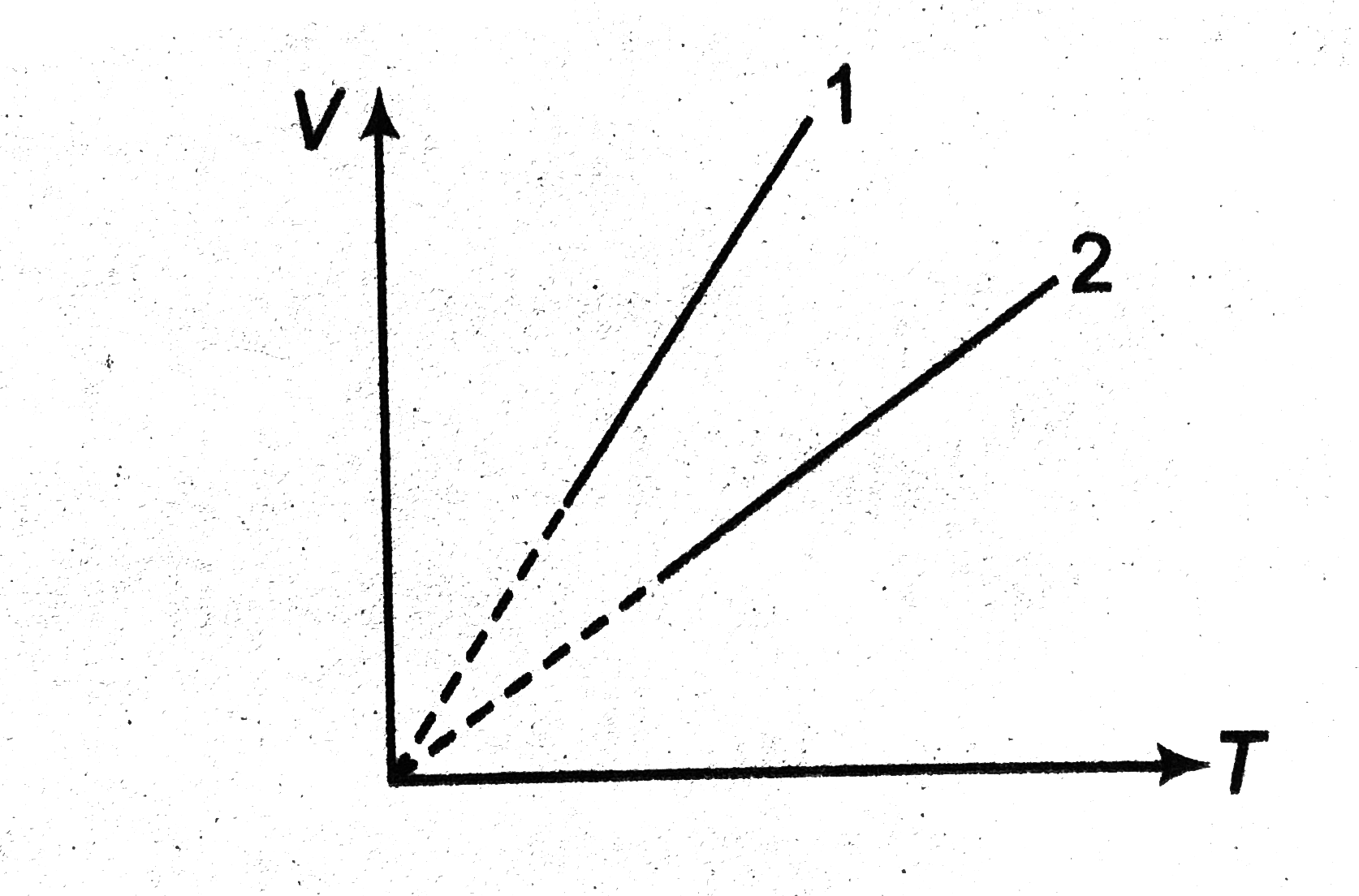 .
.
View SolutionAn insulated container of gas has two chambers separated by an insulating partition. One of the chambers has volume V1 and contains ideal gas at pressure P1 and temperature T1.The other chamber has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partition is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
View SolutionAn insulated container of gas has two chambers separated by an insulating partition. One of the chambers has volume V1 and contains ideal gas at pressure P1 and temperature T1.The other chamber has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partition is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
View SolutionA stainless steel chamber contains Ar gas at a temperature T and pressure P. The total number of Ar atoms in the chamber is n. Now Ar gas in the chamber is rep,aced by CO2 gas and the total number of CO2 molecules in the chamber is n/2 at the same temperature T. The pressure in the chamber now is P'. Which one of the following relations relations holds true ? (Both the gases behave as ideal gases)
View SolutionAt pressure P and absolute temperature T a mass M of an ideal gas fills a closed container of volume V. An additional mass 2M of the same gas is added into the container and the volume is then reduced to V3 and the temperature to T3. The pressure of the gas will now be:
View Solution
