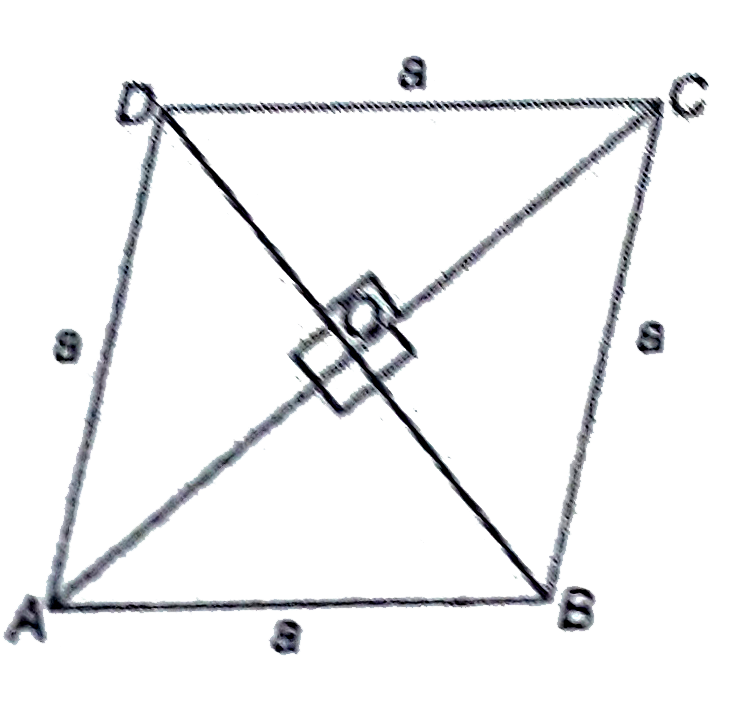
Prove that the sum of the squares of the sides of rhombus is equal to the sum of the squares of its diagonals.

Video Solution

Text Solution

Verified by Experts

Let ABCD be a rhombus whose eachd side is a. the diagonals of rhombus bisects perpendicularly at O.
Now, in right $△ A O B$
$O A^{2} + O B^{2} = a^{2}$ ( by pythagores theorem)
${(\frac{1}{2} A C)}^{2} + {(\frac{1}{2} B D)}^{2} = a^{2}$ ( diagonals bisect each other)
$\frac{1}{4} A C^{2} + \frac{1}{4} B D^{2} = a^{2}$
$\Rightarrow A C^{2} + B D^{2} = 4 a^{2}$
$\Rightarrow A C^{2} + B D^{2} = a^{2} + a^{2} + a^{2} + a^{2}$
$\Rightarrow A C^{2} + B D^{2} = A B^{2} + B C^{2} + C D^{2} + D A^{2}$ Hence proved.

Updated on:21/07/2023

|

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Each side of a rhombus is 10 cm, the sum of square of its diagonal is
AA)20 ${cm}^{2}$
BB)200 ${cm}^{2}$
CC)400 ${cm}^{2}$
DD)100 ${cm}^{2}$

NAGEEN PRAKASHAN-TRIANGLES -Revision Exercise Long Questions

Home
Ask Doubt
Profile